Journal Club for November 2023: Top-down multi scale damage mechanics

 

Chloé Arson, Ph.D.

Professor, Cornell University School of Civil and Environmental Engineering

 

 Introduction

Continuum Damage Mechanics (CDM) initially aimed to predict the reduction of stiffness and strength with the propagation of defects in solids. Kachanov’s pioneering micromechanical contributions to CDM (Kachanov, 1980, 1982a,b, 1992) inspired many researchers to use a bottom-up approach to understand the relationship between statistical microstructure descriptors (such as micro-crack distributions) and mechanical properties (such as stiffness) that can be defined at the scale of a Representative Elementary Volume (REV). In bottom-up approaches, the REV-scale field variables and properties are calculated by statistical averaging (Bazant and Oh, 1985; Bazant and Prat, 1988; Krajcinovic et al., 1991; Gambarotta and Lagomarsino, 1993; Pensee et al., 2002; Pensee and Kondo, 2003; Paliwal and Ramesh, 2008) or homogenization (Krajcinovic and Sumarac, 1989; Ju, 1991; Dormieux et al., 2006; Pouya et al., 2016; Shen et al., 2019; Shen and Arson, 2019; Shen et al., 2021a; Xu et al., 2022; Xu and Arson, 2022, 2023), from microscopic field variables that are calculated for every single type of crack or defect present in the REV. Homogenization schemes are attractive because they are explanatory, but they are notorious for being difficult to calibrate. The self-consistent homogenization method is particularly computationally intensive, because it requires an iterative calculation of the local field variables. The use of the theory of Thermodynamic of Irreversible Processes (TIP) with continuum damage dissipation variables is an alternative to the formulation of micromechanical models of crack propagation. The second order damage tensor is typically defined as follows:

in which ρ(n) is the distribution of crack planes of normal direction n on the unit sphere. It can be shown mathematically (see (Lubarda et al., 1994; Yang et al., 1999) for details) that the crack density function ρ(n) is related to the damage tensor as follows:

The free enthalpy of the REV can then be expressed as a function of the second order damage tensor D, as follows:

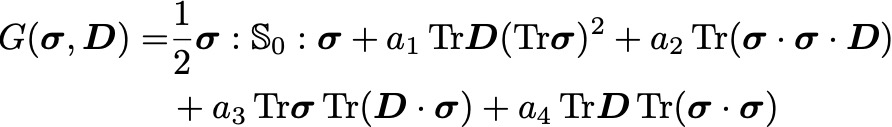
In this post, I will explore the strategies that have been explored to date to enrich the theory of TIP with microstructure variables, mainly, fabric enrichment, non-local formulations, the theory of micromorphic media and the framework of stochastic gradient plasticity. Such top-down approaches are sometimes viewed as too phenomenological and not physical enough. But they present the advantage of depending on fewer constitutive parameters than bottom-up models such as homogenization methods. Moreover, rigorous calibration procedures have been developed for several classes of top-down TIP-based models. More details on this topic are available in the review paper that I published under a Creative Commons License in Open Geomechanics (Arson, 2020), from which I used some of the text presented below.

 

Fabric enrichment

Fabric-enriched models are essentially phenomenological models in which the damage tensor is defined as a convolution of moments of probability of microstructure descriptors. The free energy and the dissipation potential are postulated at REV scale and constitutive equations are obtained from the thermodynamic conjugation relationships. For example, consider a second-order damage tensor D = kF, in which k = Tr (D) and F is a trace-less second-order fabric tensor. The most general form of the REV Helmholtz free energy density ψ that ensures that the undamaged stress/strain relationship is linear is given by:

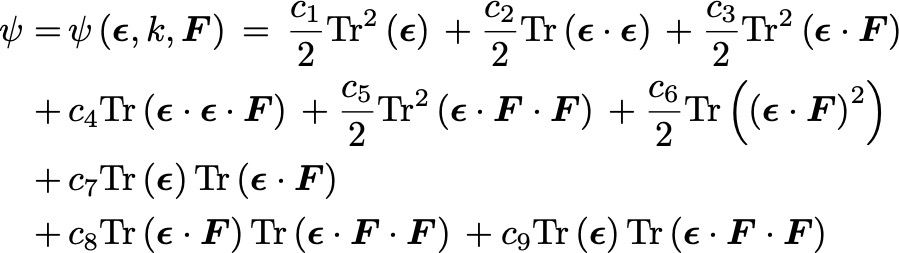
where the coefficients ci are functions of k and of the two first invariants of F, the expressions of which are given in (Zysset and Curnier, 1995). The expression of F can be customized to capture the evolution of measurable microstructure features that are relevant to the phenomena observed at REV scale. In material sciences, poly-dispersed media are often characterized by (Reid, 1955; Philleo, 1983; Torquato et al., 1990; Lu and Torquato, 1992b,a; Torquato and Lu, 1993; Blum and Eisenlohr, 2009):

• the pore size distribution: probability density function (pdf) of a pore size (typically, largest segment that can be fit in a virtual ellipse);
• the lineal-path function L(z): probability of finding a line segment of length z wholly in one of the phases (e.g., grain, pore) of the sample;
• the chord length pdf: pdf of line segments contained fully within a phase (e.g., grain or pore) and extending between two points on the surface of the object in that phase;
• the void nearest-surface distribution function hj(r): probability that at an arbitrary point, the nearest surface of a particle or pore of phase j lies at a distance between r and r + dr;
• the void exclusion probability EV(r): probability of finding a spherical region of radius r that is empty of solid particle center.

In structural geology, fabric is typically described with the distributions of crack length, aperture and orientation (Long et al., 1982; Wilson et al., 2003; Chester et al., 2004). In particulate mechanics, the fabric tensor is usually a measure of the orientation of the vectors that are normal to the plane tangent to two grains in contact (called contact vectors). Figure 1 shows two fabric tensors defined by Li and Li (Li and Li, 2009) to explain the internal variable used in the Anisotropic Critical State Theory (ACST) initially proposed by Dafalias’s group (Dafalias and Manzari, 2004; Dafalias et al., 2004; Li and Dafalias, 2011; Fu and Dafalias, 2011; Gao et al., 2014). In the ACST, any deviatoric second-order tensor can be used as a measure of fabric. Both tensors defined by Li and Li require a Voronoi-Delaunay tessellation of the granular medium. The contact-based fabric tensor depends on the contact vector from void centroid to grain contacts, and characterizes the arrangement of so-called “solid cells”, which each contains a grain and its assigned void space. The assigned void space is calculated by dividing each void element into void tetrahedra whose vertices are the three vertices of a Delaunay boundary surface and the center of the void element, and by joining these tetrahedra to the solid elements that share the same Delaunay boundary surfaces. The void-based fabric tensor depends on the contact vector from grain centroid to grain contacts, and characterizes the arrangement of so-called “void cells”, which each contains a pore (or void) and its assigned solid space. The latter is calculated by dividing each grain into solid tetrahedra whose vertices are the three vertices of a Delaunay boundary surface and the center of the grain, and by joining these tetrahedra to the void elements that share the same Delaunay boundary surfaces.

 

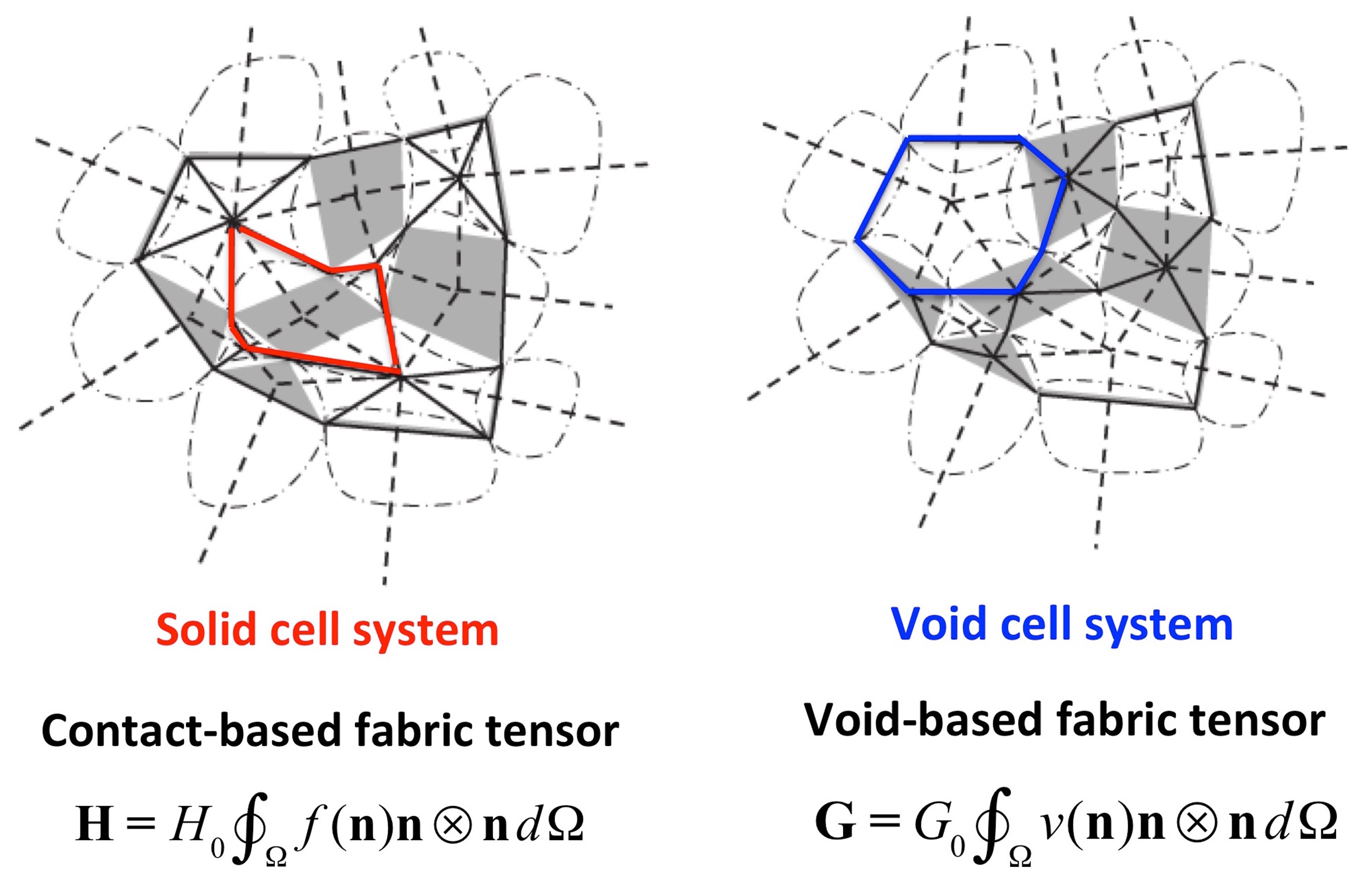
Figure 1. Fabric descriptors defined by Li and Li (2009). f(n): norm of contact vector from void centroid to grain contacts; v(n) norm of contact vector from grain centroid to grain contacts; H0, G0: normalization coefficients. Figure 6 from (Arson, 2020), article licensed under the Creative Commons Attribution Non-Commercial Share Alike 4.0 License.

The contact-based fabric tensor and the void-based fabric tensor can be used to characterize damage in rocks made of polycrystals of cemented aggregates. Cracks affect the geometry of the grains (crystals or aggregates) and of the pores, which makes the contact-based and void-based fabric tensors particularly efficient to track the evolution of intra- and inter-granular crack sizes, shapes, orientations and connectivity. A fabric tensor F can be defined for each descriptor, by integrating the corresponding pdf. In 2D, fabric tensors are 2 x 2 and take the following expression:

where F is a symmetric second-rank tensor; Γ is the whole solid angle, and equals to 2π for 2D images; E(Γ)is a pdf. The components of the fabric tensors are calculated as follows:

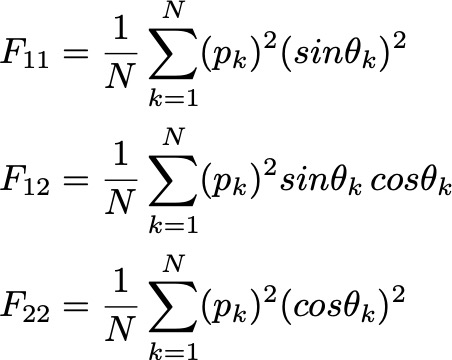
N is the total number of measures (or angles) considered in the image.

For example, my group proposed a fabric tensor based on the statistical analysis of 2D halite micrographs extracted at key stages of oedometer tests and triaxial tests (Shen et al., 2021b). Contact branches, defined as segments linking the centers of two grains in contact, were plotted. A map of polygons was obtained, as illustrated in Figure 2(a). The solid volume fraction of of each polygon was then calculated. At grain scale, the magnitude of the Local Solid Volume Fraction (LSVF) was calculated as the total solid volume fraction of the polygons that intersect with the grain. The LSVF is plotted grain by grain in Figure 2(b). The LSVF orientation of a grain was calculated as an average of the grain branch orientations, in which the weights were proportional to the solid volume fraction of the polygons intersected by the grain. Mathematically, the local solid volume fraction fabric tensor is defined by the three equations above giving the expressions of F11, F22 and F12, in which pk is the local solid volume fraction of each polygon and θk is the angle between the horizontal and the line connecting a polygon’s center with the image’s center.

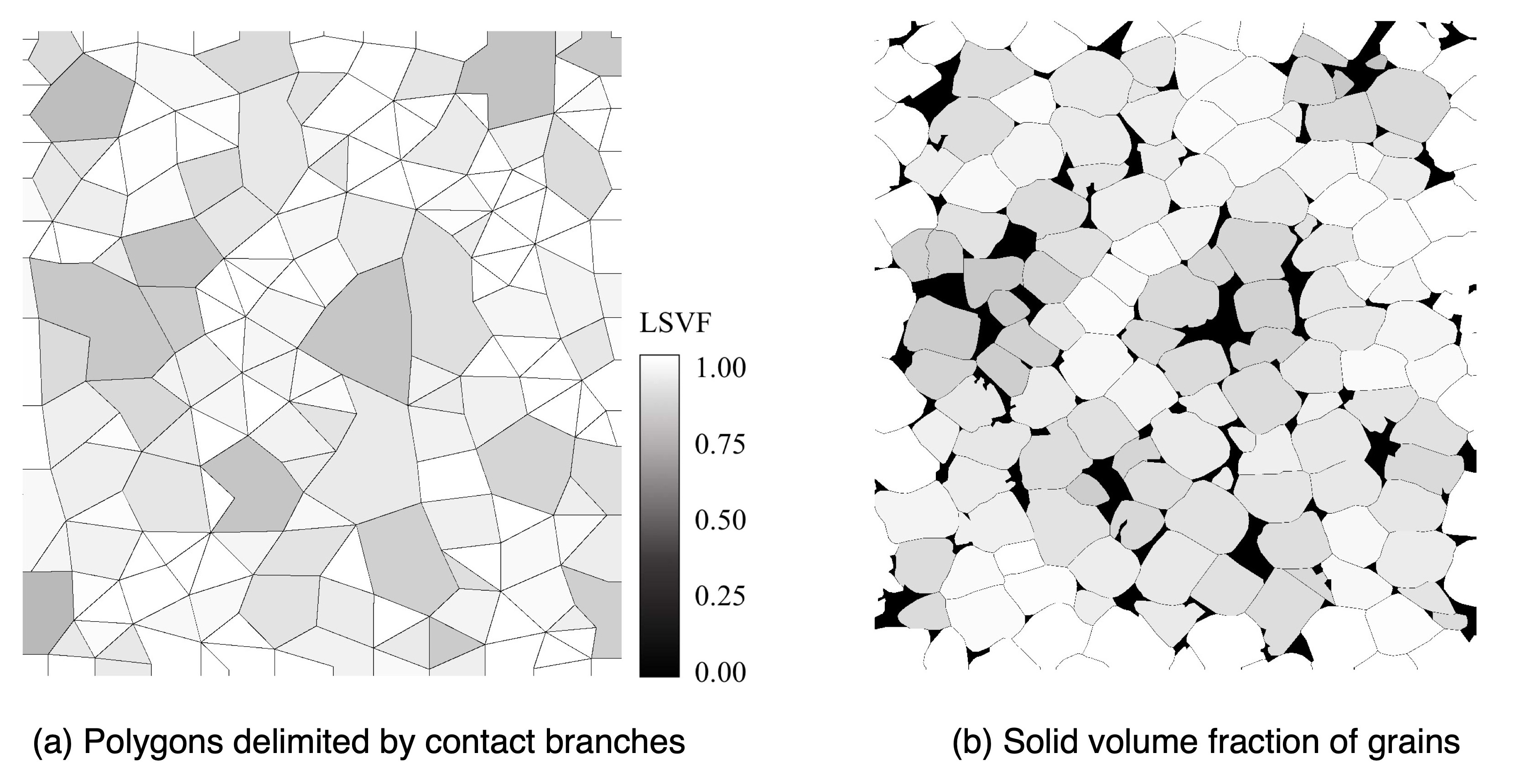
Figure 2. Local solid volume fraction of grains. a. The polygons map is constructed by plotting branches from grain centroid to grain centroid; the local solid volume fraction is then calculated for each polygon. b. The area of the surface covered by the polygons that overlap with a grain is called the domain of this grain; the solid volume fraction of a grain is the averaged solid volume fraction of the domain of that grain. Figure 5 from the article (Shen et al., 2021b) published in Acta Geotechnica, reproduced under Springer License agreement n. 501857376.

With very little error, all the fabric tensors that we calculated from image analysis were found to be diagonal and orthogonal, and for each image i, we defined a normalized fabric tensor Hi as follows:

where Gi and Bi are the grain orientation and branch orientation fabric tensors, Li and Si are the traceless components of the fabric tensors of LSVF and grain solidity, and γi is a normalizing coefficient used to make Tr(Hi) equal to 1. Grain solidity is defined as the ratio between the area of a grain image by the area of the convex hull of the grain. In the expression of the grain solidity fabric tensor, pk is the grain solidity of each grain and θk is the angle between the horizontal and the line connecting a grain’s center with the image’s center. For the tensors of grain orientation and branch orientation, θk is the orientation angle of the vector n in reference to a set axis in the image. Figure 3 shows the evolution of the descriptors used in the definition of the fabric tensor during odometer tests conducted on specimens with different initial porosities.

Figure 3. Probability density functions of four microstructure descriptors obtained in oedometer tests for samples with different porosities. Figure 7 from (Arson, 2020), article licensed under the Creative Commons Attribution Non-Commercial Share Alike 4.0 License.

The second-rank fabric tensor Hi can be written as kiI+Ki. ki is a scalar, and Ki is a traceless second-rank tensor. Calculating Hi for each image i allows tracking the evolution of fabric with deformation. Using the expression of the free energy of an elastic medium enriched with microstructure (expression of Ψ above), we expressed the stiffness tensor of salt rock as a function of k, K and material properties. The coefficients ai can be understood as functions of k and Lame-like constants, μc and λc. Considering that the salt rock Young’s modulus is an exponential function of porosity (Turner and Cowin, 1987), we proposed to relate μc and λc to the average local solid fraction αl and to average grain solidity αs as follows:

We calibrated the parameters m and n against the experimental oedometer modulus (i.e., the ratio of axial stress by axial strain). After calibration, the model was used to calculate the fabric tensor Hi for each image and to deduce the damaged stiffness tensor, according to the expression of Ψ above. Figure 4 shows how the oedometer modulus calculated numerically (red dots) fits the damaged oedometer modulus measured experimentally (solid line).

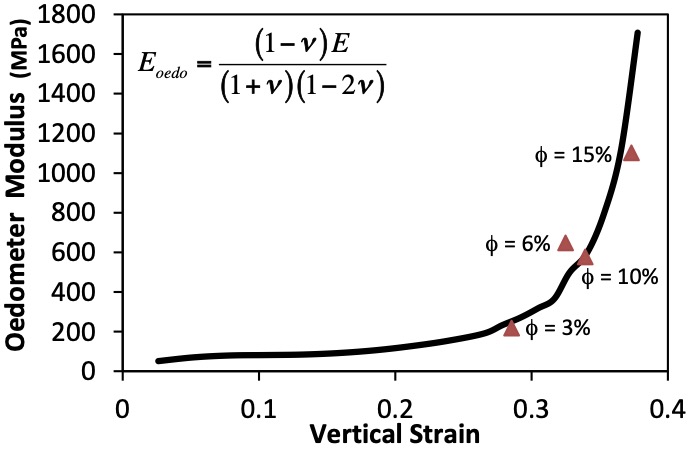
Figure 4. Calibration of the Lamé-like parameters in the fabric-enriched CDM model, against oedometer tests. Figure 8 from (Arson, 2020), article licensed under the Creative Commons Attribution Non- Commercial Share Alike 4.0 License.

It remains challenging to capture the full range of possible rock fabrics and textures encountered in nature (Vernon, 2018). For instance, connections between cracks imply enhanced hydraulic crack interaction, but not necessarily mechanical crack interaction, especially if cracks are randomly oriented and if the distribution of cracks is dense (Schubnel et al., 2006). Two different damage variables are necessary to model permeability enhancement and stiffness degradation (Maleki and Pouya, 2010).

 

Non-local damage formulations

Integral formulations

Non-local formulations consist in replacing one or more local field variables by a non-local counterpart, which translates the influence of a field variable defined at a position x on that field variable in a neighborhood around x. In integral formulations (Bazant and Ozbolt, 1990; Bazant and Jirasek, 2002), space averages are weighted by attenuation functions. If η(x) is a local field variable in a solid body occupying a domain V, the corresponding non-local field variable ηV(x) is defined as:

 

where α′(x, ξ) is a weight function that satisfies the partition of unity and depends on an internal characteristic length lc. The length lc can be determined experimentally by comparing the responses of specimens in which the damage remains distributed with the response of fractured specimens in which damage localizes (Bazant and Pijaudier-Cabot, 1989). It can also be determined by comparing the simulation results for various values of lc with the experimental response (Geers et al., 1999). Integral non-local regularization was performed on the averaged energy release rate (Pijaudier-Cabot and Bazant, 1987), the damage variable (Bazant and Pijaudier-Cabot, 1988), the equivalent strain (Bazant and Lin, 1988), the specific fracture strain (Pijaudier-Cabot and Bazant, 1987), the inelastic stress (Jirasek, 1998), the inelastic stress rate (Jirasek, 1998) and the inelastic stress calculated from the non-local strain (Bazant et al., 1996). Jirasek (Jirasek, 1998) demonstrated that only averaging the equivalent strain, the energy release rate or the specific fracturing strain can correctly reproduce large post-peak deformation or complete fracture. Other integral non-local models lead to spurious residual stresses and to a dilation of the softening zone. Of note, phase-field fracture propagation models are numerically equivalent to integral non-local isotropic damage models and mathematically similar to gradient-enhanced damage models (Planas et al., 1993; Voyiadjis and Mozaffari, 2013; Ambati et al., 2015). Phase-field methods are computationally efficient but remain challenging to use for predicting fracture surfaces explicitly.

In my group, we used an integral non-local formulation to model geomaterials that exhibit tensile softening (Jin and Arson, 2018). The equation of the free energy was similar to the expression of Ψ above, with different coefficients for open and closed cracks. In order to account for the non-local nature of damage, the equivalent strains that control damage evolution was replaced by their weighted average defined on an influence domain V, as follows:

where x is the position vector of the material point considered, and ξ is the position vector of points in the influence domain of x. α′(x, ξ) is the normalized Bell-shaped function. Figure 5 shows the results of FEM simulations of three-point bending tests, with the local and the non-local formulations of the damage model and for two mesh refinements. The internal length parameter in these simulations was lc = 0.01 m. The element size was 0.065 m for the coarse mesh and about 0.002 m for the fine mesh, so that the ratio characteristic length to element size was 0.15 for the coarse mesh and 5 for the fine mesh. Results show that non-local enhancement avoids mesh dependency during crack development: the width of the process zone is the same for both mesh refinements (marked with a rectangle in Figure 5).

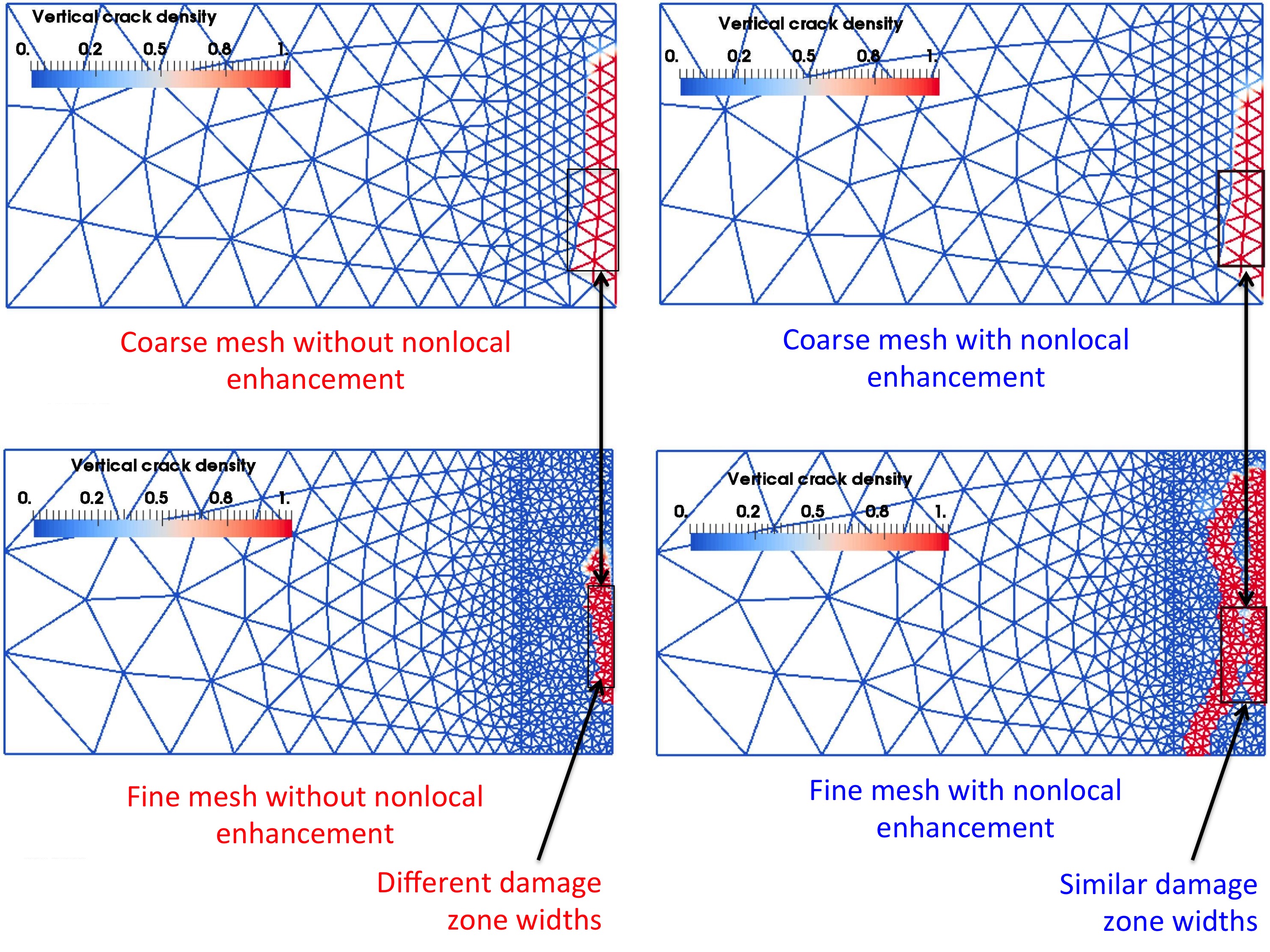
Figure 5. Horizontal damage component (i.e. vertical crack density) obtained by simulating a three-point bending test without and with non-local enhancement, for various mesh densities. Only one half of the beam is shown. Figure 8 from (Arson, 2020), article licensed under the Creative Commons Attribution Non-Commercial Share Alike 4.0 License.

 

Differential formulations

In differential formulations (de Borst et al., 1999; Bazant and Jirasek, 2002), local field variables are developed in Taylor series (De Vree et al., 1995; Peerlings et al., 1996; Askes et al., 2000; Askes and Sluys, 2002):

Δ2 denotes the Laplacian operator and the coefficients ci depend on the weight function α and on the averaging volume V. The coefficients ci can be expressed explicitly in terms of lc if the weight function is a Gaussian distribution. The implicit second-order scheme, expressed as:

is widely invoked to simulate softening in problems of dynamics (De Borst et al., 1995; Askes et al., 2000), brittle damage (De Borst et al., 1995; De Vree et al., 1995; Askes and Sluys, 2002) and plasticity (De Borst et al., 1995; de Borst et al., 1999). The parameter ζ with the dimension of a length squared is related to the internal length. As indicated by Geers et al. (Geers et al., 1998), applying the equation above with a constant ζ provides inconsistent predictions in mode I crack propagation, because the damage zone becomes wider and wider in a direction perpendicular to the crack, where the material should unload. It is possible to overcome this limitation by accounting for the transient behavior of the gradient parameter during damage evolution.

An important limitation of the non-local damage models is the lack or relationship between the internal length parameter and characteristic dimensions of the material components. Bazant and Pijaudier-Cabot (Bazant and Pijaudier-Cabot, 1989) set an internal length equal to 3 times the maximum aggregate size in concrete while using an integral non local approach. However, this number was chosen based on experiments conducted on only one type of concrete material. Giry et al. (Giry et al., 2011) and Vandoren and Simone (Vandoren and Simone, 2018) proposed to relate a variable internal length to both the characteristic size of the material and the stress field. A fiber bundle model (Villette et al., 2020) recently explored the effect of material characteristic length on the size of the fracture process zone. In a fiber bundle model, the material is seen as an assembly of fibers that can break upon traction. When Δ fibers get broken at constant displacement and only through the redistribution of forces, this is called an avalanche of size Δ. Material damage is quantified based on the number of broken fibers. The statistical damage due to the propagation of a cohesive crack embedded in the fiber was studied by introducing a characteristic length by means of a spatially correlated field of fiber tensile strength, represented by an auto-correlated random process. It was found that the avalanche distribution is sensitive to the auto-correlation length, and that a transitional regime of avalanche distributions appears if the characteristic size of the material heterogeneities is small enough compared to the (finite) characteristic size of the shape of the stress field.

Non-local formulations not only avoid non-physical localization of damage and strains, but also capture the dependence of mechanical behavior on specimen size. The key is the introduction of an internal length parameter, which scales the size of the zone of influence of local field variables. The next sections discuss two alternatives to the non-local formulations: the theory of micromorphic media and the stochastic gradient plasticity framework.

 

Theory of micromorphic media

The theory of micromorphic media introduces additional degrees of freedom for microscopic translations and rotations. Toupin (Toupin, 1964), Mindlin (Mindlin, 1964; Mindlin and Eshel, 1968) and Germain (Germain, 1973) developed the theory of microstructure enriched elasticity. Vernerey and collaborators (Vernerey et al., 2007) extended the formulation to microstructure enriched elastoplasticity for hierarchical materials. The microscopic displacement, noted u′i(x′i), is defined in reference to the macroscopic displacement, noted ui(xi). Expanding u′ i(x′i) in Taylor’s series and truncating the higher order terms, one gets the microscopic displacement field of a so-called micromorphic continuum of degree one: u′i = ui + χijx′j . The kinematic description of a micromorphic continuum of degree one only depends on the macroscopic displacement field ui and on the gradient of micro-displacement ∂ju′i = γij . By definition, γij is a second order tensor, the symmetric part of which, (γij + γji)/2, is called the micro-strain rate tensor, and the anti-symmetric part of which, (γij − γji)/2, is named the micro-rotation rate tensor. Noting the macroscopic strain ϵij = (∂iuj + ∂jui)/2, the relative deformation ηij = ∂iuj − γij and the microdeformation gradient κijk = ∂iγjk = γij,k, the volumetric Helmholtz free energy ψ can be expressed in terms of the deformation tensors ϵij , ηij , κijk. The work-conjugated stress variables are respectively the Cauchy symmetric stress σij , the micro-structure relative stress tensor sij , and the third order double stress tensor νijk.

Micro-polar media, governed by Cosserat models (Cosserat and Cosserat, 1909), are a particular case of micromorphic medium of degree one, in which only micro-rotations are accounted for (i.e. microscopic translations are ignored) (Matsushima et al., 2000; Chambon et al., 2001; Vernerey et al., 2007). Second gradient models are also models of micromorphic media of degree one, in which it is assumed that the gradient of micro deformation is equal to the macro deformation. The micropolar and second gradient theories were successfully applied to rocks (Tamagnini et al., 2001; Sulem and Vardoulakis, 2014) and granular materials (Chambon et al., 2001). To circumvent the scale effects in conventional damage models with softening, continuum damage mechanics models were enhanced with the gradient of strain (Zhou et al., 2002; Zhao et al., 2005). The conventional strain and the strain gradient are split into an undamaged elastic part and a damaged irreversible part, and the increment of work-conjugated Cauchy stress and higher order stress are updated thanks to the damage yield function and the damage potential.

Most gradient plasticity theories rely on a generalized plastic strain expressed as a function of the local plastic strain and its high-order gradients (Voyiadjis and Al-Rub, 2005):

in which l is a length parameter. The interaction coefficients γ1, γ2 and γ3 (which control how ϵp and ∇nϵp are coupled) are typically found by deriving a set of dislocation mechanics-based equations. g (ln∇nϵp) is the measure of the effective plastic strain gradient of order n. The equation above should ensure that ϵp → ϵp whenever ϵp >> l ∇ϵp and that ϵp → l ∇ϵp whenever ϵp << l ∇ϵp. In the gradient plasticity theory introduced by Fleck and Hutchinson (Fleck and Hutchinson, 1993; Fleck et al., 1994; Hutchinson and Fleck, 1997; Begley and Hutchinson, 1998; Fleck and Hutchinson, 2001), γ1 = 2m, γ2 = γ3 = 2, f (ϵp) = (ϵp)^(1/m) and g (ln∇nϵp) = l η, with l η = c1 (∇ϵp)iik (∇ϵp)jjk +c2 (∇ϵp)ijk (∇ϵp)ijk +c3 (∇ϵp)ijk (∇ϵp)kji, in which the material parameters c1, c2 and c3 are of length-square dimension. The first gradient of the plastic strain ∇ϵp is alternatively defined as the second-order gradient of the displacement field, ∇.∇u. The mechanism-based strain gradient plasticity theory and the Taylor-based non-local theory proposed by Gao and co-workers (e.g., (Nix and Gao, 1998; Gao et al., 1999; Huang et al., 2000; Gao and Huang, 2001; Guo et al., 2001; Hwang et al., 2002; Qiu et al., 2003; Huang et al., 2004)) are based on the framework proposed by Fleck and Hutchinson and correspond to the case γ1 = 2, γ2 = 1, γ3 = 2/m, f (ϵp) = (ϵp)^(1/m) and g (ln∇nϵp) = lη, where η = √[(∇ϵp)jjk (∇ϵp)jjk /4] and in which l is a length parameter that depends on the shear modulus and Burgers vector of the material.

 

Stochastic gradient plasticity framework

The main inconvenient of the gradient plasticity models that are formulated within the theory of micromorphic media is that they depend on a third- or higher-order stress tensors that are work conjugate to ∇nϵp (n being the degree of the micromorphic medium), for which higher-order surface tractions are typically not uniquely defined or difficult to satisfy when solving boundary-value problems (Voyiadjis and Al-Rub, 2005). Furthermore, it was noted by Bazant and Guo (Bazant and Guo, 2002) that the asymptotic behavior at small sizes is too strong due to the presence of third-order stresses in the formulation. That is why stochastic gradient plasticity frameworks are attractive to understand the non-local effects of micro-mechanical processes such as dislocations on the macroscopic behavior of a cracked REV.

The theory of stochastic gradient plasticity was initially proposed by Aifantis and collaborators (Aifantis, 1984, 1987; Zbib and Aifantis, 1988; Tsagrakis and Aifantis, 2002; Zbib and Aifantis, 2003) and then used by other authors (e.g., (Yang et al., 2011; Segura Valdivieso, 2017)). In this theory, the evolution of the deformation field depends on elastic deformation and on the production, annihilation and motion of defects (such as cracks or dislocations). The plastic strain is expressed as a function of the densities of these defects, based on geometric or phenomenological considerations. The starting point is to write a conservation equation for the density of each family of defects. For the kth family of defects of density ρk:

in which Jk is the flux of the defects of the kth family, Fk is a source term, vk is the velocity of the kth family of defects and Dk is a diffusion coefficient that accounts for random influences on the motion of these defects. Gkw˙k is an additional stochastic term, where each variable w˙k represents a random processes, for instance large intrinsic fluctuations of the disclocation velocity (or local slip rate). Tsagrakis and Aifantis (Tsagrakis and Aifantis, 2002) showed that for a single family of gliding dislocations, the equation above may be rewritten in terms of plastic strain. In one dimension, noting γ the plastic shear strain:

in which μ and c are a viscoplastic-like coefficient and a gradient coefficient respectively, σext is the external stress, σint is the internal stress with mean value σint and fluctuations δσint. In the absence of internal stress fluctuations (δσint = 0) or viscoplastic strains (μ = 0), one gets the simplest stochastic gradient plasticity model, in which σext = σint(γ) − c∂^2γ/∂x^2 . Noting the constitutive relationship between stress and strain as σint(γ) = κ(γ) and expressing σext in terms of equivalent (Von Mises) stress τ:

If one assumes that the strain γ is a function of a random microstrain variable Γ, a Taylor expansion of the mean ⟨Γ⟩ yields an expression that depends on the autocorrelation function of the microstrains C(r) that depends on a correlation length l, which plays the role of an internal length parameter in a non-local formulation or a micromorphic continuum mechanics framework. For a Gaussian auto-correlation function, C(r) = exp[−(r/l)^2]. A phenomenological generalization of the equation above was proposed to include the first-order strain gradient, as follows:

in which σ and ϵ are the Von Mises equivalent stress and strain, respectively and c1 and c2 are phenomenological functions of the equivalent strain. In the equation above, the gradient ∇ϵ denotes either the second gradient of the displacement field (“deformation version” of gradient plasticity) or the first gradient of the plastic strain tensor (“flow version” of gradient plasticity).

Abu Al Rub and Voyiadjis (Voyiadjis and Al-Rub, 2005) noted that the gradient plasticity framework as laid out in the following equation:

does not provide satisfactory interpretations of size effects when a fixed internal length parameter l is employed and proposed instead to make it a function of microstructure parameters that vary with plastic deformation. Based on the Taylor model in dislocation mechanics, they showed that l is in the order of the average distance between dislocations (noted Ls), as follows:

bS and bG are the magnitudes of the Burgers vectors associated with the statistically stored dislocations (SSDs) and geometrically necessary dislocations (GNDs), respectively, αS and αG are statistical coefficients which account for the deviation from the regular spatial arrangements of the SSD and GND populations, respectively, r is the Nye factor and M is the Schmidt’s orientation factor. It was later argued that Ls depends on plastic deformation, and the authors revised the equation above as follows:

in which d is the mean grain size and D is the characteristic size of the REV. In the absence of plastic strain, l = h d, which implies that the mean dislocation path in the absence of accumulated plastic strain is equivalent to the grain size. The concept of variable internal length parameter was used by other authors (e.g., (Faghihi and Voyiadjis, 2012; Zhao et al., 2015; Zhang and Aifantis, 2015)) and allowed an interpretation of macroscopic behavior of damaged media in terms of dislocations and pile ups.

 

Concluding remarks

In top-down modeling approaches, REV-scale state equations are derived from thermodynamic conjugation relationships. In fabric-enriched models, the damage variable is a convolution of moments of probability of microstructure descriptors of characteristic sizes that define internal lengths. Non-local continuum models are based on REV-scale evolution laws for strains and damage, and at least one state or internal variable is defined by developing a local variable in a Taylor’s series or by integrating that variable on a control volume. The internal length in that case is the length that intervenes in the differential development or the size of the control volume. Micromorphic media are based on a balance of energy written in terms of the REV-scale state variables and their micro-scale counterparts. The calculation requires expanding the local displacement field into a Taylor’s series which again, involves an internal length parameter. When only the first derivative of the local displacement field is conserved in the series, one obtains a second-order gradient model. Second-order gradient formulations using a non-local plastic strain tensor were often used in the gradient plasticity theory, in which the internal length parameter typically depends on the shear modulus and Burgers vector of the material. In the stochastic gradient plasticity framework, the plastic strain is expressed as a function of the densities of defects such as cracks or dislocations. The REV-scale strain is a function of a random microstrain variable, the mean of which depends on an auto-correlation function that depends on a correlation length, which plays the role of internal length.

Continuum mechanics models are specific to the REV scale at which are they are formulated, precisely because they depend on internal length parameters that have a physical meaning. Stochastic gradient plasticity approaches do account for variable internal lengths, but the evolution laws of those internal lengths are only valid so long as large localization zones do not exist. Continuum approaches break down when the internal length that characterizes the microstructure changes in order of magnitude, which typically means that the REV needs to be adjusted in size. Crack coalescence has been studied extensively in rock mechanics. Boundary Element simulations highlighted the occurrence of tensile vs. shear driven coalescence from the tips of pre-existing flaws. In metals, void coalescence and subsequent ductile fracture propagation were mostly studied through micromechanical models formulated at the scale of a unit cell containing one or few voids, or through numerical approaches, mainly, the Finite Element Method (FEM) and phase field methods. The interaction between a diffuse crack distribution and a larger fracture, which can be seen as the interaction between coalesced cracks and other cracks, was studied by combining continuum mechanics models of damage in the FEM with cohesive fracture models, either through the extended FEM (XFEM) or by means cohesive Zone Models (CZMs). My group’s work on that topic was published in (Jin et al., 2017; Jin and Arson, 2019, 2020).

Figure 6 summarizes the main analytical, numerical and experimental tools available to date to predict the propagation of a single fracture or a distribution of cracks. Although there exit several methods to couple microstructure to macroscopic damage, there is no method in the current body of literature to predict the response of a microscopic specimen that contains cracks with a length of the order of 10 nanometers (“grey area” in Figure 6). At present, statistical mechanics is too computationally expensive to simulate micro-scale domains from the interaction of atoms or molecules in that domain. Micromechanics relies on Griffith’s theory, which cannot be unconditionally applied at the scale of a few nanometers.

Figure 6. The main tools to analyze and predict fracture propagation across scales, and the links between them. Legend: SEL: Scaling Effects Laws. MD: Molecular Dynamics. LSO: Local Structure Optimization. SGCMC: Semi-Grand Canonical Monte Carlo.

 

References

Aifantis, E. C. (1984). On the microstructural origin of certain inelastic models. J. Eng. Mater. Technol. 106, 326–330

Aifantis, E. C. (1987). The physics of plastic deformation. International journal of plasticity 3, 211–247

Ambati, M., Gerasimov, T., and De Lorenzis, L. (2015). A review on phase-field models of brittle fracture and a new fast hybrid formulation. Computational Mechanics 55, 383–405

Arson, C. (2020). Micro-macro mechanics of damage and healing in rocks. Open Geomechanics 2, 1–41

Askes, H., Pamin, J., and de Borst, R. (2000). Dispersion analysis and element-free galerkin solutions of second-and fourth-order gradient-enhanced damage models. International Journal for Numerical Methods in Engineering 49, 811–832

Askes, H. and Sluys, L. (2002). Explicit and implicit gradient series in damage mechanics. European Journal of Mechanics-A/Solids 21, 379–390

Bazant, Z. P. and Guo, Z. (2002). Size effect and asymptotic matching approximations in strain-gradient theories of micro-scale plasticity. International Journal of Solids and Structures 39, 5633–5657

Bazant, Z. P. and Jirasek, M. (2002). Nonlocal integral formulations of plasticity and damage: survey of progress. Journal of Engineering Mechanics 128, 1119–1149

Bazant, Z. P. and Lin, F.-B. (1988). Nonlocal smeared cracking model for concrete fracture. Journal of Structural Engineering 114, 2493–2510

Bazant, Z. P. and Oh, B. H. (1985). Microplane model for progressive fracture of concrete and rock. Journal of Engineering Mechanics 111, 559–582

Bazant, Z. P. and Oˇzbolt, J. (1990). Nonlocal microplane model for fracture, damage, and size effect in structures. Journal of Engineering Mechanics 116, 2485–2505

Bazant, Z. P. and Pijaudier-Cabot, G. (1988). Nonlocal continuum damage, localization instability and convergence. Journal of applied mechanics 55, 287–293

Bazant, Z. P. and Pijaudier-Cabot, G. (1989). Measurement of characteristic length of nonlocal continuum. Journal of Engineering Mechanics 115, 755–767

Bazant, Z. P. and Prat, P. C. (1988). Microplane model for brittle-plastic material: I. theory. Journal of Engineering Mechanics 114, 1672–1688

Bazant, Z. P., Xiang, Y., and Prat, P. C. (1996). Microplane model for concrete. i: Stress-strain boundaries and finite strain. Journal of Engineering Mechanics 122, 245–254

Begley, M. R. and Hutchinson, J. W. (1998). The mechanics of size-dependent indentation. Journal of the Mechanics and Physics of Solids 46, 2049–2068

Blum, W. and Eisenlohr, P. (2009). Dislocation mechanics of creep. Materials Science and Engineering: A, 510, 7–13

Chambon, R., Caillerie, D., and Matsuchima, T. (2001). Plastic continuum with microstructure, local second gradient theories for geomaterials: localization studies. International Journal of Solids and Structures 38, 8503–8527

Chester, J. S., Lenz, S. C., Chester, F. M., and Lang, R. (2004). Mechanisms of compaction of quartz sand at diagenetic conditions. Earth and Planetary Science Letters 220, 435–451

Cosserat, E. and Cosserat, F. (1909). Théorie des corps déformables (A. Hermann et fils,)

Dafalias, Y. F. and Manzari, M. T. (2004). Simple plasticity sand model accounting for fabric change effects. Journal of Engineering mechanics 130, 622–634

Dafalias, Y. F., Papadimitriou, A. G., and Li, X. S. (2004). Sand plasticity model accounting for inherent fabric anisotropy. Journal of Engineering Mechanics 130, 1319–1333

de Borst, R., Pamin, J., and Geers, M. G. (1999). On coupled gradient-dependent plasticity and damage theories with a view to localization analysis. European Journal of Mechanics-A/Solids 18, 939–962

De Borst, R., Pamin, J., Peerlings, R., and Sluys, L. (1995). On gradient-enhanced damage and plasticity models for failure in quasi-brittle and frictional materials. Computational Mechanics 17, 130–141

De Vree, J., Brekelmans, W., and Van Gils, M. (1995). Comparison of nonlocal approaches in continuum damage mechanics. Computers & Structures 55, 581–588

Dormieux, L., Kondo, D., and Ulm, F.-J. (2006). Microporomechanics (John Wiley & Sons)

Faghihi, D. and Voyiadjis, G. Z. (2012). Thermal and mechanical responses of bcc metals to the fasttransient process in small volumes. Journal of Nanomechanics and Micromechanics 2, 29–41

Fleck, N. and Hutchinson, J. (1993). A phenomenological theory for strain gradient effects in plasticity. Journal of the Mechanics and Physics of Solids 41, 1825–1857

Fleck, N. and Hutchinson, J. (2001). A reformulation of strain gradient plasticity. Journal of the Mechanics and Physics of Solids 49, 2245–2271

Fleck, N., Muller, G., Ashby, M. F., and Hutchinson, J. W. (1994). Strain gradient plasticity: theory and experiment. Acta Metallurgica et materialia 42, 475–487

Fu, P. and Dafalias, Y. F. (2011). Fabric evolution within shear bands of granular materials and its relation to critical state theory. International Journal for numerical and analytical methods in geomechanics 35, 1918–1948

Gambarotta, L. and Lagomarsino, S. (1993). A microcrack damage model for brittle materials. International Journal of Solids and Structures 30, 177–198

Gao, H. and Huang, Y. (2001). Taylor-based nonlocal theory of plasticity. International Journal of Solids and Structures 38, 2615–2637

Gao, H., Huang, Y., and Nix, W. (1999). Modeling plasticity at the micrometer scale. Naturwissenschaften, 86, 507–515

Gao, Z., Zhao, J., Li, X.-S., and Dafalias, Y. F. (2014). A critical state sand plasticity model accounting for fabric evolution. International journal for numerical and analytical methods in geomechanics 38, 370–390

Geers, M., De Borst, R., Brekelmans, W., and Peerlings, R. (1998). Strain-based transient-gradient damage model for failure analyses. Computer methods in applied mechanics and engineering 160, 133–153

Geers, M., De Borst, R., Brekelmans, W., and Peerlings, R. (1999). Validation and internal length scale determination for a gradient damage model: application to short glass-fibre-reinforced polypropylene. International Journal of Solids and Structures 36, 2557–2583

Germain, P. (1973). The method of virtual power in continuum mechanics. part 2: Microstructure. SIAM Journal on Applied Mathematics 25, 556–575

Giry, C., Dufour, F., and Mazars, J. (2011). Stress-based nonlocal damage model. International Journal of Solids and Structures 48, 3431–3443

Guo, Y., Huang, Y., Gao, H., Zhuang, Z., and Hwang, K. (2001). Taylor-based nonlocal theory of plasticity: numerical studies of the micro-indentation experiments and crack tip fields. International Journal of Solids and Structures 38, 7447–7460

Huang, Y., Qu, S., Hwang, K., Li, M., and Gao, H. (2004). A conventional theory of mechanism-based strain gradient plasticity. International Journal of Plasticity 20, 753–782

Huang, Y., Xue, Z., Gao, H., Nix, W., and Xia, Z. (2000). A study of microindentation hardness tests by mechanism-based strain gradient plasticity. Journal of Materials Research 15, 1786–1796

Hutchinson, J. and Fleck, N. (1997). Strain gradient plasticity. Advances in applied mechanics 33, 295–361

Hwang, K., Jiang, H., Huang, Y., Gao, H., and Hu, N. (2002). A finite deformation theory of strain gradient plasticity. Journal of the Mechanics and Physics of Solids 50, 81–99

Jin, W. and Arson, C. (2018). Nonlocal enrichment of a micromechanical damage model with tensile softening: Advantages and limitations. Computers and Geotechnics 94, 196–206

Jin, W. and Arson, C. (2019). Xfem to couple nonlocal micromechanics damage with discrete mode I cohesive fracture. Computer Methods in Applied Mechanics and Engineering 357, 112617

Jin, W. and Arson, C. (2020). Fluid-driven transition from damage to fracture in anisotropic porous media: a multi-scale xfem approach. Acta Geotechnica 15, 113–144

Jin, W., Xu, H., Arson, C., and Busetti, S. (2017). Computational model coupling mode ii discrete fracture propagation with continuum damage zone evolution. International Journal for Numerical and Analytical Methods in Geomechanics 41, 223–250

Jirasek, M. (1998). Nonlocal models for damage and fracture: comparison of approaches. International Journal of Solids and Structures 35, 4133–4145

Ju, J. (1991). On two-dimensinal self-consistent micromechanical damage models for brittle solids. International Journal of Solids and Structures 27, 227–258

Kachanov, M. (1980). Continuum model of medium with cracks. Journal of the engineering mechanics division 106, 1039–1051

Kachanov, M. (1992). Effective elastic properties of cracked solids: critical review of some basic concepts. Applied Mechanics Reviews 45, 304–335

Kachanov, M. L. (1982a). A microcrack model of rock inelasticity part i: Frictional sliding on microcracks. Mechanics of Materials 1, 19–27

Kachanov, M. L. (1982b). A microcrack model of rock inelasticity part ii: propagation of microcracks. Mechanics of Materials 1, 29–41

Krajcinovic, D., Basista, M., and Sumarac, D. (1991). Micromechanically inspired phenomenological damage model. Journal of Applied Mechanics 58, 305–310

Krajcinovic, D. and Sumarac, D. (1989). A mesomechanical model for brittle deformation processes: Part 2. Journal of Applied Mechanics 56, 57

Li, X. and Li, X.-S. (2009). Micro-macro quantification of the internal structure of granular materials. Journal of engineering mechanics 135, 641–656

Li, X. S. and Dafalias, Y. F. (2011). Anisotropic critical state theory: role of fabric. Journal of Engineering Mechanics 138, 263–275

Long, J., Remer, J., Wilson, C., and Witherspoon, P. (1982). Porous media equivalents for networks of discontinuous fractures. Water Resources Research 18, 645–658

Lu, B. and Torquato, S. (1992a). Lineal-path function for random heterogeneous materials. Physical Review A 45, 922

Lu, B. and Torquato, S. (1992b). Nearest-surface distribution functions for polydispersed particle systems. Physical Review A 45, 5530

Lubarda, V., Krajcinovic, D., and Mastilovic, S. (1994). Damage model for brittle elastic solids with unequal tensile and compressive strengths. Engineering Fracture Mechanics 49, 681–697

Maleki, K. and Pouya, A. (2010). Numerical simulation of damage–permeability relationship in brittle geomaterials. Computers and Geotechnics 37, 619–628

Matsushima, T., Chambon, R., and Caillerie, D. (2000). Second gradient models as a particular case of microstructured models: a large strain finite elements analysis. Comptes Rendus de l’Académie des Sciences-Series IIB-Mechanics-Physics-Astronomy 328, 179–186

Mindlin, R. D. (1964). Micro-structure in linear elasticity. Archive for Rational Mechanics and Analysis 16, 51–78

Mindlin, R. D. and Eshel, N. (1968). On first strain-gradient theories in linear elasticity. International Journal of Solids and Structures 4, 109–124

Nix, W. D. and Gao, H. (1998). Indentation size effects in crystalline materials: a law for strain gradient plasticity. Journal of the Mechanics and Physics of Solids 46, 411–425

Paliwal, B. and Ramesh, K. (2008). An interacting micro-crack damage model for failure of brittle materials under compression. Journal of the Mechanics and Physics of Solids 56, 896–923

Peerlings, R. H., de Borst, R., Brekelmans, W. M., and De Vree, J. (1996). Gradient enhanced damage for quasi-brittle materials. International Journal for numerical methods in engineering 39, 3391–3403

Pensée, V. and Kondo, D. (2003). Micromechanics of anisotropic brittle damage: comparative analysis between a stress based and a strain based formulation. Mechanics of materials 35, 747–761

Pensée, V., Kondo, D., and Dormieux, L. (2002). Micromechanical analysis of anisotropic damage in brittle materials. Journal of Engineering Mechanics 128, 889–897

Philleo, R. (1983). A method for analyzing void distribution in air-entrained concrete. Cement, concrete and aggregates 5, 128–130

Pijaudier-Cabot, G. and Bazant, Z. P. (1987). Nonlocal damage theory. Journal of engineering mechanics, 113, 1512–1533

Planas, J., Elices, M., and Guinea, G. V. (1993). Cohesive cracks versus nonlocal models: Closing the gap. International Journal of Fracture 63, 173–187

Pouya, A., Zhu, C., and Arson, C. (2016). Micro–macro approach of salt viscous fatigue under cyclic loading. Mechanics of Materials 93, 13–31

Qiu, X., Huang, Y., Wei, Y., Gao, H., and Hwang, K. (2003). The flow theory of mechanism-based strain gradient plasticity. Mechanics of Materials 35, 245–258

Reid, W. P. (1955). Distribution of sizes of spheres in a solid from a study of slices of the solid. Journal of Mathematics and Physics 34, 95–102

Schubnel, A., Benson, P. M., Thompson, B. D., Hazzard, J. F., and Young, R. P. (2006). Quantifying damage, saturation and anisotropy in cracked rocks by inverting elastic wave velocities. Pure and Applied Geophysics 163, 947–973

Segura Valdivieso, G. (2017). Computational modelling of fracture: gradient damage models with variable internal length. B.S. thesis, Universitat Polit`ecnica de Catalunya

Shen, X. and Arson, C. (2019). An isotropic self-consistent homogenization scheme for chemo-mechanical healing driven by pressure solution in halite. International Journal of Solids and Structures 161, 96–110

Shen, X., Arson, C., Ferrier, K. L., West, N., and Dai, S. (2019). Mineral weathering and bedrock weakening: Modeling microscale bedrock damage under biotite weathering. Journal of Geophysical Research: Earth Surface 124, 2623–2646

Shen, X., Ding, J., Arson, C., Chester, J. S., and Chester, F. M. (2021a). Micromechanical modeling for rate-dependent behavior of salt rock under cyclic loading. International Journal for Numerical and Analytical Methods in Geomechanics 45, 28–44

Shen, X., Ding, J., Lordkipanidze, I., Arson, C., Chester, J. S., and Chester, F. M. (2021b). Fabric evolution and crack propagation in salt during consolidation and cyclic compression tests. Acta Geotechnica 16, 1679–1697

Sulem, J. and Vardoulakis, I. (2014). Bifurcation analysis in geomechanics (CRC Press)

Tamagnini, C., Chambon, R., and Caillerie, D. (2001). A second gradient elastoplastic cohesive-frictional model for geomaterials. Comptes Rendus de l’AcadÅLemie des Sciences-Series IIB-Mechanics 329, 735–739

Torquato, S. and Lu, B. (1993). Chord-length distribution function for two-phase random media. Physical Review E 47, 2950

Torquato, S., Lu, B., and Rubinstein, J. (1990). Nearest-neighbor distribution functions in many-body systems. Physical Review A 41, 2059

Toupin, R. A. (1964). Theories of elasticity with couple-stress. Archive for Rational Mechanics and Analysis 17, 85–112

Tsagrakis, I. and Aifantis, E. C. (2002). Recent developments in gradient plasticity - part I: formulation and size effects. J. Eng. Mater. Technol. 124, 352–357

Turner, C. H. and Cowin, S. C. (1987). Dependence of elastic constants of an anisotropic porous material upon porosity and fabric. Journal of materials science 22, 3178–3184

Vandoren, B. and Simone, A. (2018). Modeling and simulation of quasi-brittle failure with continuous anisotropic stress-based gradient-enhanced damage models. Computer Methods in Applied Mechanics and Engineering 332, 644–685

Vernerey, F., Liu, W. K., and Moran, B. (2007). Multi-scale micromorphic theory for hierarchical materials. Journal of the Mechanics and Physics of Solids 55, 2603–2651

Vernon, R. H. (2018). A practical guide to rock microstructure (Cambridge university press)

Villette, F., Baroth, J., Dufour, F., Bloch, J.-F., and Du Roscoat, S. R. (2020). Influence of material heterogeneities on crack propagation statistics using a fiber bundle model. International Journal of Fracture 221, 87–100

Voyiadjis, G. Z. and Al-Rub, R. K. A. (2005). Gradient plasticity theory with a variable length scale parameter. International Journal of solids and structures 42, 3998–4029

Voyiadjis, G. Z. and Mozaffari, N. (2013). Nonlocal damage model using the phase field method: theory and applications. International Journal of Solids and Structures 50, 3136–3151

Wilson, J., Chester, J., and Chester, F. (2003). Microfracture analysis of fault growth and wear processes, punchbowl fault, san andreas system, california. Journal of Structural Geology 25, 1855–1873

Xu, T. and Arson, C. (2022). Self-consistent approach for modeling coupled elastic and visco-plastic processes induced by dislocation and pressure solution. International Journal of Solids and Structures, 238, 111376

Xu, T. and Arson, C. (2023). Interface homogenization approach for mechanical healing driven by pressure solution. Journal of Engineering Mechanics 149. doi:10.1061/JENMDT/EMENG-7079

Xu, T., Shen, X., Reed, M., West, N., Ferrier, K. L., and Arson, C. (2022). Anisotropy and microcrack propagation induced by weathering, regional stresses and topographic stresses. Journal of Geophysical Research: Solid Earth 127, e2022JB024518

Yang, Q., Zhou, W., and Swoboda, G. (1999). Micromechanical identification of anisotropic damage evolution laws. International journal of fracture 98, 55–76

Yang, Y., Ching, W., and Misra, A. (2011). Higher-order continuum theory applied to fracture simulation of nanoscale intergranular glassy film. Journal of Nanomechanics and Micromechanics 1, 60–71

Zbib, H. and Aifantis, E. (1988). On the localization and postlocalization behavior of plastic deformation. I. On the initiation of shear bands. Res Mechanica 23, 261–277

Zbib, H. and Aifantis, E. (2003). Size effects and length scales in gradient plasticity and dislocation dynamics. Scripta materialia 48, 155–160

Zhang, X. and Aifantis, K. (2015). Interpreting the internal length scale in strain gradient plasticity. Rev. Adv. Mater. Sci 41, 72–83

Zhao, J., Sheng, D., and Zhou, W. (2005). Shear banding analysis of geomaterials by strain gradient enhanced damage model. International journal of solids and structures 42, 5335–5355

Zhao, J., Zhang, X., Konstantinidis, A. A., and Kang, G. (2015). Correlating the internal length in strain gradient plasticity theory with the microstructure of material. Philosophical Magazine Letters 95, 340–349

Zhou, W., Zhao, J., Liu, Y., and Yang, Q. (2002). Simulation of localization failure with straingradient- enhanced damage mechanics. International journal for numerical and analytical methods in geomechanics 26, 793–813

Zysset, P. and Curnier, A. (1995). An alternative model for anisotropic elasticity based on fabric tensors. Mechanics of Materials 21, 243–250