You are here
Contact radius of spherical indenter
Looking through books and papers I see an often quoted equation to show that the contact radius (a) of spherical indenter of radius (R) is related to the indentation depth (h):
a= √(R.h)
However, using simple trigonometry of a spherical cap it can be shown that:
a=√(2Rh-h2)
Contact area is very important for use in nanoindentation - however, if it is based on the wrong contact area calculation, then more errors become apparent.
On that note - I also want to ask if a rigid flat indenter press on a thin shell sphere, is the contact radius the same as a rigid spherical indenter pressing on a flat surface ?
Colin
- ColinGrant's blog
- Log in or register to post comments
- 16145 reads

Comments
Hello,The second equation
Hello,
The second equation is the correct one for a simple approximation.
But, in reality, pile-up or sink-in effect can occur and affects the
previous relation. Other approaches can then be used to evaluate, more
accurately, the contact radius (a) depending on the tested material.
Concerning your last question, the contact radii will be clearly
different.
Guenhael.
It is not a pure geometric
It is not a pure geometric problem.
The first equation is the result of elasticity.
Please see the following schematic: where a=sqrt(Rh)
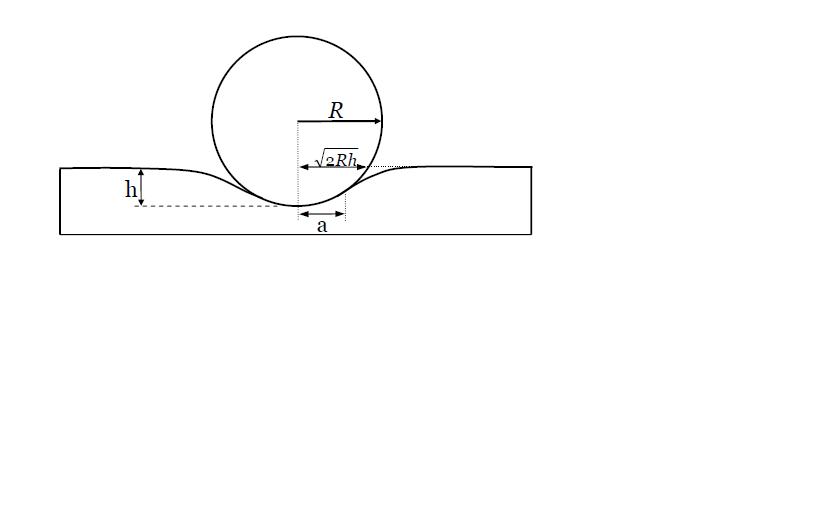
Your explanation makes
Your explanation makes sense. I thought it was because of the sphere profile approximation.
Could you please tell me where I can find the detailed procedures to develop the equation?
Thank you.
In addition, The
In addition,
The answer of your last question was yes if you were considering the particular case of elasticity (according to the Hertz theory).
Guenhael.
The answer depends on geometry and elasticity
Hi,
The contact radius between two objects depend on their material
properties (e.g. elasticity) and on their geometry. If you press a rigid
sphere into a fluid then after a while the wet area has radius a =
sqrt(2Rh-h^2). However, if you press a rigid sphere onto an elastic
block ("half-space") then the contact area will be smaller. And if the
sphere is elastic as well, then the contact area will be in between.
These latter two situations are described by Hertz' theory, which says
that a = sqrt(Rh). See http://en.wikipedia.org/wiki/Frictional_contact_mechanics and http://en.wikipedia.org/wiki/Contact_mechanics and the books that are cited there.
The situation is different for plates and shells than for massive materials (half-spaces). I don't know much about shells.
Best wishes, Edwin