You are here
How to compute the atomic stress objectively?
Atomistic simulation has been a powerful study tool in mechanics research, but how to objectively compute the atomic stress equivalent to Cauchy stress is still controversial, especially on the velocity-related part in the virial stress definition. In this paper, by strictly following the classical definition of the Cauchy stress for continuum medium, the fundamental Lagrangian atomic stress is proposed and can be used to obtain the correct Cauchy stress under any circumstances. Furthermore, the Lagrangian virial stress is proposed, which is still in virial form but does not include velocities to avoid controversial velocity treatments. It is also found that the widely used classical virial stress is actually the Eulerian virial stress, which includes the velocities of atoms, and is valid only when the impulse-momentum theorem is applicable to estimate the internal forces. However this requirement for the Eulerian atomic stress can not always be met in practical cases, such as the material volume element in rotation and the examples presented in this paper, but the proposed Lagrangian atomic stress can avoid these velocity-related nonobjectivities.
In our subsequent paper, several MD examples are used to validate various existing stress definitions. The instantaneous Lagrangian atomic stress definition is also proposed for dynamical problem.
| Attachment | Size |
|---|---|
| 459.37 KB | |
| 406.05 KB |
- Bin Liu's blog
- Log in or register to post comments
- 12718 reads


Comments
Is Zhou really 'incorrect'?
It's not clear how you can say Zhou's expression is actually incorrect. It obviously depends on what you want to measure. Instantaneously, the stress in a body in 'equilibrium' need not be zero. Otherwise, what would be the mechanism of phase change, or residual stress? Your argument showing the incorrectness of Zhou's expression seems fundamentally flawed. You assume that the chain of atoms is in mechanical equilibrium but it clearly is not. It's like saying a perfectly elastic rod floating in space cannot have any stress in it. Surely there could be residual stress or waves propagating.
Ericmock,Thanks for your
Ericmock,
Thanks for your interest in our work. You are right that “the instantaneous stress in a body in 'equilibrium' need not be zero”. We’d like also point out that the absolute equilibrium can only be realized at 0K temperature, since the ordinary thermal movements of atoms imply the inequlibrium of the system. However, the example of atom chains used in our paper is actually assumed to be in statistical equilibrium or macroscopic equilibrium, which means the mechanical and thermal states would not evolve statistically. On the other hand, the Cauchy stress is the macroscopically meaningful stress definition, should be the average of the internal forces among atoms. Our point is that if this average is carried out in the Lagrangian way, no velocity appears in the definition. Virial stress corresponds to performing this average in Eulerian way, therefore, the velocity appears. But in Zhou’s work, neither these two frameworks is used completely in the averaging process to compute the stress, the kinetic part is then missed. The incorrectness of Zhou’s work has been numerically demonstrated by Subramaniyan (2007, see http://imechanica.org/node/871 and http://imechanica.org/node/3060). Another factor discussed in our paper is that the velocity is not an objective quantity, which depends on the choice of the reference frame. We therefore suggest adopting the Lagrangian atomic stress definition to avoid these probable velocity-related nonobjectivities.
In addition, we didn’t claim that “a perfectly elastic rod floating in space cannot have any stress in it”. If one computes the atomic stress locally, the stress due to the residual stress or waves propagating should not be zero. The spatial dimension to perform averaging internal forces depends on the specific problem: usually the smaller-sized averaging sample would lead to higher error, while the larger-sized sample may lose the resolution.
Our paper has been published
Our paper has been published in Journal of computational and theoretical nanoscience. 2009, vol.6(5):1081-1089. In the final edition (http://arxiv.org/ftp/arxiv/papers/0810/0810.0803.pdf), more discussions and examples are added. The most interesting one is as follows.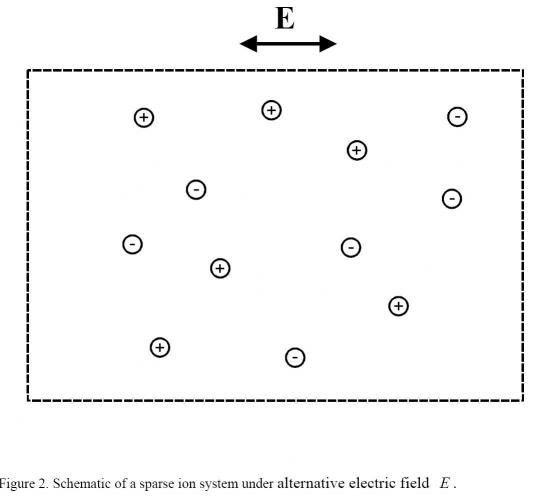
The figure shows a box consisting of some charged atoms or ions, and the distances among them are very large such that there is no interatomic interaction. If an alternative electric field E is applied to this system and the amplitude and frequency of E can be adjusted, one can imagine these charged atoms vibrate locally and the magnitude of the velocities can be very large. Therefore, the kinetic part of virial stress has negative components and the potential part is vanishing such that the virial stress also has negative components. By contrast, since the temporal average external force on any material point is zero and the system is obviously in thermodynamic equilibrium, the temporal average Cauchy stress should be zero according to the balance of forces. However, it is easy to note that the temporal average classical virial stress is not vanishing at all. This obvious inconsistence between Cauchy stress and the classical virial stress further indicates that the classical virial stress definition is not widely applicable due to the involvement of the velocity which relies on too many factors.