You are here
Journal Club Theme of August 2015: Nonlocality and yield in granular materials
Introduction:
We commonly encounter granular materials in our everyday lives — from our breakfast cereal to sand on the beach. Granular materials also play an integral role in a wide variety of industries — geotechnical, pharmaceutical, food processing, and energy, among many others — so predictive models for the mechanics of these materials are crucial. An important feature of the mechanics of granular materials is a yield condition, which differentiates between flowing and non-flowing states. In homogeneous simple shearing under the action of a shear stress, τ, and pressure, P, shown schematically below, a distinct transition between strictly elastic and flowing states is observed for a critical value of the ratio μ=τ/P, which we will refer to as μs.

Figure 1: Schematic of simple shear with pressure.
This transition may then be mathematically generalized to multiaxial states of stress through criteria such as the Mohr-Coulomb or Drucker-Prager conditions. These yield criteria are local in the sense that yield at a point is assessed only based on the stress and state variables at that point. Yield conditions of this type are widely used in engineering design involving granular materials; however, recent work in the granular physics community has uncovered nonlocal effects, arising due to cooperative interactions between grains, which lead to phenomena that cannot be captured with local yield conditions. In this journal club entry, we highlight two such phenomena: (1) secondary rheology, in which flow in one point of a granular medium erases the yield condition everywhere, and (2) the "smaller-is-stronger" size effect, in which thin granular layers must be tilted to a greater angles than thicker layers in order to flow. We will also discuss our nonlocal modeling approach, which is capable of accounting for both of these phenomenologies while still reducing to the correct local flow behavior in homogeneous flow.
Secondary rheology:
When a granular medium is subjected to inhomogeneous, boundary-driven deformation, steady flow concentrates into spatial regions, which we refer to as shear bands. To be clear, these are not shear bands induced by material instability but shear bands arising due to inhomogeneous stress fields. The sizes of these shear bands then depend both upon the geometry and the grain size. Until recently, conventional wisdom was that outside of these shear bands, the nominally quiescent material behaved statically, since the stress in regions outside of the shear bands is below the yield condition. However, recent experiments of van Hecke and coworkers [1] — an example of which is shown below — established that this is not the case. When a spherical steel intruder is placed on top of a large, static granular bed, the stress field induced by the sphere's weight is not sufficient to drive steady flow. The sphere sits statically on top of the granular bed, and the yield condition is obeyed. However, when flow is driven far away from the intruder (in this case by rotating a disk on the floor of the flow cell), the sphere immediately begins to sink into the granular media. The far-away flow only has a minimal influence on the stress field in the region of the sphere — not enough to cause the local yield condition to be reached. Hence far-away flow erases the yield condition everywhere.
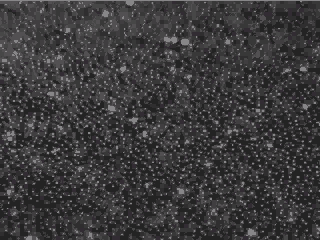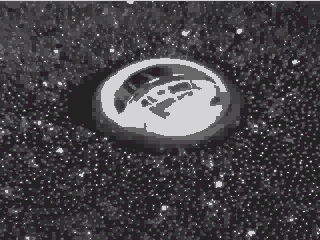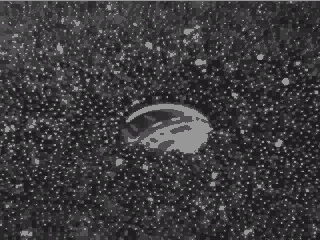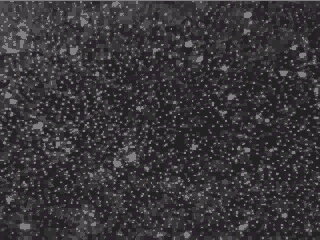

Figure 2: Left: Demonstration of secondary rheology using a steel sphere on top of a granular bed from the work of [1] (image courtesy of the website of M. van Hecke). Right: Schematic of the boundary motion used to induce the primary flow.
This phenomenology is quite general, seen in different flow configurations with different intruder geometries [2,3]. In particular, the work of Reddy et al. [2] utilizes a cylindrical intruder in an annular shear cell, and the work of Wandersman et al. [3] uses a vane-like intruder in a split bottom cell. In general, when an intruder is placed far away from a shear band — or primary flow zone — it will move when any non-zero force is applied to it. In contrast, when there is no primary flow, a distinct yield condition is observed, i.e., a critical applied intruder force must be exceeded to move it. This vanishing of the yield stress in the presence of far-away primary flow is quite remarkable and motivates the notion of a "secondary rheology" to describe the rheological changes occurring at different locations in a granular medium due to the existence of a primary flow. Importantly, several broad experimental observations have been made about the creep phenomenology: (1) For sufficiently low primary flow rates, secondary rheology is a rate-independent process, i.e., the intruder creep speed is linearly proportional to the primary flow rate. (2) The intruder creep speed is not necessarily linearly related to the force applied to the intruder. In fact, this relation is typically exponential in character. (3) The intruder creeps faster when it is placed closer to the primary flow zone.
Size-dependent yielding:
While secondary rheology encompasses the effect of far-away flow on yield at a point, a distinct nonlocal effect is the size-dependence of yield of a dense granular body. Size-dependence of yield is observed in many classes of materials, such as in the increased strength of micropillars in compression. An analogous size-effect is observed in granular materials — thoroughly characterized in the flow of thin granular layers down a rough inclined surface, shown schematically below. In the inclined plane geometry, a layer of thickness H is tilted to an angle θ. Under the action of gravity, the ratio of the shear stress to the pressure, μ, turns out to be spatially constant in the layer and given by tanθ. Hence, any local yield condition, such as Mohr-Coulomb or Drucker-Prager, would predict a universal angle of repose, i.e., any layer, regardless of thickness H, tilted above this critical angle would be predicted to flow. This point is directly contradicted by experiments. As shown initially by Pouliquen [4] and verified by others [5-7], the angle at which an initially flowing layer of grains comes to a stop, θstop, depends sensitively on the thickness of the layer when H is small. In these flows, thin layers cease flowing at a greater angle than their thicker counterparts. In fact, sufficiently thin layers will not flow for a range of tilt angles in which μ>μs — an effect not accounted for by local yield conditions. Inverting θstop(H) (as is convention in the granular physics community), one can extract a function Hstop(θ) for every granular system, which represents the critical thickness at which a flowing layer at a certain angle would arrest. The experimentally-measured Hstop(θ) curve for glass beads on a rough incline is shown below as data points (data from [4]). Hence, the maxim "smaller-is-stronger" holds true for granular materials. One expects the size-dependence of yield observed in inclined plane flow to be geometrically-general; however, this effect yet to be experimentally characterized in other flow configurations.
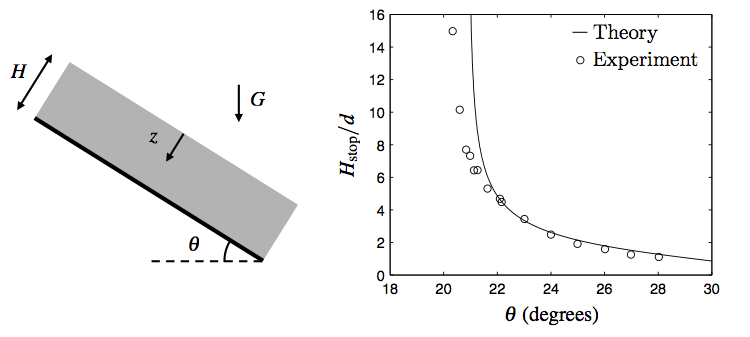
Figure 3: Left: Schematic of incline plane flow. Right: Hstop(θ) curve for glass beads on a rough surface.
Nonlocal modeling:
Local continuum models for granular materials are not equipped to predict secondary rheology or "smaller-is-stronger" size-effects. Recently, my work with Ken Kamrin of MIT has focused on developing a new continuum model — the nonlocal granular fluidity (NGF) model — geared towards predicting steady granular flow fields, including grain-size-dependent shear-band widths in a variety of flow configurations. See our papers [8,9] and Ken's prior journal club entry for an introduction to the NGF model and its application. Recently, we have shown that our nonlocal modeling approach is also capable of quantitatively capturing all aspects of secondary rheology [10] as well as describing the size-dependent strengthening of thin granular layers [11].
The NGF model's ability to describe all salient aspects of secondary rheology is summarized below. All points in the figure indicate the results of finite-element calculations using the NGF model. We consider 2D annular shear flow, shown schematically in the upper left, with a circular intruder. When there is no inner wall rotation, i.e., no primary flow, the force/velocity relation of the intruder displays a critical force, Fc, which must be applied to the intruder to move it, cf., upper right. However, when the inner wall is prescribed to move at a fixed speed, the intruder moves for all applied forces, including those that are subcritical. For instance, for the case of F/Fc=0.75, the intruder creep speed is shown in the lower left figure as a function of the wall speed. For sufficiently low wall speeds, the relationship is linear, indicating that secondary rheology is rate-independent. Finally, the lower right figure shows the intruder creep speed as a function of the force applied to the intruder. The relationship is exponential in character — the dashed line indicates an exponential function — consistent with experimental observations. For further details of these calculations, see our recent paper [10].

Figure 4: Upper left: Schematic of 2D annular shear with an intruder. Upper right: Force/velocity relation for the intruder in the absence of primary flow. Lower left: Intruder creep speed as a function of wall speed for a subcritical intruder force, demonstrating rate-independence. Lower right: Intruder creep speed as a function of intruder force, demonstrating an exponential relation. (Dashed lines are guides for the eye.)
This same continuum model is also capable of capturing the distinct phenomenon of size-dependent yielding [11]. The NGF model's prediction of the Hstop curve is shown above in Fig. 3, using the previously determined material parameters for glass beads, and the quantitative agreement is excellent. It is worth pointing out that the model's prediction was obtained using the same continuum parameters that were used to successfully predict steady flow fields of glass beads in split-bottom cells and other geometries [8]. Yet here, the question is of a different nature, one of predicting input conditions for flow stoppage rather than velocity profiles in a flowing body.
Outlook:
The NGF model's ability to quantitatively predict flow fields, capture all aspects of secondary rheology, and describe the size-dependence of flow down inclined planes speaks to its generality, and ongoing work seeks to apply the NGF model to new granular flow problems. One particularly important industrial question that could be addressed by the NGF model is that of size-sensitive flow stoppage phenomena, such as silo jamming. The famous Beverloo correlation, an empirical functional form that gives silo flow rate in terms of aperture opening size, indicates a critical opening size at which flow stops. The ability to predict such relations rather than empirically measuring them would be a boon to design in many industries. Finally, there remain several avenues for further theoretical development of the NGF model, see Ken's entry and our papers [8-11] for further discussion of these issues.
References:
[4] O. Pouliquen. Scaling laws in granular flows down rough inclined planes. Phys. Fluids, 11:542, 1999.
[7] G.D.R. MiDi. On dense granular flows. Euro. Phys. Journ. E, 14:341-365, 2004.
| Attachment | Size |
|---|---|
| 7.02 KB | |
| 139.25 KB | |
| 36.79 KB | |
| 1.72 MB | |
| 19.92 KB |
- David Henann's blog
- Log in or register to post comments
- 16871 reads


Comments
Very interesting!
Thanks David for the stimulating post! I think that nonlocal effects in granular flow may be something of far reaching implications. A phenomenon that is currently not very well understood is earthquake triggering in which an earthquale happeining on one fault may trigger an earthquake on another fault. While the direct explanation is that stress perturbations carried by the waves from one fault are sufficient to bring the other fault to a critical state,it is plausible that the granular rheology also varies under dynamic perturbation and this may enable triggering at lower stress. We started exploring the effects of vibrations, for example, on "fluidizing" dense granular systems within the shear transformation zone STZ theroy (with Charles Lieou, Jean Carlson and James Langer from UCSB) but I find your NGF another plausible route. I even suspect that the granular fluidity g may be closely related to the concept of effective temperature in STZ theory.
On the size effect issue, I think this is a fascinating topic. I wonder if this could be related to dynamic force chain buckling (which may be a generalization of a model similar to the outlined here: http://rsta.royalsocietypublishing.org/content/368/1910/249). One interesting application for this is to predict variations in sliding friction between two surfaces as a gouge layer starts to develop and increase in thickness due to wear. Experiments do suggest that coeeficient of friction decreases as gouge develops and then saturates (similar to your prediction of h-stop curve).
In any case, there are several interesting open questions in that area. So thank you for bringing it up in a vivid way. Looking forward to further interactions.
Re: Very interesting!
Hi Ahmed,
Thanks for your comment and your interest in the work. I think it's fair to say that nonlocal effects arising due to cooperativity are a crucial aspect of many systems, ranging from the simple monodisperse system of dry, spherical glass beads that I highlighted in the post to faults during earthquakes. Since you mentioned the effect of vibrations, I'll also point out the experimental work done in the van Hecke group on granular flow in the presence of externally applied vibrations, which shows that superposed vibrations have a profound effect on rheology, especially in the slow-flow regime:
J.A. Dijksman, G.H. Wortel, L.T.H. van Dellen, O. Dauchot, and M. van Hecke. Jamming, yielding, and rheology of weakly vibrated granular media. Phys. Rev. Lett. 107:108303, 2011.
G.H. Wortel, J.A. Dijksman, and M. van Hecke. Rheology of weakly vibrated granular media. Phys. Rev. E 89:012202, 2014.
To your point about the effective temperature of STZ theory, it is plausible that the granular fluidity may be related to the effective temperature. One major open question regarding the NGF model is precisely what does the fluidity represent, i.e., how can it be measured from microscopic quantities. Our ongoing work indicates that it can be related to microscopic fluctuations. Similarly, the effective temperature — which is defined thermodynamically through an entropy derivative — is also related to microscopic stress fluctuations (cf., I.K. Ono, C.S. O'Hern, D.J. Durian, S.A. Langer, A.J. Liu, and S.R. Nagel. Effective temperatures of a driven system near jamming. Phys. Rev. Lett. 89:095703, 2002). It would not be surprising if a relation between granular fluidity and effective temperature — or for that matter the granular temperature of kinetic theory — may be found.
Finally, the notion of force chain buckling as the mechanism of size-dependent strengthening is interesting. In a sense, it is built into the NGF model through the dependence of the cooperativity length, ξ, on the stress ratio, μ — the idea being that force chains have different properties depending on μ. One important benefit of the NGF model is that it can model both secondary rheology and the H-stop effect — which are seemingly disparate effects — in the same theoretical framework. This suggests that these phenomena (among others) arise due to the same underlying microscopic physics. While the model for force chain buckling can describe size-dependent strengthening, it isn't clear whether it can say anything about a phenomena like secondary rheology.
-David
moisture content
David, thanks for the fantastic reviews on the nonlocaility and yielding in granular flow. I am actually wondering if you and Ken are planning to incorporate the effect of moisture content and liquid bridges in your model? What is the potential challenge to do that?
Another question is related to the transition from granular flow to granular solid. In geotechnical engineering and geomechanics research, there is a significant body of work on modeling granular solid under confining pressure in which case the stress depends on loading history and strain instead of strain rate and we considered a material in critical state under a certain combination of shear stress, volumetric strain and mean pressure. However, for granular flow, strain rate and normal pressure seem to be dominating factors for the shear stress. Is there any work aimed to provide a unified framework to allow a smooth transition from fluid-like state to solid-like state and vice versa? What is the major difficulty to model such transitions? What are the major difference in those two transitions? The (re-)activiation of the fault guoge layer mentioned by Ahmed seems to be more related to a transition from solid-like status to the fluid-like states, and a few works are attempted to model that for dense assemblies, e.g. [1]. However, I am interested to see whether it is possible to model such transition back and forward. Any guidance is greatly appreciated.
[1] Andrade, J. E., Chen, Q., Le, P. H., Avila, C. F., & Evans, T. M. (2012). On the rheology of dilative granular media: Bridging solid-and fluid-like behavior. Journal of the Mechanics and Physics of Solids, 60(6), 1122-1136.
Re: moisture content
Hi Steve,
Thanks for your comment. You’ve touched on two important issues to which we’ve given a lot of thought and that we do plan on incorporating into the NGF modeling framework. To your first point, we’ve only considered dry granular materials so far, and the situation changes when the material is partially saturated with a pore fluid, leading to cohesion due to the liquid bridges between particles. Mathematically, the NGF model revolves around the granular fluidity − a scalar state parameter which relates the stress quantity driving flow to the consequent strain rate. For cohesionless, dry granular materials, this stress quantity is μ=τ/P, a stress invariant. (Note that one would expect the same approach to work for the fully saturated case, since it is cohesionless as well. Hence, the path forward for fully saturated granular materials is more straightforward in my opinion.) Clearly, a modified stress quantity, which accounts for cohesion, must be identified for the partially saturated case. As a first cut, one might incorporate the capillary pressure arising due to the liquid bridges into the definition of μ. The simplest definition is μ=τ/(P + P_cap), where τ and P are the stress invariants as before and P_cap is the capillary pressure due to liquid bridges, which is a function of the degree of saturation. (One may think of P_cap as the maximum hydrostatic tension that a partially saturated granular material may sustain before coming apart. Hence it should be zero for both the unsaturated and fully saturated cases but greater than zero in between.) The idea is that the normal force felt at granular contacts is a combination of both the confining loads which give rise to the pressure P and the capillary effects which give rise to P_cap. Of course this is just a first hypothesis and will need to be tested and refined by experiments.
In your second point, you ask if there are models that are aimed at the transition between solid-like and fluid-like behavior. I’d like to think that the NGF model provides such a unified framework. In fact, I would argue that the phenomenologies discussed in my post are both more solid-like than fluid-like, involving a yield condition and rate-independent behavior (as demonstrated in the lower left figure of Fig.4). The NGF model unifies this rate-independent, solid-like regime of deformation with the fluid-like regime (in which the μ(I) rheology provides an excellent description) and does so in a manner which is quantitatively consistent with experiments.
However, with all of this said, the solid-like aspect of the NGF model is not as detailed as most granular plasticity models like critical state theory. In particular, we have ignored the effects of loading and preparation history as well as shear-induced dilatancy thus far, instead focusing only on the “critical state” so as to quantitatively test the nonlocal aspect of the model. One strategy for remedying this would be to incorporate the effect of loading history into the local response, i.e., through the function g_loc. In the current model, g_loc only depends upon stress, but in a history-dependent version, it could depend on strain as well (as in the paper you cited) or some other system of internal variables (for example, as in Anand and Gu). The resulting model would be capable of describing (i) nonlocal effects, (ii) transient shear weakening/strengthening and the effect of the initial state, and (iii) the smooth transition to the rate-dependent regime as strain-rate is increased.
Finally, since you mentioned modeling the process of repeated yielding, I'll raise one additional complicating wrinkle: Yield in granular materials is hysteretic. For example, in the inclined plane geometry discussed in my post, one will robustly measure an Hstart curved based upon the initiation of flow, which is distinct from the Hstop curve based upon flow cessation (see MiDi for more). Hysteretic yield has been attributed to the local μ(I) rheology actually being non-monotonic, i.e., not invertible (see Dijksman et al.). Incorporating hysteretic yield into a continuum model is a significant challenge going forward.
-David
Yield strength
Dear Henann,
The area of granular materials is very interesting. I have encoutered the sand case yhat you have cited which is a granular media but my application is a component of concrete (sands, cement and gravels) for which the compressive strength is less or equal than 40 MPa (www.infociments.fr). The tensile one is less or equal than 4 MPa for the 28 days cement. And the yield strength of the steel is about 270 MPa (AFNOR). So i want to ask you a question : what are the methods to obtain the yield strength of the mixture Reinforced-Concrete (steel+concrete).