You are here
Journal Club for August 2019: Instabilities in Soft Composites
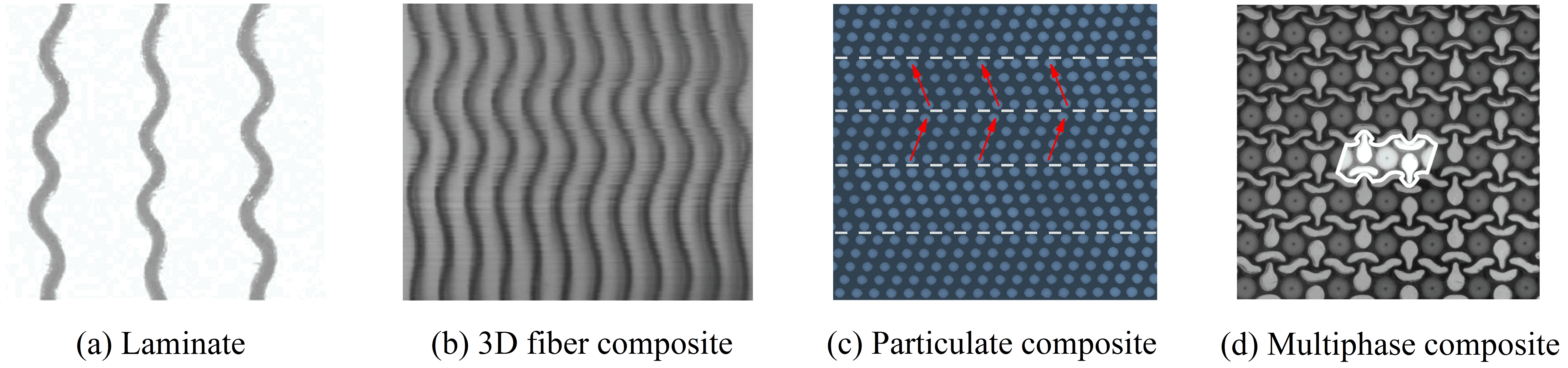
Elastic instability is a fascinating phenomenon playing an important role in pattern formations in soft biological systems. The phenomenon also has been actively used by the (i-)mechanics and physics community to design new (meta-) materials with switchable microstructures, properties, and functions [1].
This month J-Club is intended to initiate and encourage discussion of the instability phenomena in soft materials. The post focuses on the soft heterogeneous materials, typically combining soft deformable matrix and stiffer phase (such as fibers or inclusions). Here, we only provide just a few illustrative examples from the large body of relevant works on this topic. Figure 1 shows the experimentally observed wavy patterns forming in (a) soft 3D printed laminates [2] and (b) 3D-fiber composites [3], (c) twining pattern in particulate composites [4], and (d) auxetic microstructure transformations in inclusion-matrix-void soft systems [5].
On the analytical side, the superimposed “small-on-large” Bloch-Floquet analysis is commonly widely used now for instability analysis of soft periodic materials. The development and implementation of the method go back to the important series of works from the 1980s by Triantafyllidis and co-authors [6]. One should note the remarkable contribution of the paper by Geymonat et al. [7], showing the equivalence of long-wave limit by Bloch-Floquet instability analysis to the loss of ellipticity analysis. The latter is an effective method for detecting macroscopic or long-wave instabilities. Here, we follow the analysis to investigate the instabilities in microstructured hyperelastic composites and realize these patterns in experiments.
Periodic laminates. It is well known that the stiffer layers undergo buckling when the composite is compressed beyond the critical level [8]. The larger layer stiffness contrast, and concentration of the stiffer phase, the larger the critical wavelength is. This allows to regulate and pre-designed the switchable microstructure in soft laminates. Although we limit this discussion to elastic (or hyperelastic) behavior mostly neglecting inelastic aspects, these can be used to increase the pool of admissible microstructures upon instability. Thus, for example, identical samples can develop different patterns as a result of different loading histories. This has been experimentally illustrated on 3D-printed laminates loaded at different strain-rates (as shown in Fig. 2) [2].

Fig. 2. Formation of wavy interfaces in soft layered composites under different strain rates [2].
3D fiber composites. Similarly to laminates, 3D fiber composites are undergo buckling when compressed (see Fig. 1(b)), the 3D fiber composites, however, are more stable and require higher strains to trigger buckling [9]. In periodically distributed fiber composites with square in-plane periodicity, we observe the transition of the instability induced patterns from small wavelength wavy pattern to long-wave mode with an increase in fiber volume fraction. Interestingly, composites with rectangular fiber (in-plane) periodicity exhibit cooperative buckling mode developing in the direction, where the fibers are closer to each other (as shown in Fig. 3) [3].

Fig. 3. (a) Development of wavy patterns in 3D-fiber composites with rectangular in-plane periodicity; (b) Dependence of critical wavenumber on the periodicity aspect ratio [3].
Particulate Composites. In particle composites with stiff inclusions periodically distributed in a soft elastomeric matrix, we observe the domain formations and pattern transitions in 3D-printed samples subjected to large deformations (as shown in Fig. 4). Interestingly, the domain patterns are observed in the composites for which macroscopic instabilities are predicted by the Bloch-Floquet analysis. These fully determined new patterns can be achieved by fine-tuning of the initial microstructure [4].
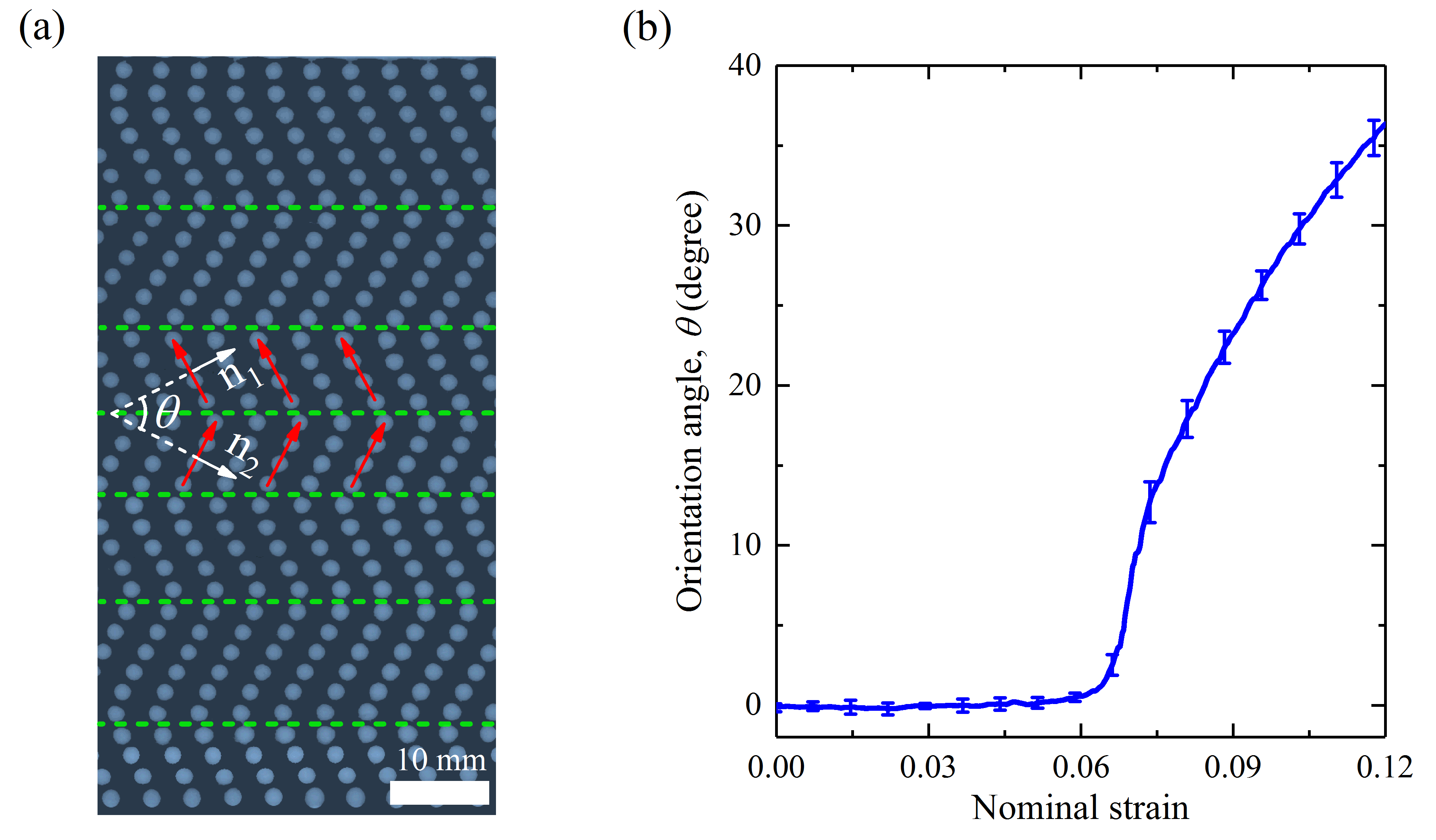
Fig. 4 (a) Instability-induced domain formations in the particulate composite; (b) Dependence of domain orientation angle on the applied strain [4].
Inclusion-Matrix-Void Composite. Finally, we illustrate the concept of multiphase auxetic composites consisting of stiff inclusions and voids periodically distributed in a soft matrix. Upon instability, the composite microstructure rearranges into new morphologies [5], (see Fig. 5). These pattern transformations are accompanied by the void collapse and lead to negative Poison’s ratio (NPR) or auxetic behavior. This mechanism is similar to the one observed in the periodic matrix-void system by Mullin et al. [10]; it is worth mentioning that this buckling mode was numerically predicted by Triantafyllidis et al. [11] in the two-phase system with compressible inclusions. In the 3-phase composite, the stiff inclusion phase plays an important role in regulating the onset of instabilities and the post-buckling microstructure transformations. Thus, for example, the NPR behavior can be pre-designed to develop at small strains, and distinct post-buckling patterns can be achieved through the positioning of stiff inclusions [12].
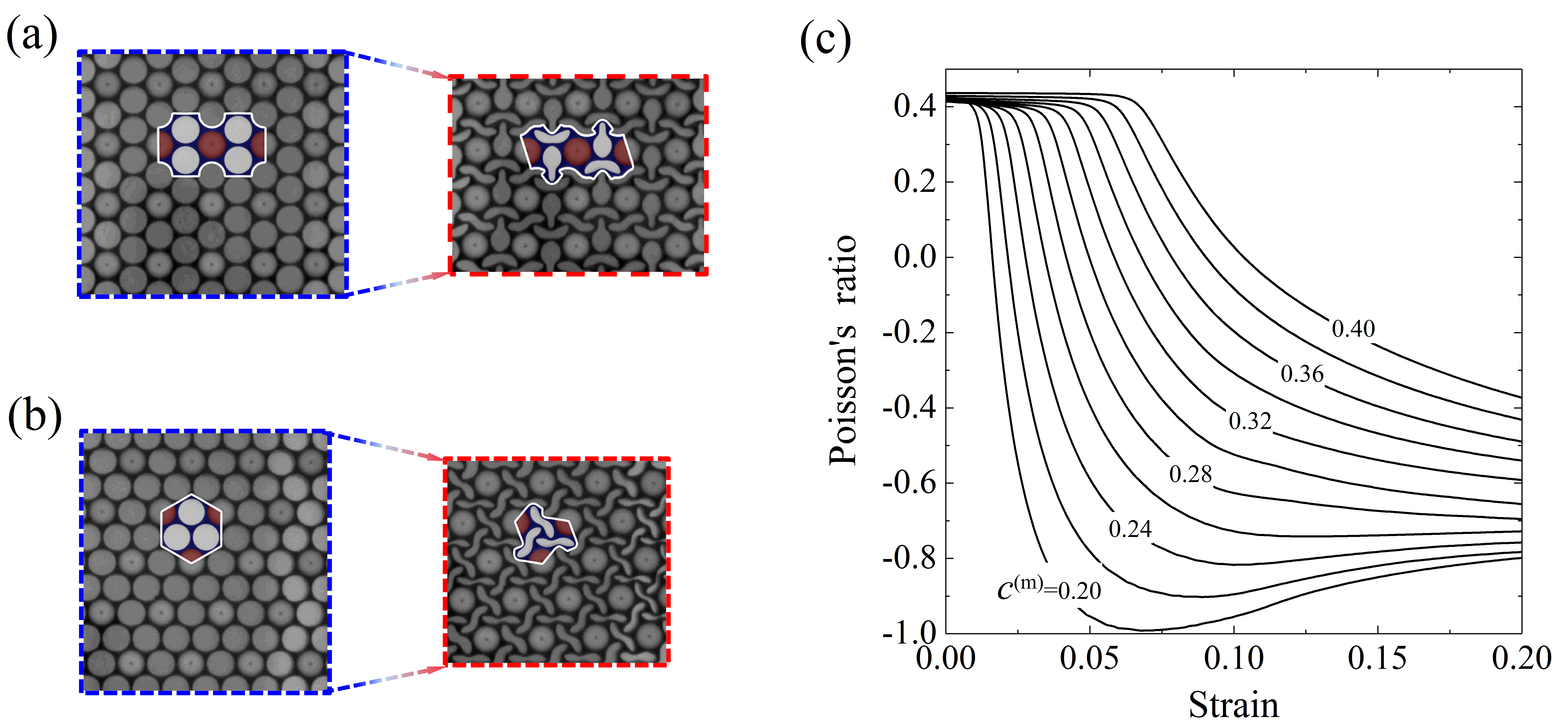
Fig. 5 Distinct pattern formations in multiphase composites in the square (a) and triangular (b) arrangement of inclusions; (c) Dependence of Poisson’s ratio on applied deformation for composites with various matrix volume fractions [12].
A video illustration can be found at https://www.youtube.com/watch?v=RRlJhnIylNk
These are just a few examples of instabilities, and we hope that this short post will initiate discussion of the very rich topic.
References
[1] Kochmann DM, Bertoldi K. Exploiting Microstructural Instabilities in Solids and Structures: From Metamaterials to Structural Transitions. Appl Mech Rev 2017;69:050801.
[2] Slesarenko V, Rudykh S. Harnessing viscoelasticity and instabilities for tuning wavy patterns in soft layered composites. Soft Matter 2016;12:3677–82.
[3] Li J, Slesarenko V, Galich PI, Rudykh S. Instabilities and pattern formations in 3D-printed deformable fiber composites. Compos Part B Eng 2018;148:114–22.
[4] Li J, Pallicity TD, Slesarenko V, Goshkoderia A, Rudykh S. Domain Formations and Pattern Transitions via Instabilities in Soft Heterogeneous Materials. Adv Mater 2019;31:1807309.
[5] Li J, Slesarenko V, Rudykh S. Auxetic multiphase soft composite material design through instabilities with application for acoustic metamaterials. Soft Matter 2018;14:6171–80.
[6] Triantafyllidis N, Maker BN. On the Comparison Between Microscopic and Macroscopic Instability Mechanisms in a Class of Fiber-Reinforced Composites. J Appl Mech 1985;52:794.
[7] Geymonat G, Müller S, Triantafyllidis N. Homogenization of nonlinearly elastic materials, microscopic bifurcation and macroscopic loss of rank-one convexity. Arch Ration Mech Anal 1993;122:231–90.
[8] Li Y, Kaynia N, Rudykh S, Boyce MC. Wrinkling of interfacial layers in stratified composites. Adv Eng Mater 2013;15:921–6.
[9] Slesarenko V, Rudykh S. Microscopic and macroscopic instabilities in hyperelastic fiber composites. J Mech Phys Solids 2017;99:471–82.
[10] Mullin T, Deschanel S, Bertoldi K, Boyce MC. Pattern Transformation Triggered by Deformation. Phys Rev Lett 2007;99:084301.
[11] Triantafyllidis N, Nestorović MD, Schraad MW. Failure Surfaces for Finitely Strained Two-Phase Periodic Solids Under General In-Plane Loading. J Appl Mech 2006;73:505–15.
[12] Li J, Rudykh S. Tunable microstructure transformations and auxetic behavior in 3D-printed multiphase composites: The role of inclusion distribution. Compos Part B Eng 2019;172:352–62.
| Attachment | Size |
|---|---|
| 1.91 MB | |
| 602.25 KB | |
| 1.66 MB | |
| 297.17 KB | |
| 1.31 MB | |
| 1.3 MB |
- Stephan Rudykh's blog
- Log in or register to post comments
- 4332 reads


Recent comments