Journal Club for October 2022: A Mechanical Approach to Shape, Flow, and Mechanoperception in Plants

 

Jean-François Louf

Department of Chemical Engineering, Auburn University

 

Introduction

Plants are multicellular organisms essential to our ecosystem in many ways: they can transform sunlight into biomaterial providing a food source for animals, produce oxygen through photosynthesis, a crucial chemical for life, and also provide chemicals for drugs used in medicine to heal patients. As a result, plants have been studied extensively by biologist, bringing a fundamental understanding of the biological machinery at play in many aspects of plant life, from reproduction to photosynthesis, sugar production, and more. However, while intrinsically biological in nature, plants cannot violate the laws of physics, which dictate fundamental principles such as their forms & size, flow control abilities, or even responses to mechanical stimuli.

From an engineering point of view, plants can be seen as an elastic medium full of water. As a result, deformations of the soft matrix surrounding the liquid will induce an overpressure locally and generate water motion, which will in turn modify the elastic matrix (Figure 1.a). Poroelasticity, the branch of mechanics that describes the interactions between a fluid contained in a porous media and the surrounding material, is therefore a natural framework to investigate plant internal responses to environmental stimuli. Interestingly, these environmental stimuli can come from other living creatures but also from the fluids wherein plants live, namely air or water, and generate stresses leading to different growth responses. 

In this discussion, we will examine plants through the eyes of an engineer and discuss the fluid and elasticity couplings responsible for plant shapes, flow motion in their vasculature, and their ability to sense.

 

1- Plant shapes

In contrast with animals, where size reaches a maximum at adulthood, trees never stop growing [1]. Yet, the highest tree in the world has a finite height of 115m. Why? What are the parameters setting this upper limit?

A possible mechanism controlling tree height is weight, and their subsequent sensitivity to buckling. Previous work modeled trees as cantilever beams that must prevent self-buckling, leading to a 2/3 power relationship between their maximum height Lc and their radius r, 
Lc= (C r2 E/γ)1/3 , with E as the Young’s modulus, γ the unit volume weight, and a parameter C [2]. This scaling law has been verified in wild trees [3] and commonly used in forest science and ecology [4]–[7]. More refined models were later on developed to investigate the self-buckling behavior of tapered rods, mimicking trees [8]–[10]. Very recently, scientists have looked at weight variation along the principal axes, to mimic tree morphology and investigate the effect of branches and leaves on the buckling behavior, and the subsequent effect on maximum tree height [11]. They found that branches and leaves were organized in a fashion that has little effects on the tree height [11], [12]. As a result, it appears that the buckling criterium for a tree is enough to explain the relationship between radius and height, but lack to impose a maximum finite tree height.

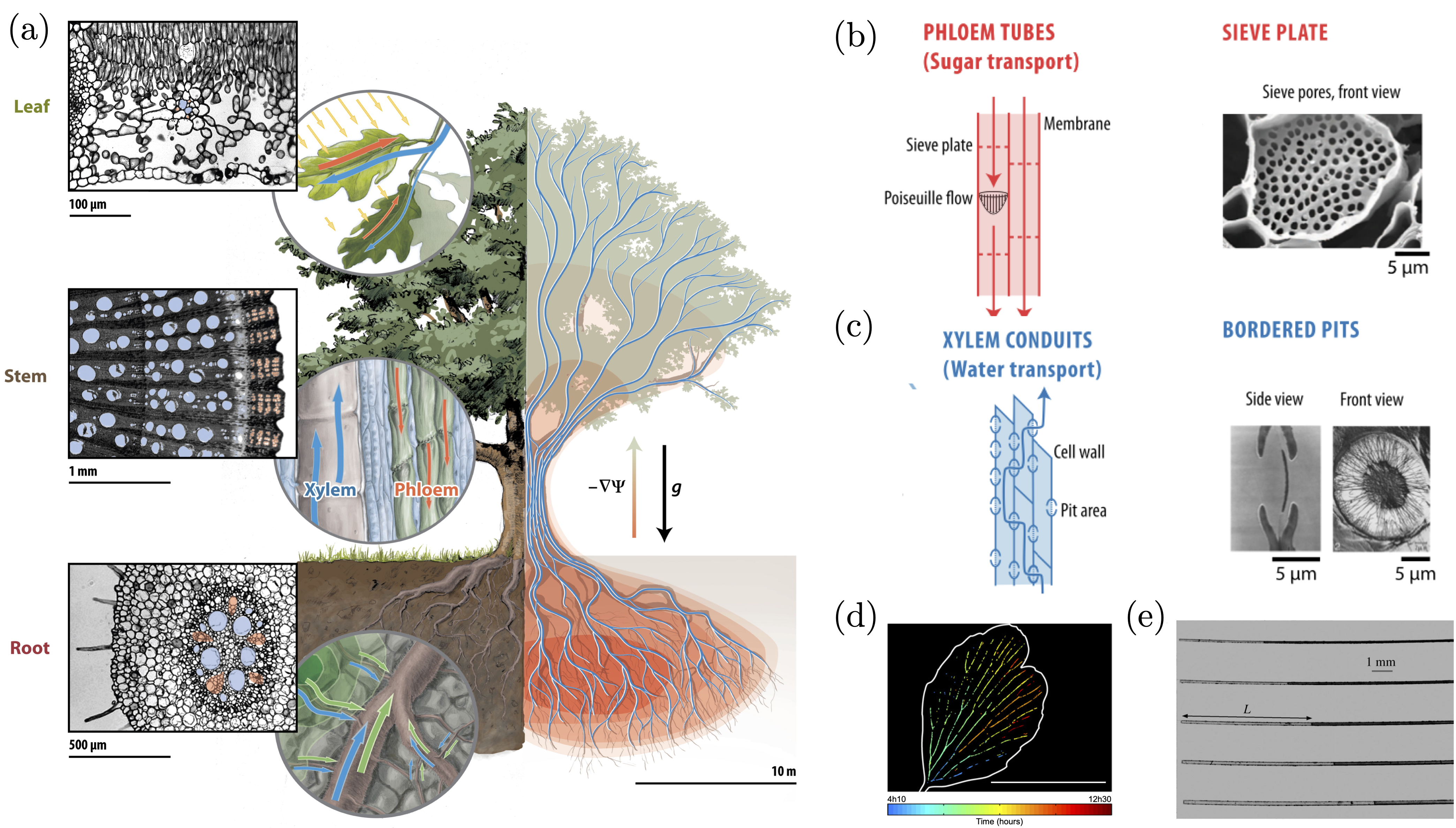
Leaves are the location where photosynthesis occurs, effectively feeding the plant. Interestingly, in a tall tree, one can observe that leaves shrink as we go up the tree [13]. This size variation may have repercussions on photosynthesis rate. Indeed, a key parameter regulating photosynthesis is stomata opening. Stomata are tiny holes in leaves, that can open or close in order to regulate the CO2 intake and concomitant water loss [14]. According to cohesion-tension theory [15], the opening of stomata induces a negative pressure gradient, that pulls water from the roots up to the leaves, through a network of tube-like dead cells called xylem [16] (Figure 1.a). Measurements conducted on several hundred terrestrial plant species suggest that stomatal conductance is limiting photosynthesis in tall trees due to a low water potential as a consequence of gravity and path length resistance [17], [18]. Together, these results suggest that hydraulics is a key factor limiting height growth [13], [17], [19], but a detailed mechanical model of stomatal conductance is still missing.

As we saw, leaf size can vary significantly within a single tree, but surprisingly their shape varies drastically among species. Such variations are the results of another key parameter controlling tree height: wind. Wind can affect the structural integrity of a tree, modifying its growth [20], but can also pull-out leaves and threaten the tree survival. As a result, leaves have evolved to have a wide variety of shapes with different mechanical properties. If we take a closer look at a leaf, and decompose it in two parts: the lamina, typically a 2D surface where photosynthesis mainly occurs, and the petiole, linking the lamina to the tree branch, we can then link the geometry of the former with the mechanical properties of the later [21], [22]. For example, a long narrow lamina is subjected to wind-induced bending, and its petiole has therefore to be able to withstand such stress by having a high flexural rigidity EI, with I the second moment of area. A contrario, a short wide lamina, will be subjected to twisting, resulting in a twisting stress at the base of the petiole, that must in turn have a high torsional rigidity GJ, with G the shear modulus of the petiole and J the polar moment of area. Interestingly, these modifications in rigidities are not due to alterations in material properties but results purely from geometrical configurations, illustrating the efficiency of biological designs. 

 

2- Elastic membranes in plants for flow control

Plants are extremely sophisticated pumps that can manipulate water in a wide variety of environments and states. This ability stems from the intricate multiscale nature of the channels that compose their vasculature. For example, phloem (Figure 1.a), the channel-like cells that circulate sugar from the leaves to the other plant organs, have circular poroelastic membranes called sieve plates that can bend when submitted to a pressure difference (Figure 1.b) [23], [24]. Upon bending, the micron size holes in the membrane will deform, affecting the flow non-linearly and thus providing a fine control of the flow rate [25]. This defensive mechanism is typically used against sap-feeding insects.

In the xylem of conifers, another type of membranes exists to regulate flow: the torus margo (Figure 1.c) [26]–[28]. In periods of high hydric stress, the water in the xylem can be under extreme tensions that can lead to cavitation events. The generation of a bubble could be fatal to a tree if it were going to spread throughout its vasculature. This is where the torus margo membrane comes into play. Located at the junction between two channels, called tracheid in conifers, the torus margo acts as a non-linear valve [29]. The pressure difference between the air phase and liquid phase generates an effective force deflecting the membrane and sealing the channel, thus preventing the spread of gas bubbles following cavitation, effectively isolating the channel full of air from the rest of the tree [29].

Despite the mechanisms developed by plants to prevent air spreading in their vasculature, a phenomenon referred to as emboli, these events are ubiquitous in a plant’s life. In leaves, the embolism bubble dynamics is driven by the evaporation of water through the air-water interface and through the lamina perforated by stomata. Such geometry has been recently investigated in vivo (Figure 1.d) [30], and in biomimetic leaves (Figure 1.e) [31]–[33], leading to the development of quantitative models that provide guiding lines to biologist to better understand the sensitivity of specific plant species to embolism. 

 

3- Plant mechanoperception

A fascinating feature of plants is their ability to feel despite their lack of a nervous system. By leveraging pressure, plants are able to feel contact locally and transport this information far away. Indeed, when a stem is touched, its soft tissues get compressed, leading to an increase of pressure of the liquid locally. Surprisingly, this phenomenon also occurs upon bending: when a branch is bent, the pressure of the fluid increases, despite linear beam theory predicting no volume change. Such response is highly nonlinear and can be explained by the theory of ovalization of tubes [34]: upon bending, it is energetically more favorable for a porous beam to gather its material closer to the neutral surface, generating a transverse strain that varies as the square of the bending strain [35] (Figure 2.a). The resulting overpressure then propagates within milliseconds as a pulse throughout the plant vasculature, until reaching mechanosensitive cells located at the top of the plant. These cells will swell, leading to the opening of ionic channels that will release calcium ions and other molecules responsible for growth response [36].  

Another interesting aspect of plants’ ability to feel is crown shyness. If we look at some forests, it is possible to observe fully stocked trees not touching each other (Figure 2.b). Naively, we can imagine that plants are able to feel other plants nearby and grow in order to not touch them, a very uncanny ability. While the physical mechanisms responsible for this phenomenon are still elusive [37], [38], a potential explanation involves mechanical abrasion [39]–[41]. Indeed, as wind blows between branches, they rub against each other, leading to a naturally occurring pruning effect, where young buds are removed from the branches. As a result, trees do not feel each other, but as a gardener, wind is responsible for their shapes. However, mysteriously, forest scientists observed a correlation between tree slenderness and crown shyness, an observation yet to be explained [42].

 

Summary and perspective

We briefly discussed the physical parameters controlling tree and leaf shapes, flow control abilities of plants, and plant mechanical responses to their surroundings.

The field of plant biomechanics is still young with a lot to investigate. As engineers, we can shed light on fundamental aspects of plant biology, simply by using elementary mechanical laws, but also learn from plants and mimic their abilities for human applications. In this discussion, we saw examples on how mechanics can be used in plant biology, at different scales, in both solid and fluid mechanics. In terms of biomimetic applications, plant-inspired designs can be extremely different, ranging from car designs [43] to morphing wings [44], passive actuators [45]–[47] to microrobotics [48], [49], and propulsion devices [50] to tactile sensors [50]–[52]. 

As a final note, I would like to quote Galileo: “the book of nature is written in the language of mathematics”, and add that us, engineers can read it; and if we understand it, use this knowledge to build something meaningful for society, echoing what Feynman said, “what I cannot create, I do not understand”.   

 

Citations 

[1]      J. McMurray, “Painting Trees in the Health Care Center,” Act Adapt Aging, vol. 14, no. 1–2, pp. 15–20, 1989.

[2]      A. G. Greenhill, Determination of the greatest height consistent with stability that a vertical pole or mast can be made, and of the greatest height to which a tree of given proportions can grow. 1881.

[3]      T. McMahon, “Size and shape in biology: elastic criteria impose limits on biological proportions, and consequently on metabolic rates,” Science (1979), vol. 179, no. 4079, pp. 1201–1204, 1973.

[4]      N. M. Holbrook and F. E. Putz, “Influence of neighbors on tree form: effects of lateral shade and prevention of sway on the allometry of Liquidambar styraciflua (sweet gum),” Am J Bot, vol. 76, no. 12, pp. 1740–1749, 1989.

[5]      M. Aiba and T. Nakashizuka, “Differences in the dry‐mass cost of sapling vertical growth among 56 woody species co‐occurring in a Bornean tropical rain forest,” Funct Ecol, vol. 21, no. 1, pp. 41–49, 2007.

[6]      M. Fournier, J. Dlouhà, G. Jaouen, and T. Almeras, “Integrative biomechanics for tree ecology: beyond wood density and strength,” J Exp Bot, vol. 64, no. 15, pp. 4793–4815, 2013.

[7]      C. Goudenhooft, T. Almeras, A. Bourmaud, and C. Baley, “The remarkable slenderness of flax plant and pertinent factors affecting its mechanical stability,” Biosyst Eng, vol. 178, pp. 1–8, 2019.

[8]      W. G. Smith, “Analytic solutions for tapered column buckling,” Comput Struct, vol. 28, no. 5, pp. 677–681, 1988.

[9]      D. J. Wei, S. X. Yan, Z. P. Zhang, and X.-F. Li, “Critical load for buckling of non-prismatic columns under self-weight and tip force,” Mech Res Commun, vol. 37, no. 6, pp. 554–558, 2010.

[10]    T. Kanahama, T. Fujimura, and M. Sato, “Critical height for self-weight buckling in tapered trees,” Journal of Japan Society of Civil Engineers, Ser. A2 (Applied Mechanics (AM)), vol. 77, no. 1, pp. 62–71, 2021.

[11]    T. Kanahama and M. Sato, “Mathematical modelling to determine the greatest height of trees,” Sci Rep, vol. 12, no. 1, pp. 1–15, 2022.

[12]    K. J. Niklas, “Interspecific allometries of critical buckling height and actual plant height,” Am J Bot, vol. 81, no. 10, pp. 1275–1279, 1994.

[13]    G. W. Koch, S. C. Sillett, G. M. Jennings, and S. D. Davis, “The limits to tree height,” Nature, vol. 428, no. 6985, pp. 851–854, 2004.

[14]    R. Hedrich and S. Shabala, “Stomata in a saline world,” Curr Opin Plant Biol, vol. 46, pp. 87–95, 2018.

[15]    M. T. Tyree, “The cohesion-tension theory of sap ascent: current controversies,” J Exp Bot, vol. 48, no. 10, pp. 1753–1765, 1997.

[16]    C. R. Brodersen, A. B. Roddy, J. W. Wason, and A. J. McElrone, “Functional status of xylem through time,” Annu Rev Plant Biol, vol. 70, no. 1, pp. 407–433, 2019.

[17]    B. J. Yoder, M. G. Ryan, R. H. Waring, A. W. Schoettle, and M. R. Kaufmann, “Evidence of reduced photosynthetic rates in old trees,” Forest Science, vol. 40, no. 3, pp. 513–527, 1994.

[18]    M. G. Ryan and B. J. Yoder, “Hydraulic limits to tree height and tree growth,” Bioscience, vol. 47, no. 4, pp. 235–242, 1997.

[19]    N. G. McDowell, N. Phillips, C. Lunch, B. J. Bond, and M. G. Ryan, “An investigation of hydraulic limitation and compensation in large, old Douglas-fir trees,” Tree Physiol, vol. 22, no. 11, pp. 763–774, 2002.

[20]    E. W. Holroyd III, “Prevailing winds on Whiteface Mountain as indicated by flag trees,” Forest Science, vol. 16, no. 2, pp. 222–229, 1970.

[21]    S. Vogel, “Twist-to-bend ratios and cross-sectional shapes of petioles and stems,” J Exp Bot, vol. 43, no. 11, pp. 1527–1532, 1992.

[22]    J.-F. Louf, L. Nelson, H. Kang, P. N. Song, T. Zehnbauer, and S. Jung, “How wind drives the correlation between leaf shape and mechanical properties,” Sci Rep, vol. 8, no. 1, pp. 1–7, 2018.

[23]    D. L. Mullendore, C. W. Windt, H. van As, and M. Knoblauch, “Sieve tube geometry in relation to phloem flow,” Plant Cell, vol. 22, no. 3, pp. 579–593, 2010.

[24]    K. H. Jensen, D. L. Mullendore, N. M. Holbrook, T. Bohr, M. Knoblauch, and H. Bruus, “Modeling the hydrodynamics of phloem sieve plates,” Front Plant Sci, vol. 3, p. 151, 2012.

[25]    J.-F. Louf, J. Knoblauch, and K. H. Jensen, “Bending and stretching of soft pores enable passive control of fluid flows,” Phys Rev Lett, vol. 125, no. 9, p. 098101, 2020.

[26]    J. Pittermann, J. S. Sperry, U. G. Hacke, J. K. Wheeler, and E. H. Sikkema, “Torus-margo pits help conifers compete with angiosperms,” Science (1979), vol. 310, no. 5756, p. 1924, 2005.

[27]    J. Pittermann, J. S. Sperry, U. G. Hacke, J. K. Wheeler, and E. H. Sikkema, “Inter‐tracheid pitting and the hydraulic efficiency of conifer wood: the role of tracheid allometry and cavitation protection,” Am J Bot, vol. 93, no. 9, pp. 1265–1273, 2006.

[28]    U. G. Hacke, J. S. Sperry, and J. Pittermann, “Analysis of circular bordered pit function II. Gymnosperm tracheids with torus‐margo pit membranes,” Am J Bot, vol. 91, no. 3, pp. 386–400, 2004.

[29]    P. J. Schulte and U. G. Hacke, “Solid mechanics of the torus–margo in conifer intertracheid bordered pits,” New Phytologist, vol. 229, no. 3, pp. 1431–1439, 2021.

[30]    T. J. Brodribb, D. Bienaimé, and P. Marmottant, “Revealing catastrophic failure of leaf networks under stress,” Proceedings of the National Academy of Sciences, vol. 113, no. 17, pp. 4865–4869, 2016.

[31]    B. Dollet, J.-F. Louf, M. Alonzo, K. H. Jensen, and P. Marmottant, “Drying of channels by evaporation through a permeable medium,” J R Soc Interface, vol. 16, no. 151, p. 20180690, 2019.

[32]    B. Dollet, K. N. C. Encarnación, R. Gautier, and P. Marmottant, “Drying by pervaporation in elementary channel networks,” J Fluid Mech, vol. 906, 2021.

[33]    K. N. Chagua Encarnación, P. Marmottant, and B. Dollet, “Pervaporation-induced drying in networks of channels of variable width,” Microfluid Nanofluidics, vol. 25, no. 9, pp. 1–10, 2021.

[34]    L. G. Brazier, “On the flexure of thin cylindrical shells and other" thin" sections,” Proceedings of the Royal society of London. Series A, containing papers of a mathematical and physical character, vol. 116, no. 773, pp. 104–114, 1927.

[35]    J.-F. Louf, G. Guéna, E. Badel, and Y. Forterre, “Universal poroelastic mechanism for hydraulic signals in biomimetic and natural branches,” Proceedings of the National Academy of Sciences, vol. 114, no. 42, pp. 11034–11039, 2017.

[36]    D. Tran et al., “Cellular transduction of mechanical oscillations in plants by the plasma-membrane mechanosensitive channel MSL10,” Proceedings of the National Academy of Sciences, vol. 118, no. 1, p. e1919402118, 2021.

[37]    A. J. Rebertus, “Crown shyness in a tropical cloud forest,” Biotropica (USA), 1988.

[38]    R. Hattimare, “Crown shyness in various tree species,” Int. J. Sci. Dev. Res, vol. 3, pp. 322–324, 2018.

[39]    M. Rudnicki, V. J. Lieffers, and U. Silins, “Stand structure governs the crown collisions of lodgepole pine,” Canadian Journal of Forest Research, vol. 33, no. 7, pp. 1238–1244, 2003.

[40]    P. Hajek, D. Seidel, and C. Leuschner, “Mechanical abrasion, and not competition for light, is the dominant canopy interaction in a temperate mixed forest,” For Ecol Manage, vol. 348, pp. 108–116, 2015.

[41]    A. J. Rebertus, “Crown shyness in a tropical cloud forest,” Biotropica (USA), 1988.

[42]    J. van der Zee, A. Lau, and A. Shenkin, “Understanding crown shyness from a 3-D perspective,” Ann Bot, vol. 128, no. 6, pp. 725–736, 2021.

[43]    M. Pagitz and J. Bold, “Shape-changing shell-like structures,” Bioinspir Biomim, vol. 8, no. 1, p. 016010, 2013.

[44]    R. Vos and R. M. Barrett, “Pressure adaptive honeycomb: A novel concept for morphing aircraft structures,” in Proceedings of the 27th Congress of the International Council of the Aeronautical Sciences, Nice, France, 2010, pp. 19–24.

[45]    D. Lunni, M. Cianchetti, C. Filippeschi, E. Sinibaldi, and B. Mazzolai, “Plant‐inspired soft bistable structures based on hygroscopic electrospun nanofibers,” Adv Mater Interfaces, vol. 7, no. 4, p. 1901310, 2020.

[46]    H. Lee, C. Xia, and N. X. Fang, “First jump of microgel; actuation speed enhancement by elastic instability,” Soft Matter, vol. 6, no. 18, pp. 4342–4345, 2010.

[47]    K. Ikuta, D. Yajima, H. Ichikawa, and S. Katsuya, “Hydrodynamic active catheter with multi degrees of freedom motion,” in World Congress on Medical Physics and Biomedical Engineering 2006, 2007, pp. 3091–3094.

[48]    R. M. Erb, J. S. Sander, R. Grisch, and A. R. Studart, “Self-shaping composites with programmable bioinspired microstructures,” Nat Commun, vol. 4, no. 1, pp. 1–8, 2013.

[49]    Z. Wei et al., “Hybrid hydrogel sheets that undergo pre-programmed shape transformations,” Soft Matter, vol. 10, no. 41, pp. 8157–8162, 2014.

[50]    M. Pagitz, E. Lamacchia, and J. Hol, “Pressure-actuated cellular structures,” Bioinspir Biomim, vol. 7, no. 1, p. 016007, 2012.

[51]    B. Su, S. Gong, Z. Ma, L. W. Yap, and W. Cheng, “Mimosa‐inspired design of a flexible pressure sensor with touch sensitivity,” Small, vol. 11, no. 16, pp. 1886–1891, 2015.

[52]    Y. Wei, S. Chen, Y. Lin, Z. Yang, and L. Liu, “Cu–Ag core–shell nanowires for electronic skin with a petal molded microstructure,” J Mater Chem C Mater, vol. 3, no. 37, pp. 9594–9602, 2015.