You are here
Polar decomposition
Mon, 2008-09-08 23:04 - ramdas chennamsetti
Hi all,
I went through a topic on polar decomposition of deformation gradient. I understood the mathematics. I would like to know the physical significance and application of this. I request somebody to explain this.
Thanks in advance,
Regards,
- Ramdas
»
- ramdas chennamsetti's blog
- Log in or register to post comments
- 17013 reads


Comments
Polar decomposition means
Polar decomposition means the deformation of the object could be decomposited as the rotation plus stretching (Rotation Matrix * Stretching Matrix).
Ying Li
Department of Engineering Mechanics Tsinghua University Beijing, 100084, P. R. CHINA
Polar Decomposition
Hi Ramadas, here is a 46 page document entitled "Kinematics, the mathematics of deformation" from Prof. Brannon at University of New Mexico. The third chapter is devoted to the mathematics and clear physical explanations of polar decomposition. She has made nice drawings to illustrate the idea. http://www.mech.utah.edu/~brannon/public/Deformation.pdf
Hi Ying, Thank you. I
Hi Ying,
Thank you. I understood that. But, I need some physical significance.
Hi Temesgen Markos,
Thank you. This notes is very good. I will go through this. I tried to download 'Finite deformation theory' notes of Prof. Brannon from imechanica site (lecture notes of interest to imechanicians), but, it was showing some problem with their server. If you have full notes, I request you to send it.
Thank you,
Regards,
- Ramdas
Polar decomposition: Brannon notes links updated
I've updated the Brannon note links. Thanks for pointing out that they weren't working.
The polar decomposition is used in situations where one need to find the rotation of a material point (the stretches can be obtained from the square root of B or C - see Amit's discussion). The rotation has been used widely in codes based on a hypoelastic formulation. In those codes the stresses and the rates of deformation are rotated to the material description, the constitutive model is evaluated, and the updated stresses are rotated back to their spatial orientation. People are moving away from such models to more elegant (and correct?) hyperelastcity based models. The rotation can also be useful in other calculations - see for instance Belytschko, Liu, Moran's Nonlinear Finite Elements.
-- Biswajit
Uniqueness of the Polar Decomposition
Hi Ramdas,
You have got some good references on developing a physical feel for the Polar Decomposition. A good article (that contains much more than kinematics) is Rodney Hill's "Aspects of Invariance in Solid Mechanics" 1978 in Advances in Applied Mechanics. Chipping away at this one over a period of years will be very useful for learning nonlinear solid mechanics.
One very useful fact you must remember about the Polar Decomposition theorem is that the left and the right Polar decompositions are both unique. That is to say, given the information that you have an invertible tensor (F) you can produce one, and only one, stretch tensors U, V, and the rotation tensor R that satisfy F = RU = VR. So one use of this is that suppose in the course of some complicated argument you have two candidate formulae for the stretch tensor, say U, and let's say they do not look the same at all. By the uniqueness, you can immediately conclude that these two representations must be the same.
I know that this use of the Polar Decomposition does not address your question on the physical feel for the theorem, but this is an important property that often does not get described.
- Amit
Thank you...
Dear Sir,
Thank you very much.
Regards,
- Ramdas
Every bounded operator
Every bounded operator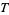 acting on a Hilbert
acting on a Hilbert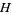 has a decomposition
has a decomposition 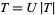 , where
, where 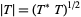 and
and 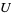 is a partial isometry.
is a partial isometry. is invertible,
is invertible, can be chosen to be unitary.
can be chosen to be unitary.
space
This decomposition is called polar decomposition. If
then