You are here
Journal Club Theme of April 2012: Phononics: Structural Dynamics of Materials
Engineering structures are made out of materials and as such there is a natural hierarchy in which a material’s intrinsic properties contribute to shaping up the structure’s response. It is possible however to reverse this hierarchy and engineer materials that are made out of structures. In this case, the intrinsic properties of a material are shaped by the structural response. Such a configuration can only be realized with a repeated structure, forming an array of identical unit cells. Of course the structural components in the unit cell will themselves be formed out of a constituent material (or more), but this is at another level and the properties of the overall material will be different from those of this constituent material. The emerging field of phononics is mostly concerned with this type of reverse construction, whereby one can conduct structural dynamics analysis and design towards the design of materials with desired properties in contrast to the conventional activity of direct design of structures with a desired response. There is various terminology in the literature for the description of this type of material, the most common are phononic materials, lattice materials and periodic materials. Figure 1 illustrates the concept of a “structure made out of a material” versus a “material made out of a structure”.
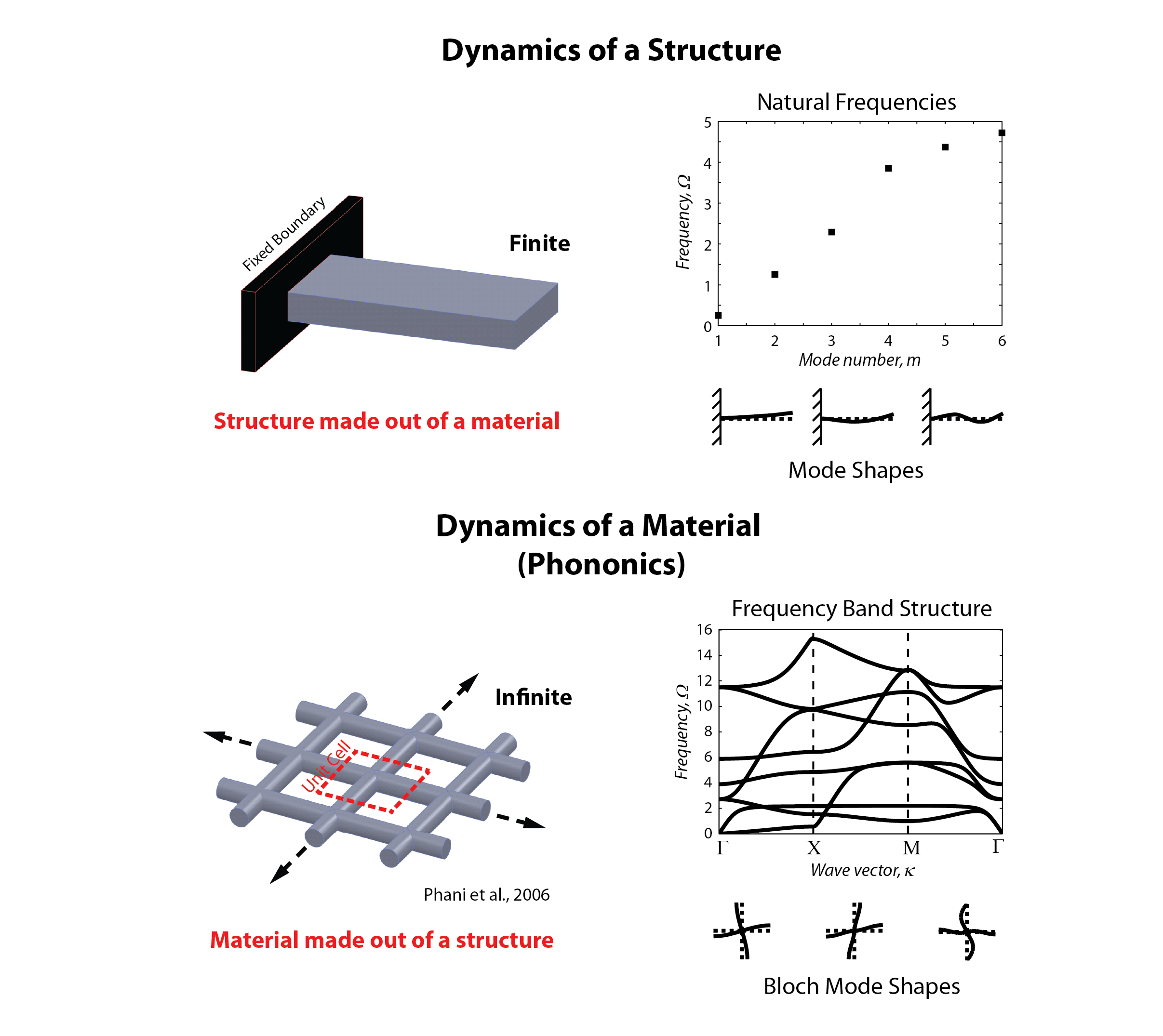
Figure 1: Demonstration of material-structure reversal in the formation of a phononic material unit cell.
So what are the implications and benefits of undergoing such a material-structure reversal, or having a material system for which we can analyze and control the dynamics rather than a conventional structural system? The answer is threefold, if not more:
-Unlike a conventional structure, which is finite in its spatial extent, a phononic material is in principle infinite and has local intrinsic properties. These properties, which are defined point-wise, include familiar static quantities, such as Young’s modulus and density, which can be obtained by a process of homogenization. This brings rise to the notions of “elasticity of a structure” and “density of a structure”. Not any less intriguing, a phononic material also exhibits frequency-dependent dynamic properties and this has recently been mathematically formulated by Willis, 2009 , Norris, 2011 and Nemat-Nasser et al., 2011. In Nemat-Nasser et al., 2011, an elegant scheme is proposed to uniquely obtain the effective dynamic density and effective dynamic modulus of a one-dimensional phononic material. The derived effective properties are shown to recover the dispersion relation, that is, the dispersion curves can be obtained using these effective properties alone.
-For a conventional structure, a dynamical characterization involves obtaining a single set of natural frequencies and mode shapes – which depend on many factors including the size of the structure and its boundary conditions. In a phononic material, we obtain an infinite number of sets of natural frequencies and mode shapes, one for each specific wavenumber (or wave vector) within what is known as the Brillouin zone (BZ). (The BZ describes an irreducible unit cell in the space of wavenumbers as opposed to the unit cell shown in Fig. 1 which is described in real space.) The implication of this difference is significant as it implies a much larger space of dynamic properties and hence much more opportunities for dynamical functionalities. For an engineer, this in turn provides a much richer design problem as now the dynamics is described in terms of both spatial and temporal frequencies as opposed to only temporal frequencies in a conventional structure. This space-time frequency space is what is commonly known as the frequency band structure (as shown in Fig. 1). It is possible to design the structural features of the unit cell in a manner that allows one to control the shape of this frequency band structure (and hence control the dynamics in this wider space of variables). See for example, the papers by Sigmund and Jensen, 2003 and Bilal and Hussein, 2011 which have tackled this problem through unit cell topology optimization.
-It is possible to link the two entities shown in Fig. 1 and form structures composed of a phononic material or more than one phononic material. In this manner a hierarchical structure-material-structure-material construction may be realized. (One more “material” is added at the end of this four-term expression because as mentioned above the structure from which a phononic material unit cell is composed is in itself made out of a material, or more). This process of designing structures using phononic materials, which is referred to as multiscale dispersive design (see Hussein et al., 2007), allows the designer to dynamically “patch” different parts of a structure to allow a remarkable level of dynamical functionality and control. For example, in Hussein et al., 2007, a rod is considered that is subjected to two excitations at different locations and different frequencies. Using this design approach, the rod may consist primarily of a regular homogeneous material but also partially from two distinct types of phononic materials (see Fig. 2). Each of these two phononic materials in turn is designed to exhibit a band gap at a specific frequency of excitation and is located as a patch at/around the location of the corresponding excitation source. (A band gap is a frequency range in which no wave propagation is permitted). The result is that the forced response of the structure as a whole is significantly less compared to a statically equivalent fully homogenous rod. One can envisage the application of this methodology to many problems in vibration control in which there are multiple excitation sources and/or a desire to spatially tailor the dynamical response of an engineering structure.
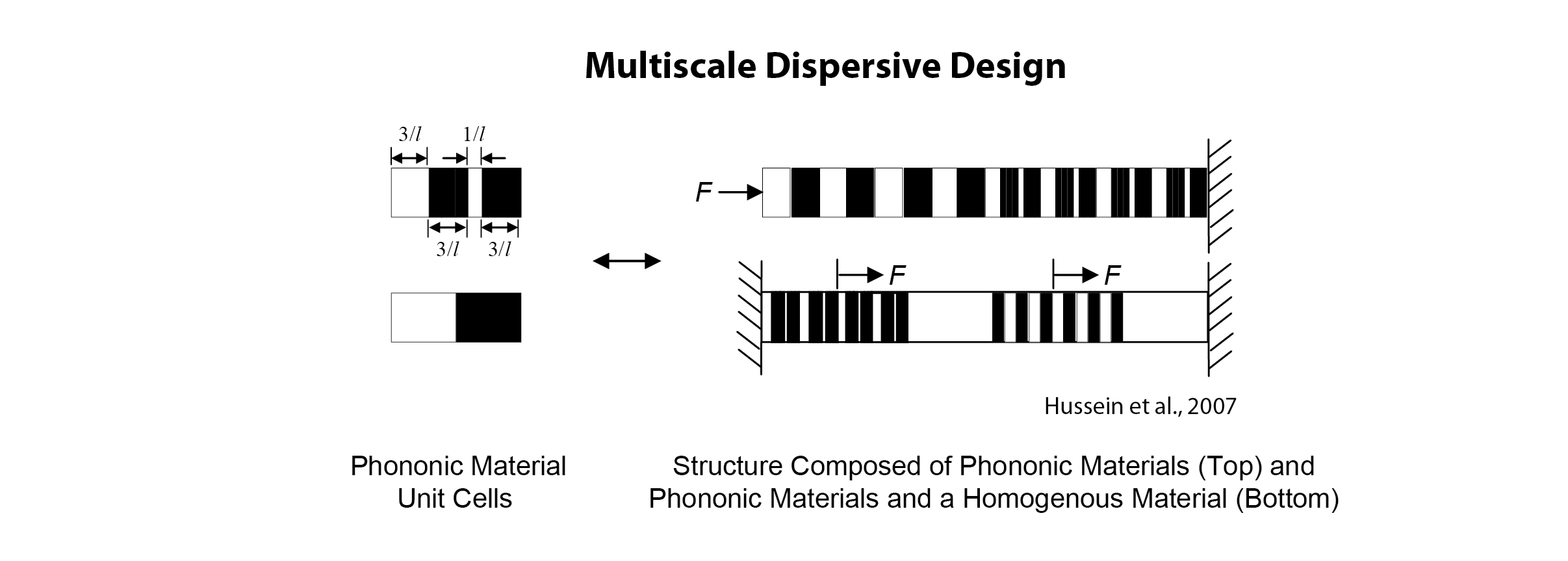
Figure 2: Demonstration of the Multiscale Dispersive Design methodology
Another aspect of interest in phononic materials is the nature of the band gap opening mechanism, which may be based on Bragg scattering (like the cases considered above) or local resonance. For further reading, there are numerous references in the literature, e.g., Kushwaha et al., 1993, Sigalas and Economou, 1993 and Liu et al., 2000. Also of interest is the development of models of phononic materials that incorporate damping (Hussein and Frazier, 2010 , Farzbod and Leamy, 2011) and nonlinearity (Porter et al., 2008 , Narisetti et al., 2011). The December 2007 edition of the iMechanica Journal club (posted by Biswajit Bannerjee) discusses the related topic of “Elastodynamic band gaps and metamaterials”, and the upcoming May 2012 edition (to be posted by Alessandro Spadoni) will also discuss this area of research.
References
Phani, A.S., Woodhouse, J. and Fleck, N.A., “Wave propagation in two-dimensional periodic lattices ,” Journal of the Acoustical Society of America, 119, 1995-2005, 2006.
Willis J. R., “Exact effective relations for dynamics of a laminated body ,” Mechanics of Materials, 41, 385-393, 2009.
Norris, A.N., “Effective Willis constitutive equations for periodically stratified anisotropic elastic media ,” Proceedings of the Royal Society A-Mathematical, Physical and Engineering Sciences, 467, 1749-1769, 2011.
Nemat-Nasser, S., Willis, J.R., Srivastava, A. and Amirkhizi, A.V., “Homogenization of periodic elastic composites and locally resonant sonic materials ,” Physical Review B, 83, 104103, 2011.
Sigmund, O. and Jensen, J.S., “Systematic design of phononic band-gap materials and structures by topology optimization ,” Philosophical Transactions of the Royal Society of London, series A-Mathematical, Physical and Engineering Sciences, 361, 1001-1019, 2003.
Bilal, O.R. and Hussein, M.I., “Ultrawide phononic band gap for combined in-plane and out-of-plane waves ,” Physical Review E, 84, 065701(R), 2011.
Hussein, M.I., Hulbert, G.M. and Scott, R.A., “Dispersive elastodynamics of 1D banded materials and structures: Design ,” Journal of Sound and Vibration, 307, 865-893, 2007.
Kushwaha, M.S., Halevi, P., Dobrzynski, L. and Djafari-Rouhani, B., “Acoustic band-structure of periodic elastic composites ,” Physical Review Letters, 71, 2022-2025, 1993.
Siglas, M. and Economou, E.N., “Band-structure of elastic waves in 2-dimensional systems ,” Solid State Communications, 86, 141-143, 1993.
Liu, Z.Y., Zhang, X.X., Mao, Y.W., Zhu, Y.Y., Yang, Z.Y., Chan, C.T. and Sheng, P., “Locally resonant sonic materials ,” Science, 289, 1734-1736, 2000.
Hussein, M.I. and Frazier, M.J., “Band structure of phononic crystals with general damping ,” Journal of Applied Physics; 108, 093506, 2010.
Farzbod, F. and Leamy, M.J., "Analysis of Bloch's method in structures with energy dissipation ," Journal of Vibration and Acoustics, 133, 051010, 2011.
Porter, M.A., Daraio, C., Herbold, E.B., Szelengowicz, I. and Kevrekidis, P.G., “Highly nonlinear solitary waves in periodic dimer granular chains ,” Physical Review E, 77, 015601, 2008.
Narisetti, R.K., Ruzzene, M. and Leamy, M.J., 2011, "A perturbation approach for analyzing dispersion and group velocities in two-dimensional nonlinear periodic lattices ," Journal of Vibration and Acoustics, 133, 061020, 2011.
- mihussein's blog
- Log in or register to post comments
- 27107 reads


Comments
Nice Job
Nice Job Mahmoud
Cheers
Massimo
M. Ruzzene
Re: Phononics theory and experiment
I'd like to point out another direction in which this area of reseach is progressing - the guiding of elastic waves. Prof. William Parnell has a couple of recent papers on the subject:
1) Nonlinear pre-stress for cloaking from antiplane elastic waves http://arxiv.org/abs/1203.3246
2) Employing pre-stress to generate finite cloaks for antiplane elastic waves http://arxiv.org/abs/1203.3243
Periodicity may or may not be important/useful under these conditions.
The ratio of experiment to theory/numerics papers in the literature tends to 0 in the field. That gap needs to be closed.
-- Biswajit
Reply to Biswajit
Dear Biswajit,
Indeed the guiding of elastic (or acoustic) waves is one of the interesting applications in this area. The following paper considers a phononic crystal waveguide:
-Khelif, A., Choujaa, A., Benchabane, S., Djafari-Rouhani, B., and Laude, V., Guiding and bending of acoustic waves in highly confined phononic crystal waveguides, Appl. Phys. Lett. 84, 4400, 2004;
And this paper considers a locally resonant material waveguide:
-Oudich, M., Assouar, M. B., and Hou, Z., “Propagation of acoustic waves and waveguiding in a two-dimensional locally resonant phononic crystal plate ,” Appl. Phys. Lett., 97, 193503, 2010;
This is in addition to numerous papers that achieve waveguiding using transformation acoustics, such as:
-Cummer, S. A., Schurig, D., One Path to Acoutic Cloaking , New J. Phys., 9 45, 2007.
Regarding experiments, the field can certainly benefit from more experimental investigations. Here are some references that involve experiments (concerning phononic crystals, acoustic metamaterials, and applications)
-Liu, Z., Zhang, X., Mao, Y., Zhu, Y. Y., Yang, Z., Chan, C. T., and Sheng, P., Locally Resonant Sonic Materials , Science, 289, 1734, 2000.
-Vasseur, J. O., Deymier, P. A., Khelif, A., Lambin, Ph., Djafari-Rouhani, B., Akjouj, A., Dobrzynski, L., Fettouhi, N., and Zemmouri, J., Phononic crystal with low filling fraction and absolute acoustic band gap in the audible frequency range: A theoretical and Experimental Study , Phys. Rev. E, 65, 056608, 2002
-Morvan, B., Tinel, A., Hladky-Hennion, A., Vasseur, J., and Dubus, B., Experimental demonstration of the negative refraction of a transverse elastic wave in a two-dimensional solid phononic crystal , Appl. Phys. Letts., 96, 101905, 2010.
-Zhu., J., Christensen, J., Jung, J., Martin-Moreno, L., Yin, X., Fok, L., Zhang X., and Garcia-Vidal, F. J., A holey-structured metamaterial for acoustic deep-subwavelength imaging , Nature Phys., 7, 52-55, 2011.
-Moiseyenko, R. P., Herbison, S., Declercq, N. F., and Laude, V., Phononic Crystal diffraction Gratings , J. Appl. Phys., 111, 034907, 2012.
Best,
Mahmoud
M. Ruzzene
M. Ruzzene
A very nice article Dr.
A very nice article Dr. Hussein. In my opinion it is high time that the heirarchichal nature of such periodic composites is tackled within a predictive quantitative framework. Homogenization is going to be important for this and we have started working on some simple experiments regarding this. The essential theory of the coupled dynamic constitutive relations for 2-, and 3-D (recent papers by Willis, Norris and our group) remain very mathematically involved. Still, I am reasonably convinced that homogenization of the Willis form would end up being very important even in experiments. It would be a nice comprehensive framework then. Wave dynamics above the first branch, displaying exotic phenomenon like stop-bands and pass-bands could be designed using band-structure optimization and dynamics over the first branch (and the second in some cases) could be more finely controlled using homogenization.
Re: A very nice article Dr.
This is a good point, Ankit. Optimization can very well be combined
with homogenization at both levels: long wave (low frequency) and
dynamic (high frequency). This as well as homogenization-related
experiments are two very promising avenues.
Best,
Mahmoud
A timely discussion topic
A very nice overview Mahmoud.
Ankit: your ideas on dynamic homogenisation are interesting. Can these representations capture the elatic boundary layers that develop at a free surface under static loading, such as the ones described in the following article?
Elastic Boundary Layers in Two-Dimensional Isotropic Lattices
J. Appl. Mech. -- March 2008 -- Volume 75, Issue 2, 021020 (8 pages)
http://dx.doi.org/10.1115/1.2775503
Hi Srikanth, It's nice to
Hi Srikanth,
It's nice to get in touch with you again after Santa Fe last year. I will have to read your paper carefully before commenting upon the applicability of the Willis form to elastic boundary layers. The theory says that the effective properties are dependent upon the 'kind' of boundary conditions under which they are calculated especially near the boundaries. Dr. Willis once mentioned that these effective properties should give rise to effective field variables which are essentially free of the boundary conditions away from the boundaries. This result is non-trivial and remains to be proven from what I understand.
I will get back to you on your specific query to the best of my uderstanding!
Ankit