You are here
Journal Club for November 2018: Beyond piezoelectricity: Flexoelectricity in solids
Beyond piezoelectricity: Flexoelectricity in solids
Jiawang Hong
School of Aerospace Engineering, Beijing Institute of Technology
1. Introduction
Piezoelectricity, describing the linear coupling between electric polarization and strain, plays an important role in the energy transfer between mechanical and electric energy. However, piezoelectricity only arises in the noncentrosymmetric materials and the most widely used piezoelectric devices are lead-based which is not environmental friendly. Meanwhile, piezoelectricity may vanish as size reduces below the critical size. It will be of great importance to have new electromechanical coupling effect which can overcome these drawbacks of piezoelectricity and still efficiently transfer mechanical energy to electric energy and vice versa.Flexoelectricity, describing the linear coupling between electric polarization P_i and a strain gradient \epsilon_{kl} (Eq. 1 and Figure 1), is such new electromechanical coupling effect.
Flexoelectricity was theoretically proposed more than 50 years ago[1] and was discovered experimentally four years later by Scott[2] and Bursian et al.[3]The flexoelectric effect was overlooked for decades because of its relatively weak effects. Recently, it has attracted increasing attention due to much higher flexoelectric response observed in bending measurements, which is three orders larger than previous theoretical estimations.[4] The change coupling of polarization from strain to strain gradient causes huge difference between piezoelectricity and flexoelectricity: Flexoelectricity can exist in all insulators due to strain gradient breaking the inversion symmetry of the materials. This provides us much more candidate materials from simple silicon to complex perovskites for electromechanical applications. In addition, flexoelectric effect will be more pronounced with size reducing as the strain gradient can increase by 6-7 orders of magnitude at nanoscale compared with bulk materials.[5]


Figure 1. Schematic illustration of flexoelectric effect based on an ionic crystal.[6] The strain gradient induces the center of negative charge away from the center of positive charge, causing the flexoelectric polarization in centrosymmetric materials.
2. Novel phenomena from flexoelectricity in nanostructures
Due to much higher strain gradient at nanoscale, flexoelectricity is expected to have a significant effect on the properties of nanostructures. For example, the transition temperature, distribution of polarization, hysteresis curves, domain walls and even the optical properties can be significantly influenced in nanostructures due to flexoelectricity.[5,7-13]Recently, it was demonstrated that the polarization domains can be written in purely mechanical way in in thin BaTiO3 films and high-density data-storage memories were proposed due to flexoelectricity (Figure 2a).[14] The polarizations in PbTiO3 films can be rotated by the in-plane flexoelectricity and could enhance the piezoelectricity (Figure 2b). [15] Interestingly, the flexoelectricity can be used as a novel tool to control the polarization switching path (Figure 2c)[16]and the oxygen vacancy distribution by using the mechanical force of AFM tip.[17] It can also be used to manipulate molecular alignment at nanoscale through the charge concentration on the crinkles of graphene due to the flexoelectric effect. [18,19] Flexoelectricity induces some novel phenomena and enhances properties at nanoscale, but it can weaken bulk properties sometimes. For example, flexoelectricity can induce the decrease of the dielectric constant[20,21]and an increase in critical thickness[22]in ferroelectric thin films. Fortunately, this effect could be removed by applying a proper external electric field.[23]

Figure 2. (a) Sketch of the strain gradient and associated flexoelectric field (arrows) induced by the AFM tip pushing on the surface of heterostructure and the domains written by AFM tip.[14] (b) Sketch of stresses, strain gradients and polarization vectors in the twinned film.[8] (c) Schematic of polarization switching due to the trailing flexoelectric field tracing the SPM tip motion, and phase-field modelling of in-plane flexoelectric distribution under both a static and mobile tip.[16]
3. Characterizing flexoelectric coefficients
Since the fourth rank flexoelectric coefficient tensor, \mu_{ijkl}, is the key parameter for quantifying the flexoelectric effect (Eq.1), it is critical to characterize full tensor components in order to understand the flexoelectric effect in complicated strain state, as well as design flexoelectric devices. The characterization of flexoelectric coefficient(FEC) has being one of the most concerned topic in flexoelectric field.
Experimentally, dynamically bending a beam is widely used method to induce a strain gradient and measure the polarization (current) to obtain the flexoelectric coefficient (Figure 3a), which is about the order of uC/m, 3-4 orders higher than the theory prediction and first-principles calculations. [24-28] However, what measured from these methods is the effective flexoelectric coefficient, which is the combination of the flexoelectric coefficient tensor component. [29,30] Different crystal orientations of single crystal ([100], [110] and [111]) were measured in order to obtain the individual tensor component, [29] but it was found that the effective FEC from three different crystal oriented beams linearly depends on each other (Eq.2), indicating bending measurement alone can’t obtain the full FEC tensor component[29,30], even for the cubic symmetry material (like SrTiO3) which only has three independent FEC tensor components. Other methods, like compressing a truncated pyramid[4], a shock wave[31] or split Hopkinson pressure bar[32], as well as nano-indentation[33] etc. were developed recently to measure FEC. However, there is still lack of a standard approach to measure the full FEC tensor component, even for the simplest cubic symmetry materials. All the methods above are required to measure electric current under the dynamical mechanical loading, which is not convenient at nanoscale. Especially, it is difficult to distinguish the contribution from the piezoelectricity and flexoelectricity when measuring the current for ferroelectric materials, indicating the effective flexoelectric coefficient may also include the piezoelectric contribution. One solution is to perform the measurement at high temperature beyond the Cure temperature to avoid bulk piezoelectric effect. However, the surface piezoelectric polar regions could persist far above Cure temperature and still have surface piezoelectric effect to the flexoelectricity.[34] Recently, a pure mechanical method was proposed to obtain FEC by measuring the stiffness of variable cross-section nanorod (Figure 3b) to avoid applying dynamical loading and electric current measurement, based on an analytical model. [35] However, this method still needs to be verified by the measurement.
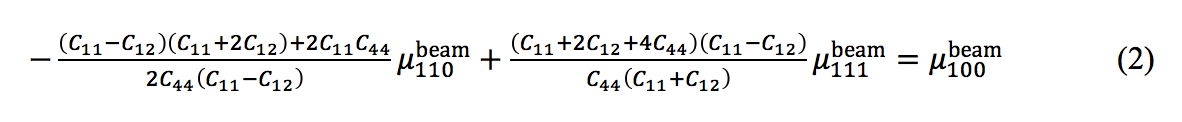

Figure 3. (a) The setup of the three-point bending measurement of FEC.[29] (b) The nano-compression method to measure stiffness to obtain FEC.[35]
Theoretically, a rigid-ion model was developed to calculate FEC.[28,36] In order to maintain periodic boundary condition after applying strain gradient in simulation, an accordion model (Figure 4) was proposed to calculate the longitudinal FEC component[27] and later on it was extended to calculate the transverse component as well.[37,38] However, FEC is the order of nC/m from the theory and first-principles calculations,[39] which is 3-4 orders of magnitude smaller than the experimental value. And this is a long puzzle in the flexoelectric community. Recently, a microscopic theory was developed to understand the origin of the flexoelectricity, [39,40] showing the flexoelectricity comes from the pure electron contribution and the coupling between the electron and lattice distortion. Interestingly, it was shown that the intrinsic FEC linearly depends on the dielectric constant, being in consistent with previous experimental observation [4] and providing a simple thumb rule to search for high FEC materials. Meanwhile, a large FEC discrepancy between experiment and theory was also partially resolved from this microscopic theory: the FEC values from previous first-principles calculations and experiment are used different electronic boundary condition (BC). Measurement usually is done at short circuit BC (constant electric field) while the calculation is done at open circuit BC (constant electric displacement field). The microscopic theory says that their discrepancy of FEC origins from the dielectric constant.[39] For example, the FEC for BaTiO3 single crystal is ~2500 nC/m,[30]and the first-principles gives 0.15 nC/m. [39] After considering the different BC and the dielectric constant of 2300 for BaTiO3, the discrepancy decrease from 4 orders of magnitude to less than 7 times. If the external factor (such as surface effect [41]) is considered, the experiment and theory value will be more close. The FEC theory was developed fast in the last few years and the calculation method was implemented recently,[42-44] which allows for the efficient calculation of the full FEC tensor for different materials.

Figure 4. The “accordion” model for calculating FEC with periodic strain gradient.[27]
4. Flexoelectricity in smart structures and devices
A macroscopic flexoelectric theoretical framework was developed about ten years ago for the flexoelectric applications in smart materials and structures.[45,46] Based on the flexoelectric phenomenological theory, numbers of analytical models have been proposed to design the flexoelectric devices for the energy harvesting.[47-52] For example, it was found that the flexoelectricity can dramatically enhance energy harvesting for a narrow range of dimensions in piezoelectric nanostructures[53], which provides a scheme for engineering energy scavenging. Some piezoelectric devices (Figure 5a, 5b) based on the flexoelectric effect have been proposed and their effective piezoelectric response has been measured.[54-57]Recently, the concept of the flexoelectric nanogenerator was proposed[58] which enlightens a new technique for energy harvesting. This flexoelectric nanogenerator could yield enhanced performance with specific nanostructures and provide a wider materials choice, compared with piezoelectric counterpart.[59]
The proposed flexoelectric structures show promising working performance, however, there are very rare flexoelectric devices in application due to the relative complex composition of those materials and great difficulties in the fabrication of corresponding nanostructures. Recently, a nano-sized flexoelectric cantilever actuator (Figure 5c) has been fabricated and its performance exceeds that of the best piezoelectric devices.[60] A flexoelectric strain gradient sensor was also designed for monitoring crack and characterizing the opening mode stress intensity factor. [61] Moreover, based on the vibration of dual cantilever beam, the flexoelectricity was introduced in the design of microphone, which possesses high sensitivity and a wide working frequency range simultaneously. [62] With the development of flexoelectric characterization and the nanostructure fabrication technology, it is expected that more high performance flexoelectric devices will be applied in energy harvesting, sensing and actuating in the near future.

Figure 5. (a) A flexure-mode devices based on transverse flexoelectric effect.[56,58] (b) A piezoelectric composite based on the longitudinal flexoelectric effect.[57,58] (c) The flexoelectric cantilever actuator.[60]
5. Concluding Remarks
Strain was widely used to tune and enhance the device performance in last decades and “strain engineering” is well developed.[63] Since the flexoelectricity shows novel properties and promising applications in nanodevices, the “strain gradient engineering”, which refers to the general strategy to induce, tune and improve the materials properties by strain gradient, is attracting intensive attention. In addition to coupling with electric polarization, strain gradient can also couple with with other properties, such as magnetic, chemical and photovoltaic properties etc, which leads to some novel phenomena, like flexomagnetic[7,64-66], flexochemical[17,67,68] and flexophotovoltaic effect[69] etc. For all these strain gradient coupling effects, one of the big challenges is to characterize the full coupling coefficient tensor component from theoretical and experimental aspect at different strain gradient conditions, as well as to build the database of the related materials parameters. This is crucial for designing the flexodevices working in the complicated strain distribution environment.
References:
[1] S. M. Kogan, Soviet Physics-Solid State 5, 2069 (1964).
[2] J. F. Scott, The Journal of Chemical Physics 48, 874 (1968).
[3] E. V. Bursian and Zaikovsk.Oi, Soviet Physics Solid State,Ussr 10, 1121 (1968).
[4] L. E. Cross, Journal of Materials Science 41, 53 (2006).
[5] D. Lee, A. Yoon, S. Y. Jang, J. G. Yoon, J. S. Chung, M. Kim, J. F. Scott, and T. W. Noh, Physical Review Letters 107, 057602 (2011).
[6] R. Maranganti, N. D. Sharma, and P. Sharma, Physical Review B 74 (2006).
[7] E. A. Eliseev, A. N. Morozovska, M. D. Glinchuk, and R. Blinc, Physical Review B 79, 165433 (2009).
[8] G. Catalan et al., Nature Materials 10, 963 (2011).
[9] P. Gao, S. Yang, R. Ishikawa, N. Li, B. Feng, A. Kumamoto, N. Shibata, P. Yu, and Y. Ikuhara, Physical Review Letters 120, 267601 (2018).
[10] P. V. Yudin, A. K. Tagantsev, E. A. Eliseev, A. N. Morozovska, and N. Setter, Physical Review B 86, 134102 (2012).
[11] E. A. Eliseev, A. N. Morozovska, G. S. Svechnikov, P. Maksymovych, and S. V. Kalinin, Physical Review B 85, 045312 (2012).
[12] X. W. Fu et al., Frontiers of Physics 8, 509 (2013).
[13] X. F. Xu, L. M. Jiang, and Y. C. Zhou, Smart Materials and Structures 26, 115024 (2017).
[14] H. Lu, C. W. Bark, D. Esque de los Ojos, J. Alcala, C. B. Eom, G. Catalan, and A. Gruverman, Science 336, 59 (2012).
[15] R. Tararam, I. K. Bdikin, N. Panwar, J. A. Varela, P. R. Bueno, and A. L. Kholkin, Journal of Applied Physics 110, 052019 (2011).
[16] S. M. Park, B. Wang, S. Das, S. C. Chae, J. S. Chung, J. G. Yoon, L. Q. Chen, S. M. Yang, and T. W. Noh, Nature Nanotechnology 13, 366 (2018).
[17] S. Das et al., Nature Communications 8, 615 (2017).
[18] M. Kothari, M. H. Cha, and K. S. Kim, Proceedings of the Royal Society A 474, 20180054 (2018).
[19] R. Li, M. Kothari, A. K. Landauer, M.-H. Cha, H. Kwon, and K.-S. Kim, MRS Advances 3, 2763 (2018).
[20] G. Catalan, B. Noheda, J. McAneney, L. J. Sinnamon, and J. M. Gregg, Physical Review B 72 (2005).
[21] G. Catalan, L. J. Sinnamon, and J. M. Gregg, Journal of Physics: Condensed Matter 16, 2253 (2004).
[22] H. Zhou, J. W. Hong, Y. H. Zhang, F. X. Li, Y. M. Pei, and D. N. Fang, Physica B-Condensed Matter 407, 3377 (2012).
[23] H. Zhou, J. W. Hong, Y. H. Zhang, F. X. Li, Y. M. Pei, and D. N. Fang, EPLl 99, 47003 (2012).
[24] W. Ma and L. E. Cross, Applied Physics Letters 79, 4420 (2001).
[25] W. H. Ma and L. E. Cross, Applied Physics Letters 78, 2920 (2001).
[26] W. H. Ma and L. E. Cross, Applied Physics Letters 81, 3440 (2002).
[27] J. Hong, G. Catalan, J. F. Scott, and E. Artacho, Journal of physics. Condensed matter, 22, 112201 (2010).
[28] A. K. Tagantsev, Phys Rev B 34, 5883 (1986).
[29] P. Zubko, Physical Review Letters 99 (2007).
[30] J. Narvaez, S. Saremi, J. W. Hong, M. Stengel, and G. Catalan, Physical Review Letters 115, 037601 (2015).
[31] T. T. Hu, Q. Deng, X. Liang, and S. P. Shen, Journal of Applied Physics 122, 055106 (2017).
[32] T. Hu, Q. Deng, and S. Shen, Applied Physics Letters 112, 242902 (2018).
[33] M. Gharbi, Z. H. Sun, P. Sharma, K. White, and S. El-Borgi, International Journal of Solids and Structures 48, 249 (2011).
[34] A. Ziębińska, D. Rytz, K. Szot, M. Górny, and K. Roleder, Journal of Physics: Condensed Matter 20, 142202 (2008).
[35] H. Zhou, Y. M. Pei, J. W. Hong, and D. N. Fang, Applied Physics Letters 108, 101908 (2016).
[36] R. Maranganti and P. Sharma, Physical Review B 80, 054109 (2009).
[37] T. Xu, J. Wang, T. Shimada, and T. Kitamura, Journal of Physics-Condensed Matter 25, 415901 (2013).
[38] I. Ponomareva, A. K. Tagantsev, and L. Bellaiche, Physical Review B 85, 104101 (2012).
[39] J. W. Hong and D. Vanderbilt, Physical Review B 88, 174107 (2013).
[40] M. Stengel, Physical Review B 88, 174106 (2013).
[41] X. Zhang, Q. Pan, D. Tian, W. Zhou, P. Chen, H. Zhang, and B. Chu, Physical Review Letters 121, 057602 (2018).
[42] C. E. Dreyer, M. Stengel, and D. Vanderbilt, Physical Review B 98 (2018).
[43] M. Stengell and D. Vanderbilt, Physical Review B 98, 125133 (2018).
[44] M. Kothari, M.-H. Cha, and K.-S. Kim, Proceedings of the Royal Society a-Mathematical Physical and Engineering Sciences 474, 20180054 (2018).
[45] R. Maranganti, N. D. Sharma, and P. Sharma, Physical Review B 74, 014110 (2006).
[46] S. P. Shen and S. L. Hu, Journal of the Mechanics and Physics of Solids 58, 665 (2010).
[47] M. S. Majdoub, P. Sharma, and T. Cagin, Physical Review B 77, 125424 (2008).
[48] X. Liang, S. L. Hu, and S. P. Shen, Smart Materials and Structures 23, 035020 (2014).
[49] L. Qi, S. J. Zhou, and A. Q. Li, Composite Structures 135, 167 (2016).
[50] N. S. Rupa and M. C. Ray, International Journal of Mechanics and Materials in Design 13, 453 (2017).
[51] L. Qi, S. Huang, G. Fu, S. Zhou, and X. Jiang, International Journal of Engineering Science 124, 1 (2018).
[52] Q. Deng and S. Shen, Smart Materials and Structures 27 (2018).
[53] M. S. Majdoub, P. Sharma, and T. Cagin, Physical Review B 78, 121407 (2008).
[54] J. Fousek, L. E. Cross, and D. B. Litvin, Materials Letters 39, 287 (1999).
[55] J. Y. Fu, W. Y. Zhu, N. Li, and L. E. Cross, Journal of Applied Physics 100, 024112 (2006).
[56] B. J. Chu, W. Y. Zhu, N. Li, and L. E. Cross, Journal of Applied Physics 106, 104109 (2009).
[57] J. Y. Fu, W. Y. Zhu, N. Li, N. B. Smith, and L. E. Cross, Applied Physics Letters 91, 182910 (2007).
[58] X. N. Jiang, W. B. Huang, and S. J. Zhang, Nano Energy 2, 1079 (2013).
[59] Z. L. Wang and J. Song, Science 312, 242 (2006).
[60] U. K. Bhaskar, N. Banerjee, A. Abdollahi, Z. Wang, D. G. Schlom, G. Rijnders, and G. Catalan, Nature Nanotechnology 11, 263 (2016).
[61] W. B. Huang, S. R. Yang, N. Y. Zhang, F. G. Yuan, and X. N. Jiang, Experimental Mechanics 55, 313 (2015).
[62] S. R. Kwon, W. B. Huang, S. J. Zhang, F. G. Yuan, and X. N. Jiang, Journal of Micromechanics and Microengineering 26, 045001 (2016).
[63] J. Li, Z. Shan, and E. Ma, MRS Bulletin 39, 108 (2014).
[64] E. A. Eliseev, M. D. Glinchuk, V. Khist, V. V. Skorokhod, R. Blinc, and A. N. Morozovska, Physical Review B 84, 174112 (2011).
[65] P. Lukashev and R. F. Sabirianov, Physical Review B 82, 094417 (2010).
[66] J. H. Lee et al., Physical Review B 96, 064402 (2017).
[67] D. Lee et al., Advanced Materials 26, 5005 (2014).
[68] E. A. Eliseev, I. S. Vorotiahin, Y. M. Fomichov, M. D. Glinchuk, S. V. Kalinin, Y. A. Genenko, and A. N. Morozovska, Physical Review B 97 (2018).
[69] M. M. Yang, D. J. Kim, and M. Alexe, Science 360, 904 (2018).
- hongjw04's blog
- Log in or register to post comments
- 15868 reads


Comments
Computational models of flexoelectricity
Dear Jiawang,
Thank you for sharing this very nice review. I would like to bring to attention some of our recent works on computational modeling of flexoelectricity and its applications.
Mathematically, the self-consistent electromechanical field equations of flexoelectricity are a coupled system of fourth-order partial differential equations. Despite that analytical solutions are starting to emerge for simple geometries and loads [1,2], most of the field operates with approximate solutions that are valid under very restrictive assumptions [3,4]. Furthermore, to interpret experiments, the two-way flexoelectric coupling is often ignored [5]. To go beyond these simple approximate solutions, one can resort to computational methods, but because the equations involve high-order spatial derivatives, flexible methods such as conventional finite elements cannot be used. On rectangular or brick geometries, finite difference calculations have been applied to flexoelectricity [6-8]. To deal with more general geometries with nonuniform grid refinement, we have recently resorted to mesh-free methods, relying on smooth basis functions, to solve numerically the continuum equations of flexoelectricity in two [9] and three dimensions [10]. Surprisingly, we found that previous simplified calculations on beam and truncated triangle configurations provided only rough order-of-magnitude estimations of the flexoelectric response. These observations can partially explain the discrepancy between different experimental measurements and theoretical estimates [11]. We have also employed this computational model to study the manifestations of flexoelectricity in the fracture mechanics of piezoelectrics [12] and constructive and destructive interplay between piezoelectricity and flexoelectricity in flexural sensors and actuators [13].
[1] M. C. Ray, J. Appl. Mech. 81, 091002 (2014).
[2] S. Mao and P. K. Purohit, J. Appl. Mech. 81, 081004 (2014).
[3] Z. Yan and L. Y. Jiang, J. Appl. Phys. 113, 194102 (2013).
[4] M. S. Majdoub, P. Sharma, and T. Cagin, Phys. Rev. B 79, 119904 (2009).
[5] P. Zubko, G. Catalan, P. R. L. Welche, A. Buckley, and J. F. Scott, Phys. Rev. Lett. 99, 167601 (2007).
[6] R. Ahluwalia, A. K. Tagantsev, P. Yudin, N. Setter, N. Ng, and D. J. Srolovitz, Phys. Rev. B 89, 174105 (2014).
[7] Y. Gu, M. Li, A. N. Morozovska, Y. Wang, E. A. Eliseev, V. Gopalan, and L.-Q. Chen, Phys. Rev. B 89, 174111 (2014).
[8] H. Chen, A. Soh, and Y. Ni, Acta Mech. 225, 1323 (2014).
[9] A. Abdollahi, C. Peco, D. Millan, M. Arroyo, and I. Arias, J. Appl. Phys. 116, 093502 (2014).
[10] A. Abdollahi, D. Millan, C. Peco, M. Arroyo, and I. Arias, Phys. Rev. B 91, 104103 (2015).
[11] P. Zubko, G. Catalan, and A. K. Tagantsev, Annu. Rev. Mater. Res. 43, 387 (2013).
[12] A. Abdollahi and I. Arias, Phys. Rev. B 92, 094101 (2015).
[13] A. Abdollahi and I. Arias, J. Appl. Mech. 82, 121003 (2015).
Dear Amir,
Thank you very much for bringing attention of your recent works on computational modeling of flexoelectricity and its applications. They are very nice works! Your work shows the previous simplified model may cause one order of magnitude overestimation of the flexoelectric coefficient. Including this effect (1 order of magnitude), surface effect, as well as the different electric boundary condition used in theory and experiment (2-3 order of magnitude), it would be expected that the large discrepancy (3-4 orders of magnitude) between theory and experiment will be small or even disappear.
Best,
Jiawang
Dear Jiawang,
Dear Jiawang,
Thank you for your interest on our works. I would also like to share our recent work published in Physical Review Letters. In this paper, we have shown that piezoelectricity can indeed imitate flexoelectricity on the condition that the piezoelectric coefficient is inhomogeneously and asymmetrically distributed across the sample. If this condition is met, asymmetric piezoelectricity becomes indistinguishable from intrinsic flexoelectricity in single cantilever-bending experiments. Our calculations show that, for standard perovskite ferroelectrics, even a tiny gradient of piezoelectricity (1% variation of piezoelectric coefficient across 1 mm) is sufficient to yield a giant effective flexoelectric coefficient of 1 μC/m, three orders of magnitude larger than the intrinsic expectation value. This mimicry complicates the task of interpreting experimental results although we have suggested some approaches to separate inhomogeneous piezoelectricity from flexoelectricity.
https://journals.aps.org/prl/abstract/10.1103/PhysRevLett.121.205502
flexoelectricity enables domain at 800 °C
Hello Jiawang,
We recently found domain-like nanoregions (DLNRs) up to an extreme temperature of 800 °C in a NBT-25ST core–shell nanoparticles by TEM. By applying electric field to the nanoparticles in temperature-dependent in-situ TEM, the change of DLNRs indicates a polorizaiton-switching-like behavior. By combing the TEM observation and phase-field simulation including flexoelectricity, we found that the DLNRs at extreme temperature is possibly attributed to the flexoelectricity which is originated from the strain gradient induced by the diffusion of strontium cations at high temperatures.
Ref:
Enabling nanoscale flexoelectricity at extreme temperature by tuning cation diffusion. Nature Communicationsvolume 9, Article number: 4445 (2018)
https://www.nature.com/articles/s41467-018-06959-8
All the best,
Min