You are here
Journal Club for August 2020: Mechanics of High-capacity Rechargeable Batteries
Mechanics of High-capacity Rechargeable Batteries
Cole Fincher, Yuwei Zhang, Matt Pharr
Department of Mechanical Engineering, Texas A&M University
1. Introduction
Rechargeable batteries are ubiquitous in everyday life. Li-based batteries have become the power source of choice in portable electronics and electric vehicles [1]. Distributing batteries into a connected grid would enable energy storage from renewable resources. Still, commercial batteries utilize materials with relatively low energy densities; batteries add substantial weight to vehicles and occupy huge volume in portable electronics but must be re-charged every few hours [2]. Fortunately, several battery chemistries with tremendous theoretical storage capacities have emerged, including batteries “beyond Li-ion” based on metallic Li & Na, that are primed to meet these growing demands [3-6]. However, these systems suffer from severe issues of cyclability and safety that have precluded practical use. While the electrochemistry of these systems has received extensive study, at the heart of the issue lies a mechanics of materials problem. Specifically, as atoms move and rearrange under electrochemical driving forces, the material deforms, thereby generating stresses under constraint. These stresses can result in fracture, delamination, and/or unstable deformation of the electrodes, diminishing their capacity. Such mechanical degradation has precluded the practical deployment of several high-capacity electrode materials.
Previous iMechanica journal club issues have also highlighted phenomena related to mechanics of rechargeable batteries: https://imechanica.org/node/9413, https://imechanica.org/node/19189, https://imechanica.org/node/20073, and https://imechanica.org/node/21788. In this issue, we will focus on recent experimental research from our group aimed at basic understanding of mechanical behavior of high-capacity battery electrodes.
2. Mechanics of High-capacity Cathodes
2.1 In-Situ Measurements of Stress Evolution in Composite Sulfur Cathodes [7]
Sulfur has emerged as a leading candidate to replace conventional cathodes primarily due to its tremendous capacity of 1672 mAh/g, which is an order of magnitude larger than existing transition-metal cathodes [8-9]. Additionally, sulfur is one of the most abundant materials in the earth’s crust and is environmentally benign and may thus provide a low cost and sustainable option for cathodes [9-10]. However, when sulfur reacts with lithium, intermediate lithium polysulfides form, which are soluble in most liquid electrolytes and can shuttle through them to the anode, resulting in loss of active material [8-9]. Additionally, Li2S is electronically insulating; a thick layer of Li2S can passivate the surface of the electrode, inhibiting further lithiation [11-12]. Furthermore, an enormous volume expansion (~80%) accompanies converting S to Li2S, which can lead to pulverization of the electrodes [8]. However, the mechanics of electrochemical cycling of sulfur is fundamentally distinct from other electrode materials due to solid-to-liquid, liquid-to-liquid, and liquid-to-solid phase transformations, and thus remains poorly understood.
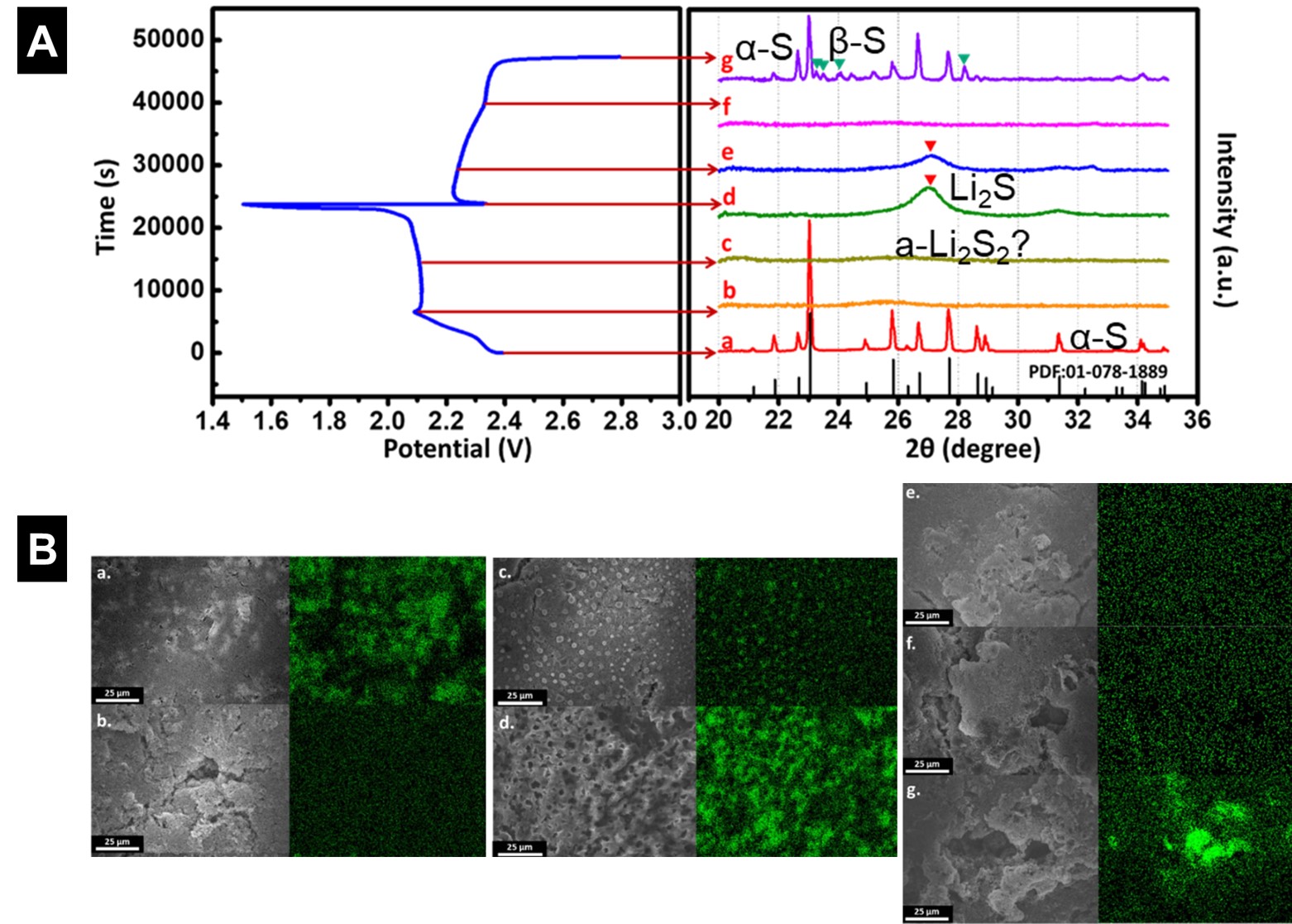
Figure 1. (A) Potential of a composite sulfur electrode during an electrochemical cycle and corresponding XRD patterns at the indicated potentials. (B) SEM and EDS images of a sulfur cathode at different states of charge, with the labels (a) - (g) corresponding to those in (A). The green color in the EDS images indicates the presence of sulfur. Adapted from Reference [7].
To provide insight into its mechanical behavior, we have used a multibeam optical stress sensor (MOSS) to measure the evolution of stresses in composite sulfur cathodes during electrochemical cycling, which we linked to structural evolution through complementary XRD and EDS [7]. The electrochemical and structural studies indicate a sequence of events (Figure 1): during lithiation, 1) solid-phase conversion of sulfur into electrolyte-soluble polysulfides (a-b), followed by 2) deposition of an amorphous solid phase, which ultimately converts to crystalline Li2S (b-d), and during de-lithiation: 3) dissolution of Li2S (d-f), followed by 4) re-deposition of two distinct phases of crystalline sulfur (f-g). Figure 2 shows the potential and corresponding stress response during the initial cycle of a composite sulfur cathode. In Region 1, the dissolution of solid sulfur removed residual stresses from fabrication and resting in the electrolyte (which were tensile here). Region 2 reflects stress evolution typical of thin film nucleation and growth processes. Namely, solid-phase island formation and coalescence (as seen in Figure 1) initially induced relative tension between points b and c. As more species were deposited (points c to d), compressive stresses were generated, likely stemming from: 1) incorporation of atoms from the electrolyte into the interface between solid LixS and the carbon matrix and/or 2) solid-phase conversion of Li2S2 to Li2S, which induces ~30% volumetric expansion. In Region 3 (de-lithiation), the dissolution of solid Li2S led to a near-linear relative increase in tensile stress from (d-e), which represents removal of the stresses from lithiation. During (e-f), lower order liquid polysulfides converted to higher order polysulfides, which had little effect on the stress since the phase changes occurred in the liquid state. In Region 4, as the higher order polysulfides began to deposit back onto the cathode as crystalline sulfur, relative tensile stresses initially occurred in the cathode (shortly after point (f)), followed by relative compression as the sulfur film was regenerated albeit with a different morphology and phase. In this sense, Region 4 can also be regarded as a thin film nucleation and growth process.
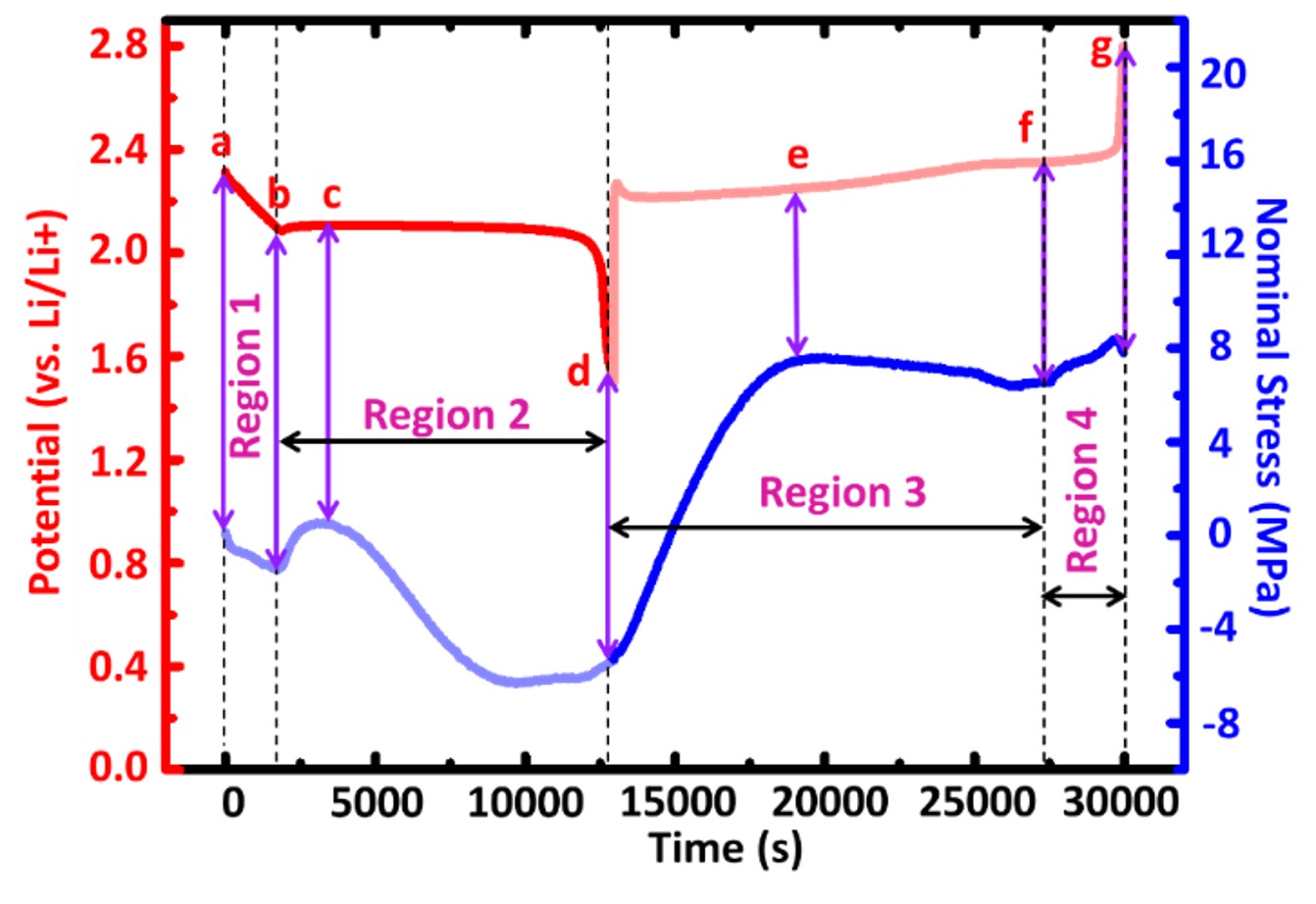
Figure 2. Potential and corresponding stress response during the initial cycle of a composite sulfur cathode [7].
Overall, the first cycle shows significant hysteresis from structural transformations and corresponding irreversible deformation. Subsequent cycling (details in [7]) led to more reversible mechanics. As a result, electrodes that withstand the first cycle while retaining active species, which can be engineered through precise mesoscale texturing, hold tremendous promise for structurally robust sulfur-based cathodes. Going forward, we hope these studies will provide fundamental insight into the practical design of mechanically robust sulfur cathodes as well as inspire modeling of coupled electrochemistry, phase transformations, and mechanics.
2.2 Chemo-Mechanical Degradation in V2O5 Thin Film Cathodes [13]
Vanadium oxide is a promising material for next-generation cathodes, with a large theoretical capacity of 442 mAh/g, as it can host up to 3 Li atoms per formula unit (V2O5) [14]. Moreover, V2O5 can be stabilized as different polymorphs with varying atomic connectivities [14-16]. Indeed, recent studies have suggested that several polymorphs of V2O5 are ideal candidates for hosting multivalent metal-ions with large volumes while maintaining excellent electrochemical performance [17-18]. However, the extent of reversible intercalation in this system has been found to be much lower than theoretical predictions, which likely stems from phase transformations and the corresponding structural/mechanical degradation.
To investigate these effects, we have performed in-situ MOSS measurements of stress that develop in V2O5 thin films during electrochemical cycling, Figure 3A [13]. In doing so, we devised an approach to fabricate dense V2O5 thin films without binders or conductive additives, which allows us to scrutinize the root cause of capacity fade in V2O5 cathodes of Li-ion batteries. During deep discharge (4.0 – 2.0 V vs Li/Li+), V2O5 undergoes several phase transformations, as indicated by the varying colored background regions in Figure 3B, which induce varying levels of stress. The first transformation (α-V205 → ε-LixV2O5) induces compressive stresses in the film due to the volumetric expansion associated with the transformation and the constraint provided by the substrate. The second transformation (ε-LixV2O5 → δ-LixV2O5) induces further compression and at an increased rate due to the even more significant volumetric expansion per Li added. The final transformation studied (δ-LixV2O5 → γ-LixV2O5) interestingly initially induces relative compression in the film, followed by relative tension upon further lithiation. The relative tensile stress associated with this phase transformation arises from relative volume contraction with lithiation that stems from an orthogonal rotation of two square-pyramidal VO5 units in opposite directions, despite additional lithium insertion. De-lithiation initially induces tension, followed by a nearly flat stress profile. The residual stresses remaining after a complete cycle are indicative of plastic deformation, thereby attesting to the irreversibility of the transformation to the γ-LixV2O5 phase.
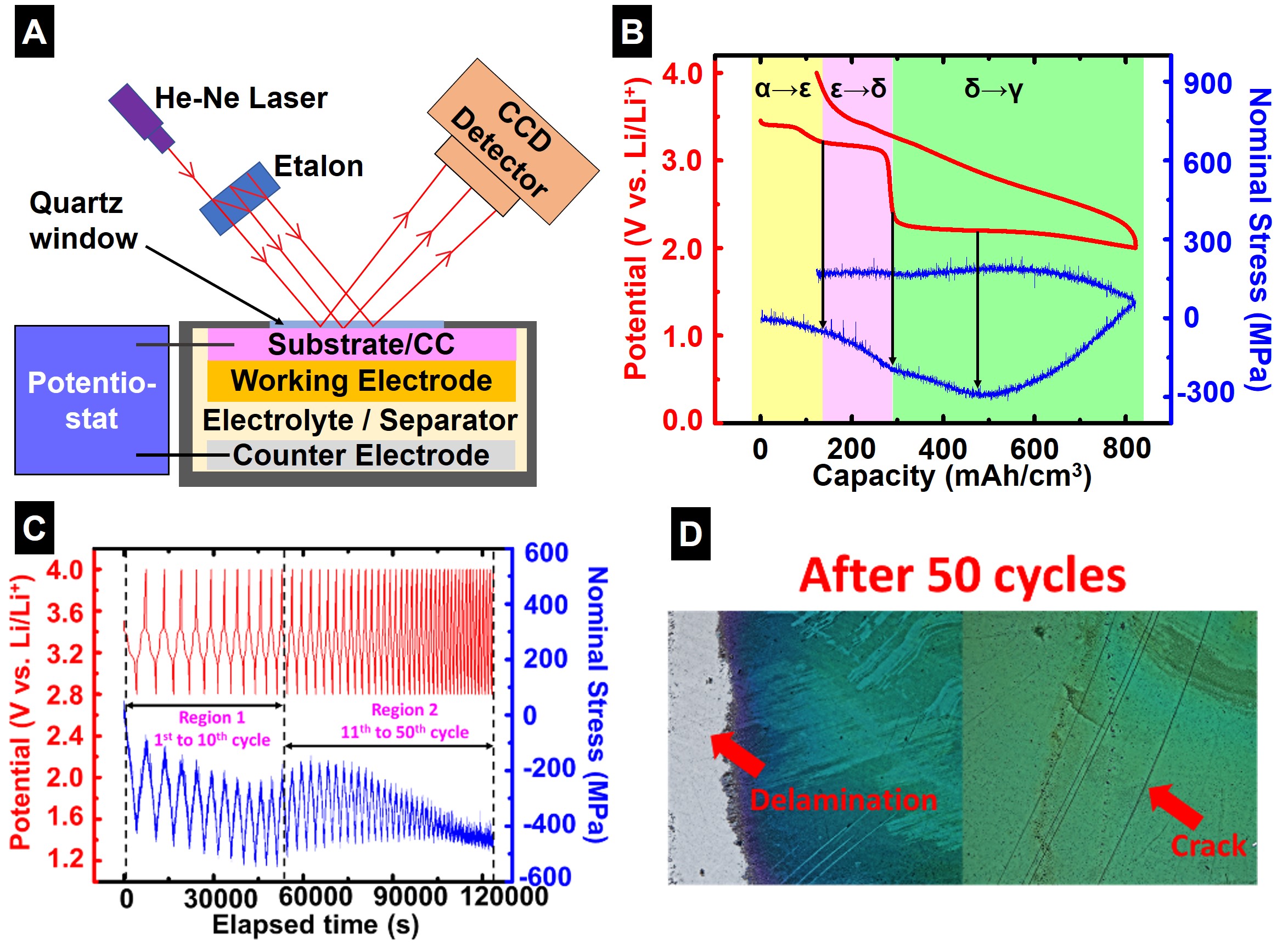
Figure 3. (A) A schematic of a typical experimental setup, which employs a laser split through an etalon, a CCD detector, a potentiostat, and an electrochemical cell with a quartz window for optical access. Potential and corresponding stress response during (B) a deep discharge of a V2O5 thin film electrode between 4.0 – 2.0 V vs. Li/Li+ at 0.2 C and (C) a relatively shallow discharge of a V2O5 thin film electrode between 4.0 – 2.8 V vs. Li/Li+ at 0.2 C over 50 cycles. (D) Optical microscopy images after the 50 cycles shown in part (C). Adapted from Reference [13].
By comparison, relatively shallow discharge (4.0 - 2.8 V vs Li/Li+) induces primarily elastic deformation (Figure 3C). However, the compressive stresses gradually increase with cycle number, likely due to side reactions and/or residual Li left in the V2O5, even after delithiation. Further cycling leads to accumulated mechanical damage (e.g., fracture, delamination) and structural damage (e.g., amorphization), which ultimately result in severe capacity fade (Figure 3D). Overall, despite the relatively small volume changes in cathodes during cycling (a few percent), the observations here highlight the intimate coupling between electrochemistry and mechanics in cathodes of lithium-ion batteries.
3. Mechanics of High-capacity Metallic Anodes
3.1 Mechanical Properties of Metallic Lithium: from Nano to Bulk Scales [19]
Lithium metal is known as the “Holy Grail” of anode materials for Li batteries, as it has the highest theoretical capacity (3860 mAh/g), lowest density, and most negative electrochemical potential (-3.04 V vs. the SHE) [20]. State-of-the-art Li-ion batteries have practical energy densities of ~250 Wh/kg but transitioning to systems based on Li anodes would drastically increase these capacities, e.g., Li-S systems could produce ~650 Wh/kg, and Li-air batteries could produce ~950 Wh/kg [20]. However, growth of Li dendrites can produce short-circuits and induce explosion hazards [21-22]. Additionally, reduction of essentially all electrolytes occurs readily at Li’s surface, producing a solid electrolyte interphase (SEI), which consumes active material [20-21]. Finally, Li metal suffers from so-called “infinite volume change” due to its host-less nature (i.e., it involves a plating process) [20]. Cycling exacerbates degradation, producing porous Li electrodes, thick accumulated SEI, Li dendrites, and electronically isolated, aka “dead,” Li, resulting in capacity fading and safety hazards that must be addressed prior to practical implementation [20]. Notably, a few studies have indicated that mechanics plays a key role in the formation/suppression of dendrites, including modeling work by Newman and Monroe [23], Jana and Garcia [24], and Narayan and Anand [25]. Experimental studies have shown that the morphology of Li during electrochemical deposition depends on external pressures applied to battery stacks [26], as does the propensity for maintaining interfacial contact in all-solid-state batteries [27]. Despite the significant mechanical issues outlined above, relatively little is known regarding even the basic mechanical behavior/properties of Li metal itself. Studies in tension by Tariq et al. [28], followed recently by Masias et al. [29] and LePage et al. [30] indicate ductile and rate-dependent behavior of bulk Li. Studies under compression at the nano [31], micro [32], and bulk [33] scales find yield strengths (or hardness/3 values) of ~100, 10, and 1 MPa, respectively. Directly comparing these results is somewhat tricky, given the studies’ various loading conditions and methods of sample preparation.
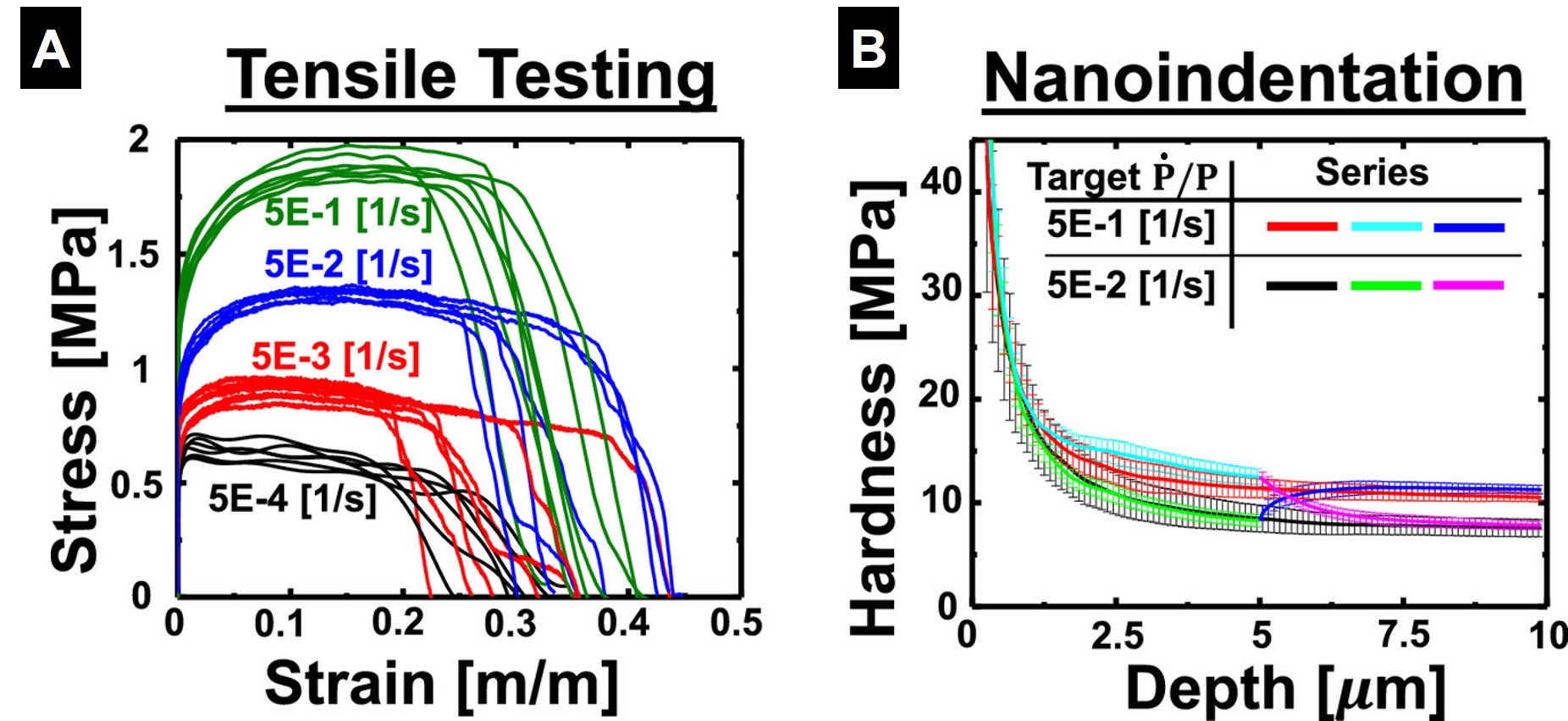
Figure 4. (A) Stress-strain relationship from uniaxial tension testing of as-received bulk lithium metal at various strain rates. (B) Nanoindentation hardness as a function of depth, with each series representing the average of 7 individual indents conducted under identical test conditions. Scatter bars span one standard deviation from the mean [19].
To this end, through a combination of bulk tensile testing, nanoindentation, and other data reported in the literature, we have performed a comprehensive assessment of the strain-rate and length-scale dependent mechanical properties of Li in its most commonly used form: high purity commercial foil [19]. Performing tests in inert environments (Ar), we find that bulk Li exhibits a yield strength between 0.57 and 1.26 MPa for strain rates from 0.05%/s to 50%/s (Figure 4A). Through nanoindentation tests (at P·/P=0.05 1/s), the hardness of lithium decreases precipitously from nearly 43 MPa at depths of 250 nm to values of 7.5 MPa as the depth approaches 10 μm (Figure 4B). A comprehensive comparison of measured hardness and or yield strengths from this study and literature are provided in Figure 5 as a function of the relevant length scale associated with the mechanical test. The plastic properties measured from both our bulk and nanoindentation testing exhibit strong strain-rate dependencies, with stress exponents of n = 6.55 and 6.9, respectively (in the form ϵ·=Aσ^n). The measured yield stresses provide representative values of stresses that can be applied to Li electrodeposits prior to permanent deformation (i.e., flattening). As such, the results serve as a basis to estimate the stack pressure required to blunt electrodeposits with the goal of improving performance. Additionally, the rate-sensitivity of lithium metal suggests that deformation mechanics may play a role in dictating the dendrite morphology, e.g., dendrite morphology depends significantly on current density, i.e., it depends on deposition/strain rate. Li’s size dependent properties may provide a means of tuning the deformation mechanics, and thus perhaps the robustness of the Li anode by adjusting the Li deposit sizes through design of 3D current collectors and seeding the deposition of Li, among other methods. As such, these studies may help to guide the design of battery architectures and charging conditions to mitigate unstable growth of Li during electrochemical cycling [19].
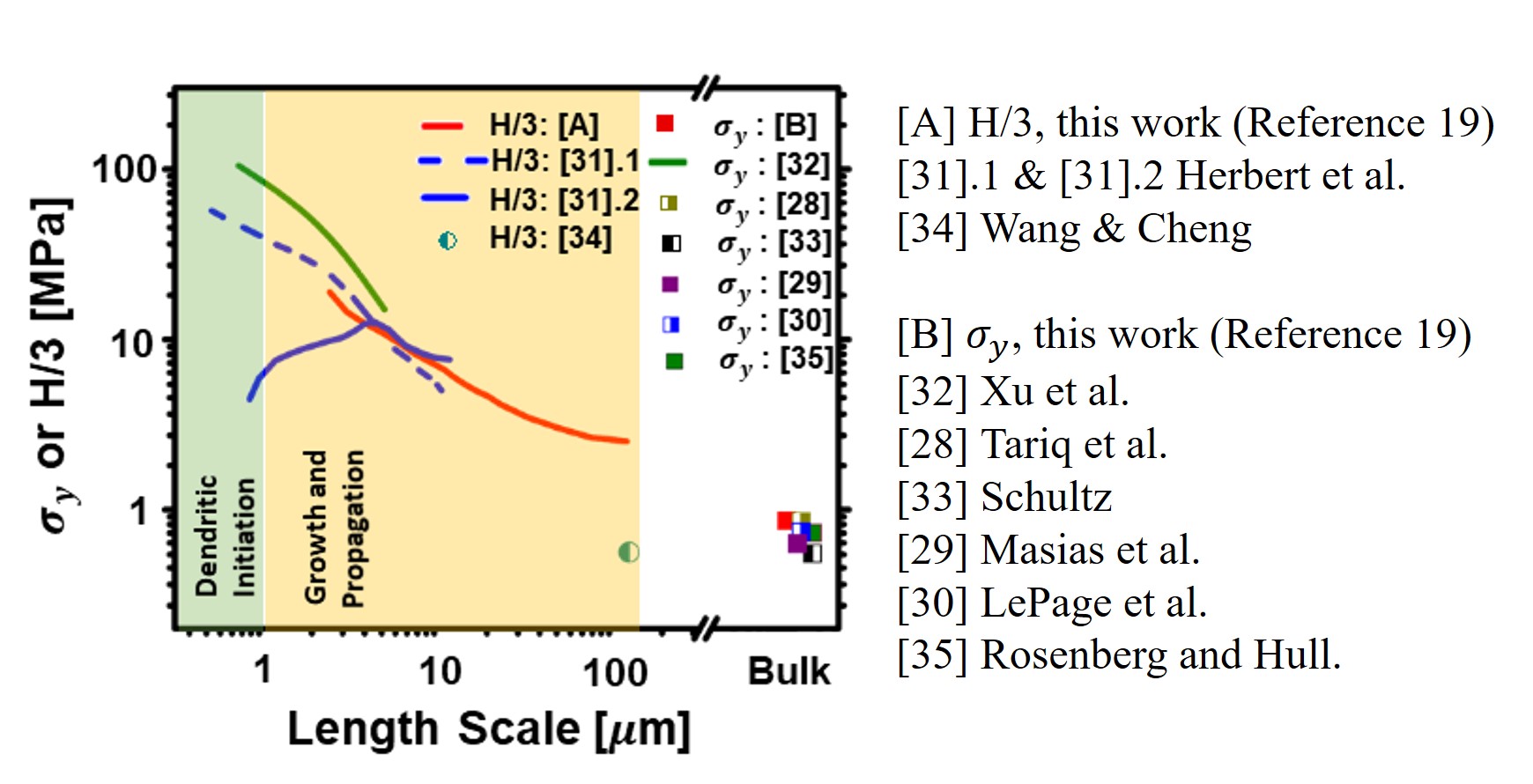
Figure 5. The σy or H/3 of Li is plotted versus a representative length scale, displaying data from this study as well as previous literature. Feature sizes corresponding to dendritic initiation are shown as a green background, while the yellow region corresponds to dendritic growth and propagation. Adapted from [19].
3.2 Elastic and Plastic Characteristics of Sodium Metal [36]
Sodium-based batteries have garnered recent attention largely due to Na’s earth abundance, low cost, and limited geographic constraints [37-38]. As such, they may find applications where Li-based batteries are not as viable, such as in grid-scale energy storage from renewable resources. Of potential candidates for Na-based anodes, Na metal itself has the most promise, as it is earth abundant, has the lowest electrochemical potential (-2.71 V vs. SHE), and has the highest theoretical capacity (1165 mAh/g) [37-38]. However, concerns mirror that of Li metal: SEI forms unstably; “infinite volume change” occurs; and unstable (dendritic) morphologies and microstructures can form, all of which lead to severe capacity fade and safety hazards [37-38]. Given the importance of mechanical deformation in this process, unlocking the potential of sodium metal as an anode material requires a thorough understanding of its mechanical properties, which dictate the stress sustained by the electrode (and the surrounding separator or solid electrolyte) under various geometries and loading conditions, e.g., at different charging rates. The stresses in turn may affect the electrode kinetics, the growth morphology under cycling, and/or the integrity of the contact at the anode/solid-state-electrolyte (SSE) interface. Strategies to mitigate these cycling issues will require a thorough understanding of Na’s mechanical properties.

Figure 6. (A) Stress-strain relationship for Na under bulk compression at several loading rates. (B) Nanoindentation tests of Na, with each series representing the average of 7 or more individual indents conducted under identical test conditions.
To this end, we have evaluated the mechanical properties of sodium metal at room temperature through a combination of bulk compression, microhardness, and nanoindentation tests [36]. Regarding elastic properties, nanoindentation testing produced an elastic modulus of 3.9±0.5 GPa. Regarding plastic properties, bulk compression testing revealed the flow stress at 8% strain as varying between 102 and 254 kPa at strain-rates between 0.01 - 1%/s (Figure 6A). Nanoindentation indicated a decrease in hardness from 26.6 to 2.3 MPa (at P·/P=0.05 1/s) as the indentation depth increased from 0.25 to 10 μm (Figure 6B), while microhardness testing indicated hardness values between 1.6 and 1.1 MPa at depths varying between 50 and 130 μm. A comparison of Li and Na metal in terms of strength/hardness as a function of representative length scale is provided in Figure 7A. We also found that Na exhibits a marked strain-rate sensitivity, with a strain-rate sensitivity exponent of m = 0.14 from nanoindentation and m = 0.20 from bulk compression (in the form σ=κϵ⋅^m). Likewise, nanoindentation demonstrated that Na is even more susceptible to creep than is Li metal (Figure 7B). Overall, our studies indicate that Na metal is extremely soft, readily creeps, and exhibits pronounced size effects. The soft nature of Na metal may play a positive role in maintaining uniform deposit morphology and in maintaining anode/SSE contact. However, Na’s extremely soft and creep-prone behavior may also exacerbate other failure modes, e.g., leading to the transport of Na into SSE grain boundaries, which can precipitate fracture of the SSE [36].

Figure 7. (A) σf or H/3 of Li and Na versus the representative length scale from the mechanical test. (B) Nanoindentation load-depth curves of both Li and Na conducted at a target P·/P=0.05 1/s to a depth of 5 μm, followed by a 30 minute load hold. The indenter tip drifts forward ~70% farther during the load hold in the sodium as compared to the lithium, despite the sodium hold occuring at a lower load. Adapted from [19] and [36].
4. References
1. Nazri, G. A.; Pistoia, G. Lithium Batteries. 1 ed.; Springer US: 2003; p 708.
2. Buchmann, I. Bu-1003: Electric Vehicle (Ev) 2016. http://batteryuniversity.com/learn/article/electric_vehicle_ev (accessed 7/22/2020).
3. Goodenough, J. B.; Park, K.-S. “The Li-Ion Rechargeable Battery: A Perspective.” Journal of the American Chemical Society 135 (4), 1167-1176 (2013).
4. Bruce, P. G.; Freunberger, S. A.; Hardwick, L. J.; Tarascon, J.-M. “Li-O2 and Li-S Batteries with High Energy Storage.” Nature Materials 11 (1), 19-29 (2012).
5. Pan, H.; Hu, Y.-S.; Chen, L. “Room-Temperature Stationary Sodium-Ion Batteries for Large-Scale Electric Energy Storage.” Energy & Environmental Science 6 (8), 2338-2360 (2013).
6. Zheng, X.; Bommier, C.; Luo, W.; Jiang, L.; Hao, Y.; Huang, Y. “Sodium Metal Anodes for Room-Temperature Sodium-Ion Batteries: Applications, Challenges and Solutions.” Energy Storage Materials 16, 6-23 (2019).
7. Zhang, Y.; Luo, Y.; Fincher, C.; McProuty, S.; Swenson, G.; Banerjee, S.; Pharr, M. “In-Situ Measurements of Stress Evolution in Composite Sulfur Cathodes.” Energy Storage Materials 16, 491-497 (2019).
8. Manthiram, A.; Fu, Y.; Chung, S.-H.; Zu, C.; Su, Y.-S. “Rechargeable Lithium–Sulfur Batteries.” Chemical Reviews 114 (23), 11751-11787 (2014).
9. Wang, D.-W.; Zeng, Q.; Zhou, G.; Yin, L.; Li, F.; Cheng, H.-M.; Gentle, I. R.; Lu, G. Q. M. “Carbon-Sulfur Composites for Li-S Batteries: Status and Prospects.” Journal of Materials Chemistry A 1 (33), 9382-9394 (2013).
10. Bruce, P. G.; Freunberger, S. A.; Hardwick, L. J.; Tarascon, J.-M. “Li-O2 and Li-S Batteries with High Energy Storage.” Nature Materials 11 (1), 19-29 (2012).
11. Rauh, R. D.; Abraham, K. M.; Pearson, G. F.; Surprenant, J. K.; Brummer, S. B. “A Lithium/Dissolved Sulfur Battery with an Organic Electrolyte.” Journal of The Electrochemical Society 126 (4), 523-527 (1979).
12. Yamin, H.; Peled, E. “Electrochemistry of a Nonaqueous Lithium/Sulfur Cell.” Journal of Power Sources 9 (3), 281-287 (1983).
13. Zhang, Y.; Luo, Y.; Fincher, C.; Banerjee; Pharr, M. “Chemo-Mechanical Degradation in V2O5 Thin Film Cathodes of Li-ion Batteries during Electrochemical Cycling.” Journal of Materials Chemistry A 7, 23922-23930 (2019).
14. Yue, Y.; Liang, H. “Micro- and Nano-Structured Vanadium Pentoxide (V2O5) for Electrodes of Lithium-Ion Batteries.” Advanced Energy Materials 7, 1–32 (2017).
15. Horrocks, G. A.; Likely, M.F.; Velazquez, J.M.; Banerjee, S. “Finite size effects on the structural progression induced by lithiation of V2O5: a combined diffraction and Raman spectroscopy study.” Journal of Materials Chemistry A 1, 15265–15277 (2013).
16. De Jesus, L. R.; Andrews, J. L.; Parija, A.; Banerjee, S. “Defining Diffusion Pathways in Intercalation Cathode Materials: Some Lessons from V2O5 on Directing Cation Traffic.” ACS Energy Letters 3, 915–931 (2018).
17. Parija, A.; Liang, Y.; Andrews, J.; De Jesus, L.R.; Prendergast, D.; Banerjee, S. “Topochemically De-Intercalated Phases of V2O5 as Cathode Materials for Multivalent Intercalation Batteries: A First-Principles Evaluation.” Chemistry of Materials 28, 5611–5620 (2016).
18. Kundu, D.; Adams, B. D.; Duffort, V.; Vajargah, S. H.; Nazar, L. F. “A high-capacity and long-life aqueous rechargeable zinc battery using a metal oxide intercalation cathode.” Nature Energy 1, 1–8 (2016).
19. Fincher, C.; Ojeda, D.; Zhang, Y.; Pharr, G.; Pharr, M. “Mechanical Properties of Metallic Lithium: from Nano to Bulk Scales.” Acta Materialia 186, 215-222 (2020).
20. Lin, D.; Liu, Y.; Cui, Y. “Reviving the Lithium Metal Anode for High-Energy Batteries.” Nature Nanotechnology 12 (3), 194-206 (2017).
21. Xu, W.; Wang, J.; Ding, F.; Chen, X.; Nasybulin, E.; Zhang, Y.; Zhang, J.-G. “Lithium Metal Anodes for Rechargeable Batteries.” Energy & Environmental Science 7 (2), 513-537 (2014).
22. Whittingham, M. S. “Lithium Batteries and Cathode Materials.” Chemical Reviews 104 (10), 4271-4302 (2004).
23. Monroe, C.; J. Newman. “The impact of elastic deformation on deposition kinetics at lithium/polymer interfaces.” Journal of The Electrochemical Society 152 (2), A396-A404 (2005).
24. Jana, A.; García R.E. “Lithium dendrite growth mechanisms in liquid electrolytes.” Nano Energy 41, 552-565 (2017).
25. Narayan, S.; Anand, L. “A Large Deformation Elastic–Viscoplastic Model for Lithium.” Extreme Mechanics Letters 24, 21-29 (2018).
26. Gireaud, L.; Grugeon, S.; Laurelle, S.; Yrieix B.; Tarason, J. M., “Lithium metal stripping/plating mechanisms studies: A metallurgical approach.” Electrochemistry Communications 8 (10), 1639-1649 (2006).
27. Luntz, A. C.; Voss J.; Reuter, K. “Interfacial challenges in solid-state Li ion batteries.” The Journal of Physical Chemistry Letters 6 (22), 4599-604 (2015).
28. Tariq, S.; Ammigan, K.; Hurh, P.; Schultz, R.; Liu, P.; Shang, J. “Li Material Testing - Fermilab Antiproton Source Lithium Collection Lens.” Proceedings of the 2003 Particle Accelerator Conference 3, 1452-1454 (2003).
29. Masias, A.; Felten, N.; Garcia-Mendez, R.; Wolfenstine, J.; Sakamoto, J. “Elastic, Plastic, and Creep Mechanical Properties of Lithium Metal.” Journal of Materials Science 54 (3), 2585-2600 (2019).
30. LePage, W. S.; Chen, Y.; Kazyak, E.; Chen, K.-H.; Sanchez, A. J.; Poli, A.; Arruda, E. M.; Thouless, M. D.; Dasgupta, N. P. “Lithium Mechanics: Roles of Strain Rate and Temperature and Implications for Lithium Metal Batteries.” Journal of The Electrochemical Society 166 (2), A89-A97 (2019).
31. Herbert, E. G.; Hackney, S. A.; Thole, V.; Dudney, N. J.; Phani, P. S. “Nanoindentation of High-Purity Vapor Deposited Lithium Films: A Mechanistic Rationalization of Diffusion-Mediated Flow.” Journal of Materials Research 33 (10), 1347-1360 (2018).
32. Xu, C.; Ahmad, Z.; Aryanfar, A.; Viswanathan, V.; Greer, J. R. “Enhanced Strength and Temperature Dependence of Mechanical Properties of Li at Small Scales and Its Implications for Li Metal Anodes.” Proceedings of the National Academy of Sciences 114 (1), 57-61 (2017).
33. Schultz, R. P. “Lithium: Measurement of Young's Modulus and Yield Strength.” Fermi National Accelerator Lab., Batavia, IL (2002).
34. Wang, Y.; Cheng, Y. T. “A nanoindentation study of the viscoplastic behavior of pure lithium.” Scripta Materialia 130, 191-195 (2017).
35. Hull, D.; Rosenberg, H. M. “The Deformation of Lithium, Sodium, and Potassium at Low Temperatures: Tensile and Resistivity Experiments.” The Philosophical Magazine: A Journal of Theoretical Experimental and Applied Physics 4 (39), 303-315 (1959).
36. Fincher, C.; Zhang, Y.; Pharr, G.; Pharr, M. “Elastic and Plastic Characteristics of Sodium Metal.” ACS Applied Energy Materials 3, 1759-1767 (2020).
37. Pan, H.; Hu, Y.-S.; Chen, L. “Room-Temperature Stationary Sodium-Ion Batteries for Large-Scale Electric Energy Storage.” Energy & Environmental Science 6 (8), 2338-2360 (2013).
38. Zheng, X.; Bommier, C.; Luo, W.; Jiang, L.; Hao, Y.; Huang, Y. “Sodium Metal Anodes for Room-Temperature Sodium-Ion Batteries: Applications, Challenges and Solutions.” Energy Storage Materials 16, 6-23 (2019).
| Attachment | Size |
|---|---|
| 332.37 KB | |
| 150.1 KB | |
| 668.78 KB | |
| 289.13 KB | |
| 200.82 KB | |
| 256.28 KB | |
| 163.56 KB |
- Matt Pharr's blog
- Log in or register to post comments
- 21006 reads


Comments
I can't see these images. How about other people?
Might a problem with me, but I checked twice since yesterday. Thanks.
Same with me .... Can't see images
Images not visible
Sorry about that; I have re
Sorry about that; I have re-uploaded. They should now display and are also attached.
Stress at the interface of electrode and current-collector
Dear Matt,
Thanks for the outstanding JClub post. I learned a lot.
We studied the utilization of graphene as a van der Waals (vdW) slippery interfaces to enhance the electrochemical stability of silicon film anodes in lithium-ion batteries.
https://www.dropbox.com/s/cjw85mg4d4isyrx/2D_SlipperySurface_ACS_AMI.pdf...
Si undergoes volume expansion (contraction) under lithiation (delithiation) of up to 300%. This large volume expansion leads to stress build-up at the interface between the Si film and the current collector, leading to delamination of Si from the surface of the current collector. We showed that by coating the current collector surface with graphene sheets, we could engineer a vdW slippery interface between the Si film and the current collector. For such an interface, the Si film slips with respect to the current collector under lithiation/delithiation, while retaining electrical contact with the current collector. Computational results indicate (i) less stress build-up and (ii) less stress "cycling" on a vdW slippery substrate as opposed to a fixed surface. Experimental results by our collaborators confirm more stable performance and much higher Coulombic efficiency for Si films on graphene-coated nickel (i.e., slippery surface) as compared to conventional nickel current collectors.
QUESTION :
Can this technique (using 2D materials as slippery surface) be useful for stress reduction of various electrodes you are studying, e.g., sulfur, V2O5?
Re: Stress at the interface of electrode and current-collector
Hi Dibakar,
Thanks for your comments and interesting paper. I believe your type of approach is likely applicable to many high-capacity electrodes that exhibit large volume changes during electrochemical cycling. The main issues as you point out are (1) whether the interface engenders sliding/slipping and (2) whether we can maintain good (electrical) contact as the active material slips/slides. Graphene seems a natural place to start as the coating layer; MD and DFT simulations such as yours are excellent tools for assessing other potential materials for this purpose to pair a given current collector to a given electrode material. We would be happy to assess any potential candidates experimentally :).
size effect
In our experience graphene is beneficial for half cell and thin electrodes, but seems of limited use for full cell and thick electrodes, here is a paper FYI: https://www.sciencedirect.com/science/article/pii/S2405829719308797
The strong size effect on their plasticity of Li and Na is intriguing. @Matt, do you know of other materials behave similarly? do we expect that this trend can be extrapolated to the nanometer scale, so the property applies to Li dendrites at early stage? Thank you!
Re: size effect
Thanks for your question, Kejie. The origin of the observed size effects in Li and Na is an open question, which warrants future microstructural studies aimed at determining deformation mechanisms. Generally speaking, it could be related to some characteristic microstructural feature size (e.g., grain size, dislocation spacing, precipitate size, etc.). It is also possible that some (but likely not all, according to some unpublished work we are pursuing) of this size effect stems from surface contamination, even when fresh surfaces are prepared in a glovebox.
In terms of extrapolating to dendrites, we do think these observations are relevant and potentially quite important, although there are some subtleties there. First, I would not recommend extrapolating below any dimension to which we (or others) have established that the instrument, sample prep., etc. will provide meaningful data. In our experiments, we assess through a few mechanisms, particularly by ensuring that the phase shift between the force and displacement oscillations in the continuous stiffness measurement technique is relatively small. Through this procedure, we ensure that the so-called plasticity error is low. (Note that if you are not careful, the plasticity error can easily become large for these materials due to their large E/H ratio, which will lead to erroneous data). Second, the dendrite itself may have solid electrolyte interphase (SEI) on it, which could change its overall mechanical response. That being said, dendrites initiate at a small size (tip radii are often observed as ranging between 0.1 to 1.5 microns) and typically grow to sizes on the order of microns. As such, we do think that our measurements will provide meaningful data and insight at a size range relevant to real batteries.
In terms of size effects in other materials, some good references are here:
https://doi.org/10.1146/annurev-matsci-070909-104456
https://doi.org/10.1016/j.pmatsci.2011.01.005
Kinematic hardening analysis of Li-ion battery
Dear Prof. Pharr,
Thank you for the post. Kinematic hardening analysis of Li-ion battery with concentration-dependent material behaviours under cyclic charging and discharging has been studied by us. Your test results will help us to improve our model to consider the viscoplastic behaviour.
Our paper can be read and commented and its address is:
https://www.sciencedirect.com/science/article/abs/pii/S0378775320304584
With Best Regards
Nayebi
Re: Kinematic hardening analysis of Li-ion battery
Hi Nayebi,
Very nice work; thanks!
Degradation of sulfur electrodes
Hi Matt, thanks a lot for this thorough and informative review! I have one question about the competition of mechanics against chemistry: For sulfur electrodes, the high stress built up during cycling may fracture the electrodes mechanically; and lithium polysulfides can dissolve in many liquid electrolytes, thereby failing the electrodes chemically. Between these two degradation mechanisms, which one is more detrimental to the durability of sulfur electrodes?
Re: Degradation of sulfur electrodes
Hi Zheng,
Thanks for your question. The main problems that have limited practical implementation of sulfur are chemical in nature: dissolution of polysulfides and their shuttling to the anode. This process consumes active material, which leads to capacity fade. Recent studies have begun to mitigate these issues, e.g., by incorporating blocking/confining/trapping-type materials and geometries to limit polysulfide shuttling. As such, S has picked up steam as a viable high-capacity cathode material. Still, the mechanics of this system remains relatively understudied, perhaps because the chemical problems were so severe or because of complicated liquid-solid, liquid-liquid, and solid-liquid phase transformations. Our studies indicate that stresses indeed build up during these phase transformations, which could potentially lead to damage. For instance, stresses during deposition and growth could drive delamination of Li2S from the current collector scaffolds. In doing so, active materials would be consumed, i.e., Li2S would form irreversibly in this scenario, as it would be electronically isolated from the system. To what extent these potential mechanical issues come into play in practical systems is an open question.