You are here
Journal Club for 15 June 2008: Kinetics of Structural Phase Transformations
A structural phase transformation is the transformation of a crystal structure due to the displacement of atoms, with no diffusion and no changes in the relative positions. These transformations are often induced by changes in temperature; however stress, electromagnetic fields and other loads can also induce transformations. The schematic below shows a cubic to tetragonal transformation. The crystal is cubic at high temperature and tetragonal at low temperature. Due to the symmetry of the cube stretching along any of its axes, there are 3 possible variants or twins at low temperature.

The phenomenon of twinning is closely related to structural phase transformations. A twin plane is an interface with different twins on either side of the interface. Twinning can compete with plasticity in some settings. The schematic below shows twinning of 2 tetragonal twins
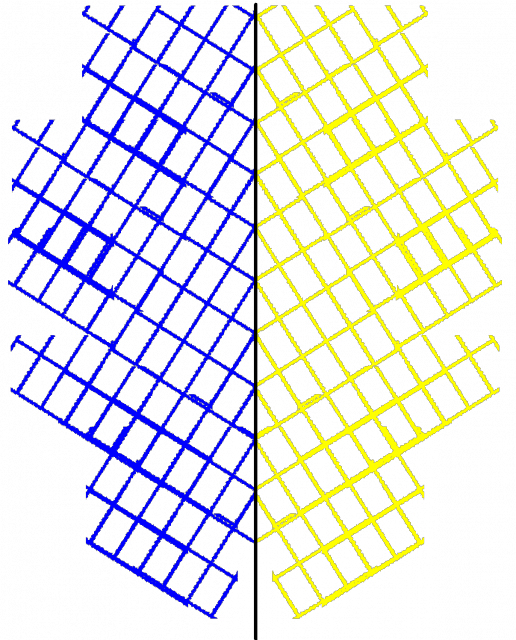
By considering the different twins as a distinct phases, the concepts and methods developed for phase transformations can be applied with minor modifications to twin plane motion. Hence, in the continuum mechanics literature, the term phase transformation often includes twinning.
A common example of a phase transformation is in iron: it has a body-centered cubic (BCC) structure at room temperature, called ferrite, that transforms to face-centered cubic (FCC) austenite structure above 1200K. The iron-carbon phase diagram is full of interesting examples. Structural transformations and twin motion also drive the interesting behavior of shape-memory alloys (coupling deformation and temperature) and ferroelectrics (coupling deformation and electric fields - the recent summary by Chad Landis provides more information).
Send a blank email to temporary.for.imechanica@gmail.com if you need a PDF of any of these papers.
1. Equilibrium of bars, by J. L. Ericksen. Journal of Elasticity, 5(3-4), 1975.
This paper by Ericksen first applied continuum elasticity to the modeling of phase transformations. In the process, it also exposed the inadequacy of continuum theory in describing such phenomena.
Prior to this work, the Landau-Ginzburg framework had been applied to phase transformations of various types (such as superconductivity). However, these did not deal with structural phase transformations that carefully considered deformations. Further, this framework typically had been applied to uniformly transforming specimens, not considering the phase transformation process as being at different stages in different regions, ie, a field problem. In this paper, Ericksen introduces the idea of modeling structural phase transformations using continuum mechanics, and, in the process, showed that classical continuum mechanics is inadequate to describe such phenomena.
The outline of this paper is as follows. In a one-dimensional bar, a strain-energy density with 2 minima is studied. Each minimum corresponds to a different crystal structure. For certain values of the stress, both phases can coexist in the bar with interfaces (phase boundaries) separating them. However, the positions and numbers of these interfaces are not predictable as an outcome of the balance laws of continuum mechanics. This is a surprising result; typical materials modeled in classical elasticity have convex strain energy and have unique solutions for the simple BCs that Ericksen considers.
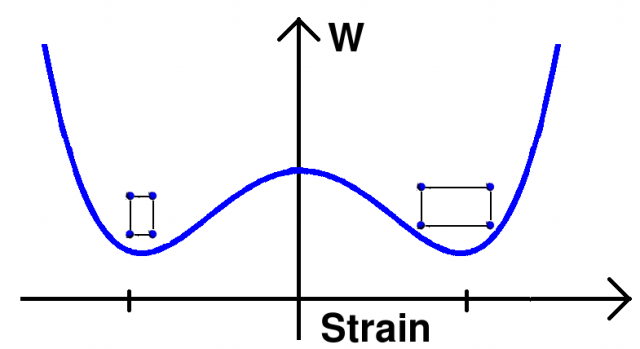
![]()
In equilibrium, this non-uniqueness can be resolved by going beyond the balance laws and further requiring that the system be at an absolute energy minimum (similar to the Maxwell construction in Van der Walls fluids). However, when elastodynamics is used to model the kinetics of phase transformations, the situation is much more complex.
Work by Abeyaratne and Knowles and others showed that further material information is required, beyond that contained in the strain energy. That is, given a continuum elastodynamics problem with non-convex strain energy, the solutions may contain interfaces, and the velocity and number of these interfaces is not unique; one possible way of specifying this material information to precisely achieve uniqueness is to add the kinetic relation for the phase boundaries (how fast interfaces move) and the nucleation criterion (when new phase boundaries nucleate). This viewpoint is the subject of the recent book by Abeyaratne and Knowles, and the key issues are discussed in Sections 4 to 6 here.
A major focus in recent years is to extract the kinetic relation for the phase boundary from atomic models, ie, to do for the kinetic relation what the Cauchy-Born rule does for strain energy density. Truskinovsky and Vainchtein study a 1D chain of atoms. The bond potentials are highly simplified, and this permits the use of analytical techniques. By using a traveling-wave form as an ansatz for a phase-boundary, the structure of a phase boundary as well as the kinetic relation (relating the velocity to the driving force) are found. In principle, such a procedure can provide input to the continuum model.
(preprint attached below)
The closed-form solutions of Truskinovsky and Vainchtein provide much insight. But their closed form comes at a cost; a key missing component is higher dimensionality. Hildebrand and Abeyaratne use MD techniques to examine the motion of a twin boundary in a 2D collection of atoms. The potentials are of Lennard-Jones type. While these are not as accurate as current potentials, they are simple and yet sufficiently capture a lot of interesting mechanics of the atomic motion. One observation is the motion of ledges or steps across the width of the phase boundary, rather than the phase boundary propagating as a uniform interface.
The kinetic relations that are calculated from MD provide a mesoscopic view of phase boundary motion, ie, it allows us to go from atoms to a continuum but still at a small scale. At this small scale, phase boundaries can interact with other defects in the material, for example inclusions. Bhattacharya studies a continuum phase boundary with a simple kinetic relation interacting with a large number of defects, and derives the effective kinetics that would be observed at much larger scales. One interesting feature that comes out of the analysis naturally is the stick-slip nature of the effective phase boundary motion.
| Attachment | Size |
|---|---|
| 1.62 MB |
- Kaushik Dayal's blog
- Log in or register to post comments
- 27178 reads


Comments
Tin Pest: An Important Phase Transition
Recent years, the electronics industry is shifting from SnPb solders (e.g., Sn63Pb37, Sn10Pb90, Sn5Pb95, etc.) into lead-free solders due to the environmental legislation issue. Most of the lead-free solders are high Sn solders (Sn96.5Ag3.0Cu0.5, Sn98.5Ag1.0Cu0.5, etc.). For high tin solder, there is a phase transition phenomenon called "Tin Pest". At temperatures of above room temperature, tin is a grey metal called beta-Sn. It has a body-centered-tetragonal structure. When temperature is lower than 13C, there is a spontaneous phase transition and the crystal structure changes into diamond cubic structure, which is called alpha-Sn. alpha-Sn is white and brittle. Most of the time, it is in powder or granular form. Unfortunately, the mechanism of the transition is not well understood. This makes the reliability of the Sn-rich solder unpredictable. I hope this iMechanica forum can stimulate more research on this topic. Here I am attaching a few references to start the discussion.
W.J. Plumbridge, “Recent Observations on Tin Pest Formation in Solder Alloys” Journal of Electronic Materials, Vol. 37, No. 2, pp218-223, 2008
O. Semenova, H. Flandorfer, H. Ipser, “On the non-occurrence of tin pest in tin–silver–indium solders”, Scripta Materialia, vol. 52, pp89–92, 2005
Y. Kariya, N. Williams, C. Gagg, and W. Plumbridge, “Tin Pest in Sn-0.5 wt.% Cu Lead-Free Solder”, JOM, June issue, pp 39-41, 2001.
Video: Tin Pest
Here's a video of the tin pest phenomenon (via Bainite ).
Nice video
Thanks to Biswajit for the nice video. It is really amazing to observe the metal transforms from one phase to another under microscope.
Tin is really a headache to the semiconductor industry. Tin pest is part of the story. The other is the tin whiskering. When tin is plated onto Cu lead-frames. It grows whiskers spontaneously. The whiskers are single crystal tin with diameter around a few microns. Their length might be in millimeters, which will cause the circuit to short. Again, there is no conclusive mechanism on the whiskering. As a starting point please see NASA's info page http://nepp.nasa.gov/WHISKER/background/index.htm and my post on iMechanica http://www.imechanica.org/node/226
Diffusion required?
Given the time scale in the video, it appears that diffusion of species is required for this transformation. Is this the case? If so, what are the details?
There could be some diffusion involved in this video
Chad,
Although the phase transition mechanism is not clear, literature points to the direction that impurities play an important role in beta-tin to alpha-tin transformation. Here is an excerpt from Plumbridge's JEM paper list above:
"The most notable, and challenging, aspect of tin pest in both pure tin and dilute tin alloys is the inconsistency in the findings, both within and between laboratories. Nominally identical specimens which have experienced identical treatments may exhibit quite different responses, as far as tin pest formation is concerned. In broad terms, elements soluble in tin, such as Pb, Sb, or Bi, tend to suppress the transition to a tin, whereas elements insoluble in tin, such as Zn, Al, or Mg, are likely to accelerate it, as does the presence of prior cold work. These should be regarded as general observations: the true complexity of the situation is described in greater detail in Ref. 1. The process itself involves nucleation and growth, and may take years or even decades to occur without the assistance of artificial nucleation by seeds of a tin. Nucleation criteria are unknown, and the role of impurities may be critical. This could account for the inconsistency reported so far."
Since the video mentioned that it was made using a chemical method to speed up the process, there might be some impurities which diffuse into the beta-tin to cause the phase transition.
Possible answer
This is a fascinating mechanism. After reading some of the papers you referenced, I think the answer is that it is "diffusion" but not of the type I thought. Alpha tin has an enormous volume change with respect to beta tin, such that the lattice parameter of the cubic alpha phase does not lie between the a and c parameters of the tetragonal beta phase. I believe that this implies, a la Bhattacharya and James, that there is no compatible phase boundary between the two phases. So in order to propagate the alpha phase into the beta phase you need the beta phase to creep. This is pure conjecture on my part, but it could explain the rather long time scales needed for the degradation to occur. You can tell me I'm crazy if you think so. I won't be offended.
Kinetics of Structural Phase Transformations
Dear all,
I would like to attract your attention to some books:
M.Pitteri and G.Zanzotto.
Continuum Models for Phase Transitions and Twinning in Crystals
Chapman & Hall/CRC, Boca Raton, 2003
R.Abeyaratne and J.K.Knowles. Evolution of Phase Transitions: A Continuum Theory, Cambridge University Press, 2006.
A.Berezovski, J.Engelbrecht, G.A.Maugin.
Numerical simulation of waves and fronts in inhomogeneous solids.
World Scientific, Singapore, 2008
http://www.worldscibooks.com/mathematics/6931.html
Thanks,
Arkadi Berezovski
Stress induced phase transformation
Thanks for the post. It is indeed a nice discussion. To well related to our mechanics, I think stress induced phase transformation is of great interest. This has potential applications in sensors and other functional devices. Examples I can think of and add to your post are stress induced domain switch (like in BTO) and stress induced phase transofrmation (ZrO2). I think that our mechanics community can contribute a lot to this area.
Again, thanks.