You are here
Journal Club for July 2022: Liquid crystal elastomers: programming, multifunctionality, and opportunities
Xueju (Sophie) Wang, Department of Materials Science and Engineering; Polymer Program, Institute of Materials Science, University of Connecticut
The focus of this special issue is on liquid crystal elastomers (LCEs), which has a rich varieity of dynamic phenomena and is an emerging research area. There have been a Journal Club theme on iMechanica related to this topic (Journal club for January 2018, Recent advances in liquid crystal elastomers, Shengqiang Cai ) that focuses on the syntehsis and mechanical behaviors of LCEs. For this special issue, I will focus on the proramming and multifunctionality of LCEs and discuss related challenges and opportunities.
1. Introduction
Liquid crystal elastomers (LCEs) have received increasing interest due to their unique properties including soft elasticity and large, reversible shape changes in response to external stimuli (e.g., heat or light)[1-4]. LCEs are networks of cross-linked polymer chains containing stiff rodlike molecules, called mesogens (Figure 1). The mesogens are either incorporated into the polymer backbone forming main-chain LCEs, or linked as a side group, leading to side-chain LCEs. The coupling between the rubber elasticity of polymer networks and the orientational order of the mesogens, which is stronger in main-chain LCEs, gives rise to the thermomechanical behaviors including soft elasticity and large, reversible shape changes[5]. More specifically, to program a thermo-responsive LCE for actuation, the mesogens in polydomain LCEs, where mesogens are only locally oriented in each domain, are first aligned along a director to form a monodomain (i.e., nematic mesophase), followed by heating above a nematic-isotropic transition temperature (Tni) to disrupt the order of the mesogens into an isotropic state and drive the macroscopic shape change. LCEs recover their nematic state when it cools below Tni.

Figure 1. Schematic illustration of the microstructure and reversible state transition in main-chain LCEs.
2. Programming (alignment) of LCE Structures
2.1 Existing alignment techniques.
The macroscopic shape-morphing behavior of LCEs is tightly coupled with the alignment of LC molecules, which is usually characterized via wide-angle X-ray scattering (WAXS) [6] and Fourier transform infrared spectroscopy (FTIR) [7]. The widely used alignment techniques rely on the mechanical orientation (like uniaxial stretching and bending) of a lightly cross-linked polydomain network in a two-step process for relatively simple shape changes (Figure 2A) [8-11]. With the subsequently developed one-step polymerization process for synthesizing LCEs[12], in situ molecular alignment techniques, including surface patterning[13-16] (Figure 2B), photoalignment[17], and the use of external fields (magnetic [18] and electric fields [19]) (Figure 2C), are employed to achieve complex patterning of surfaces. However, the alignment of LC molecules based on such techniques is typically limited to thin-film samples (<100 µm) due to the challenge of maintaining alignment away from the surface. More recently, direct ink writing (DIW) 3D printing is introduced to fabricate 3D structures of LCEs, where mesogens are aligned along the direction of the printing path resulting from filament-extrusion-induced stress[20-27] (Figure 2D). Diverse 3D structures of LCEs, including those with spatially programmed nematic order[24] and functionally graded properties[23], are fabricated. The nature of the DIW 3D printing, however, makes it very challenging to fabricate hollow, open-mesh 3D structures of LCEs, such as helices and conical spirals, without the use of sacrificial supporting materials. Also limited by the printing resolution, DIW 3D printing usually yields large-scale structures, although the recently developed two-photon polymerization (direct laser writing) enables voxelated programming of LC molecular orientations for 3D microstructures of LC networks[26-27].
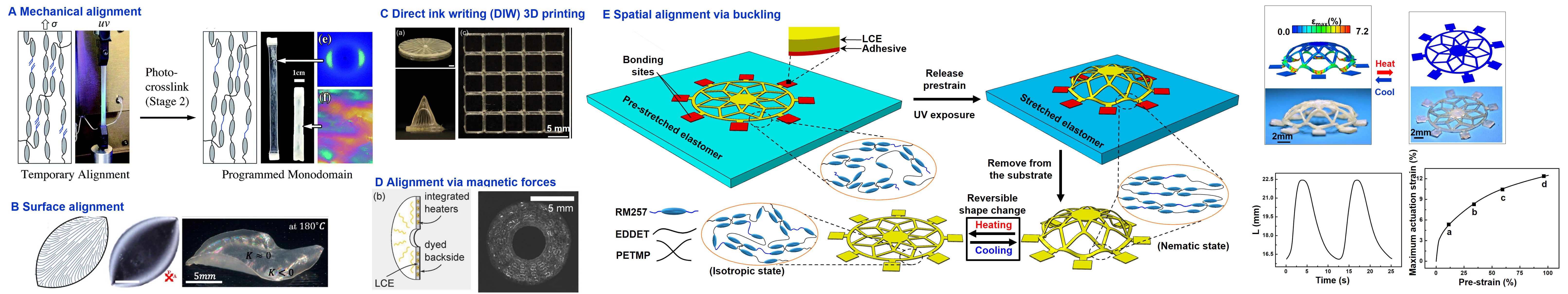
Figure 2. Programming of mesogen orders in LCEs. (A) Mechanical alignment. (B) Surface patterning. (C) 3D printing. (D) Alignment via magnetic forces. (E) Spatial alignment via buckling, including the schematic, experimental and FEA results, as well as characterizations.
2.2 Spatial programming via buckling.
We have recently reported a facile and versatile strategy to create previouly inaccessible reconfigurable 3D mesostructures of LCEs and ferromagnetic LCE composites via spatial programming of LC molecules into complex patterns [28] (Figure 2E). The approach exploits compressive forces induced via the release of a biaxially prestrained substrate[29] for deterministic geometric transformation of 2D LCE patterns into 3D structures, where mesogens are spatially aligned into their monodomains induced by compressive deformation. Figure 2E shows a schematic illustration of creating open-mesh 3D LCE structures through this approach as well as the reversible shape-switching behavior of the assembled 3D LCE mesostructures. The scheme begins with the fabrication of 2D patterns of LCEs synthesized following the two-stage thiol–acrylate Michael addition reaction (TAMAP) methodology[30] due to its high processibility and ease of use. LCE films of intermediate polydomain networks are formed after the first-stage reaction and patterned into desired geometries, which are then transferred onto a pre-stretched silicone elastomer substrate as an assembly platform. Application of an ultra-thin layer of superglue between the 2D pattern and the substrate at selective locations forms strong adhesion at those sites (bonding sites), while the interface at all other locations is governed by relatively weak Van der Waals forces. Releasing the pre-strain in the elastomer substrate induces large compressive forces at the bonding sites and therefore in- and out-of-plane translations, transforming the 2D LCE pattern into a 3D structure. At the microscale, under deformations induced by compressive buckling, mesogens undergo spatial mechanical alignment into local monodomains of ordered LC phases. The alignment of the mesogens and polymer chains tend to be along the direction of maximum principal strains[31]. Ultraviolet light (UV) exposure of the buckled 3D LCE structure initiates the second-stage polymerization process, where the ordered phases (nematic state) of the LCE are permanently programmed, thereby “locking” the buckled 3D shape. Such shape fixing effect allows immediate access to fully freestanding 3D LCE mesostructures. Heating the structure above the isotropic clearing temperature (62 ℃ in this study[31]) disrupts the mesogen molecular order and creates internal stresses driving the 3D LCE structure to return to its 2D configuration. The nematic-to-isotropic transition can be reversibly realized via heating and cooling across the transition temperature, thereby enabling the macroscopic reversible shape-switching capability of the 3D LCE mesostructure. Figure 3 shows experimental results and finite element analysis (FEA) predictions for a collection of reconfigurable 3D LCE structures (material thickness: 60 µm-400 µm) formed via spatial patterning of LC molecules during compressive buckling as well as their reversible shape changes, which demonstrates the reliability of the strategy.
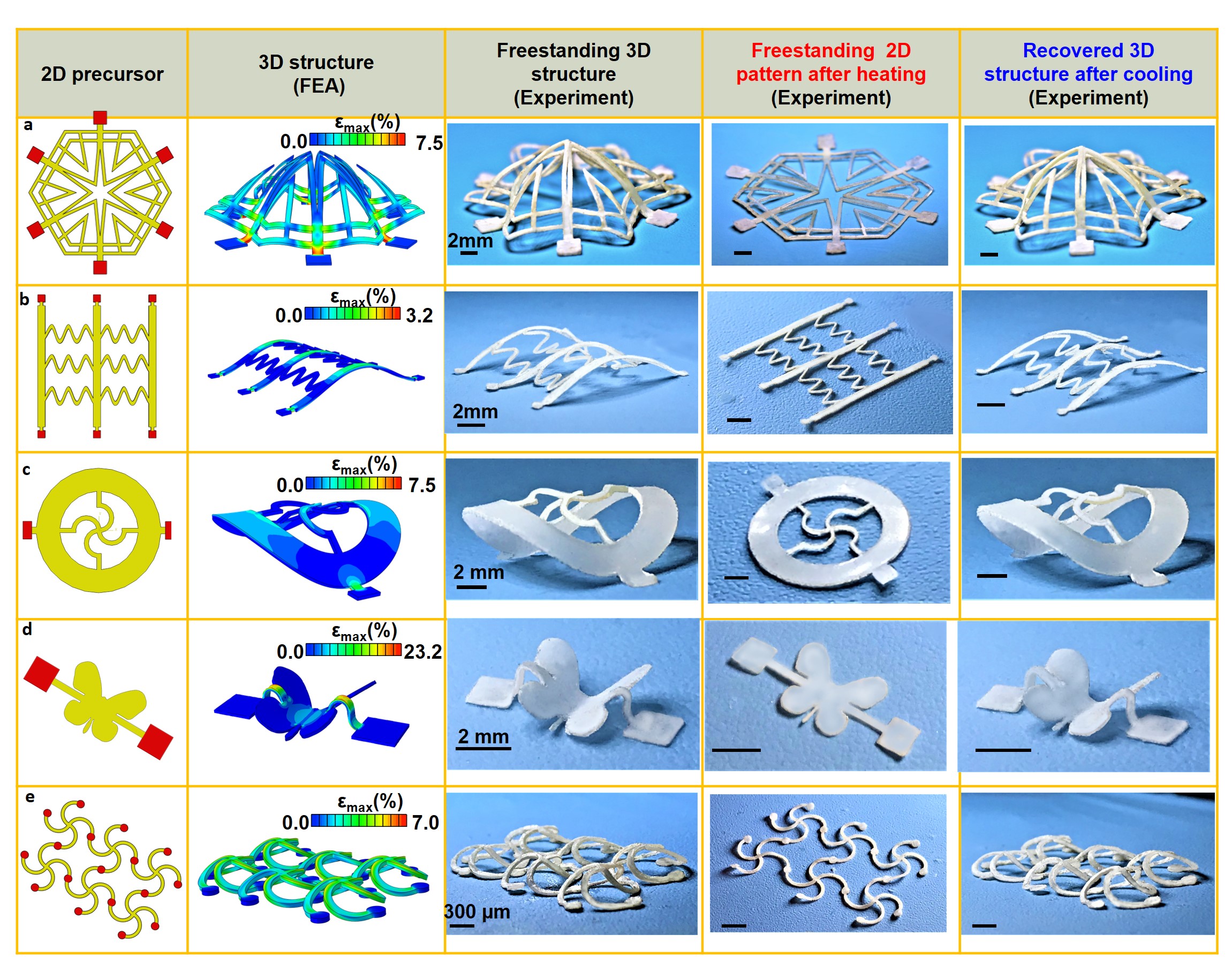
Figure. 3. Diverse reconfigurable, freestanding 3D LCE structures enabled by spatial alignment during compressive buckling.
2.3 Local programming
We further tailor the stiffness and the morphing behavior of reconfigurable LCE structures via locally controlled mesogen alignment and crosslinking densities at the molecular level [32]. Selective photopolymerization of spatially aligned LCE structures yields well-controlled lightly and highly crosslinked domains of distinct stiffness and selective permanent mesogen programming. More specificaly, applying a photomask during photopolymerization yields localized programming of LCE monodomains and highly crosslinked networks in the exposed regions. (Figure 4A). The Young's modulus of the light-exposed regions is found to be almost 50 times larger than that of the non-exposed ones, creating heterogeneous stiffness in a single 3D LCE structure (Figure 4B). Heating and cooling across Tni of LCEs induces the nematic–isotropic transition at the locally programmed regions, driving the shape morphing of the entire LCE structure into an interesting, asymmetric LCE structure.
Harnessing the correlations between mesogen alignment and crosslinking at the molecular level and material properties and morphing behaviors at the material/structure level create many opportunities for locally programmed LCE structures for potential applications in smart muscles, biomedical devices, and many others. This techquniqe based on mechanical training and locally controlled photopolymerization can enable the following salient features: 1) Formation of asymmetric, open-mesh 3D LCE structures with heterogeneous stiffness that are inaccessible with previous techniques, 2) sequential programming for repurposing or reshaping of structures by programming the lightly crosslinked regions, 3) complaint, stretchable, as well as large, reversible shape morphing due to the softer lightly crosslinked region, and 4) ease of use, versatile, scalable, and compatible with existing mesogen alignment techniques including folding and surface patterning.
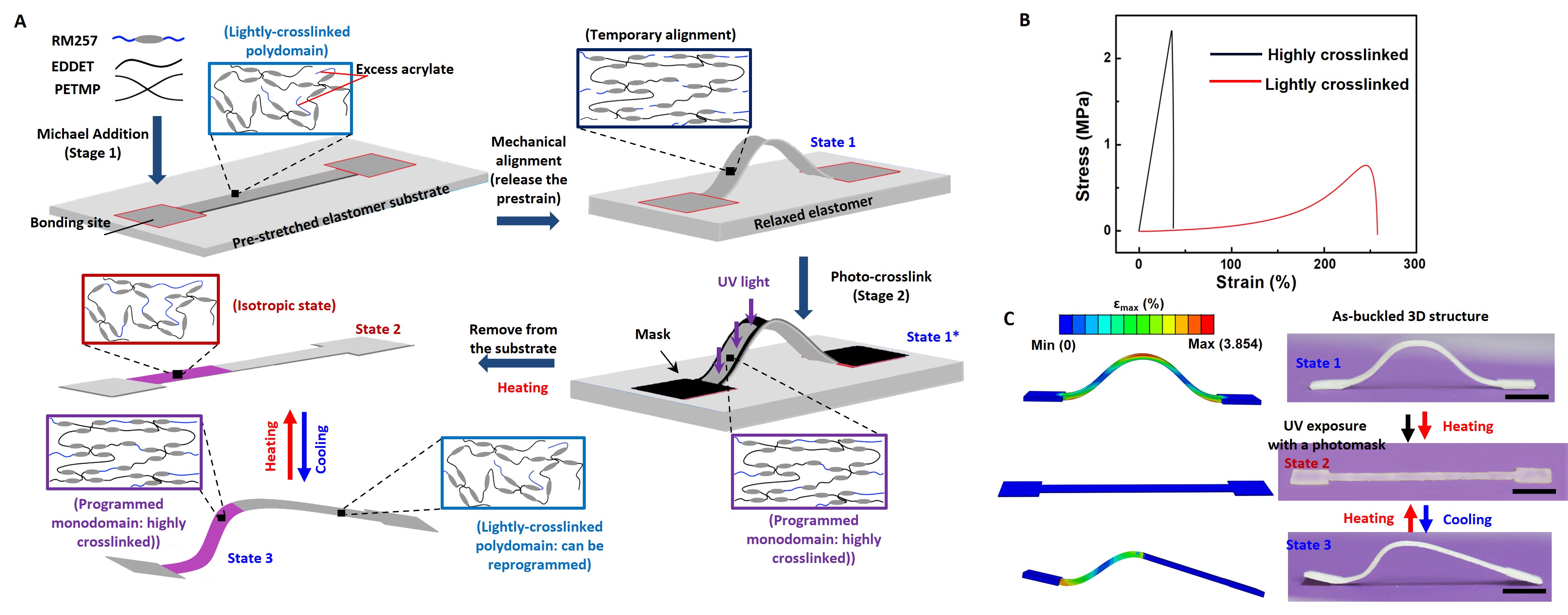
Figure 4. Stiffness-heterogeneous morphing LCE structures via local photopolymerization and mechanical training. (A) Schematic illustration of the fabrication and shape-morphing behavior of 3D LCE structures with heterogeneous stiffness, as well as the associated microscale mechanism. (B) Stress-strain curves of lightly and highly crosslinked LCEs. (C) FEA simulation and experimental results of the shape-morphing behavior of a 3D LCE ribbon structure after local photopolymerization and mechanical training. Scale bars, 2 mm.
3. Multifunctional LCEs for diverse applications
In addition to programming, we could enable multifunctional LCEs by incorporating other functional elements like magnetic particles, themochormic dyes, etc.
3.1 Integrating magnetic particles into LCEs for multi-stimuli responsiveness
By incorporating magnetic particles into LCEs, we have realized two functionalities: 1) enhance the mechanical properties of pure LCEs to enable the assembly of more compliant structures, and 2) enable dual magnetic and thermal actuation of the ferromagnetic LCE composite [28]. More specifically, we homogeneously embed hard neodymium-iron-boron (NdFeB) microparticles with an average diameter of 5 μm within LCEs during its synthesis. Figure 5A-B shows that the modulus of magnetic composites increases almost linearly with the magnetic particle concentration. In addition, adding magnetic particles to LCEs up to 50 wt% does not affect teh reversible shape changing behavior of LCEs (Figure 5C-D). Figure 5E presents experimental results and FEA predictions of three representative reconfigurable 3D structures assembled from 10 wt% ferromagnetic LCE composites (200 µm thick) via compressive and tensile buckling, including those in spiral configurations, which are too compliant to be buckled from pure LCE precursors. Such 3D magnetic LCE structures and their reversible shape-shifting behaviors demonstrate that the ferromagnetic LCE composite with enhanced modulus extends the structural design space while maintaining the large, reversible shape-morphing effect. The modulus of LCE can be further enhanced through the modulation of the mesophase structure[33].

Figure 5. 3D ferromagnetic LCE composite structures. (A) Engineering stress-strain curves of ferromagnetic LCE composite films with various mass percentage of ferromagnetic microparticales. (B) Plot of Young's modulus as a function of the percentage of ferromagnetic microparticales. (C) 3D shape storage ratio change curve: comparing 3D shape on the substrate with freestanding 3D shape. (D) 2D shape storage ratio change curve: comparing original 2D pattern with 2D state under heating. (E) Experimental and finite element simulation of the first reconfiguration cycle of 3D ferromagnetic LCE structures. Scale bars, 2 mm.
The integration of magnetic particles with LCEs also enables magnetic responsiveness of the mateiral. By assembling the ferromagnetic LCE composite films into a biomimetic 3D structure, we demonstrate a multistimuli-responsive robot that can achieve multiple motion modes, including jumping, rolling across an obstacle, passing through a narrow crack, and transferring a cargo, via integrated magnetic and thermal actuation. In particular, under integrated magnetic and thermal stimuli, it can pass through a narrow crack, which is half the height of the 3D robot (Figure 6). To get the robot through such a narrow crack, thermal stimuli applied using a heat gun first induces the nematic-to-isotropic transition of the LCE and drives the structure to morph into its 2D configuration, allowing it to enter and crawl underneath the narrow crack. Subsequently, magnetic actuation enables the 2D ferromagnetic LCE composite robot to propel through the crack quickly. Temporary removal of the thermal and magnetic stimuli leads to the transition of the LCE to its nematic state and, therefore, causes the robot to recover its 3D configuration. The ferromagnetic LCE composite robot enables responsiveness to both thermal and magnetic stimuli, allowing more flexibility for actuation. In addition, there are many opportunties to leverage existing efforts in magnetic actuation[34,35] and, more broadly, other functional materials and electronics with reconfigurable 3D LCE mesostructures to realize multifunctional systems.
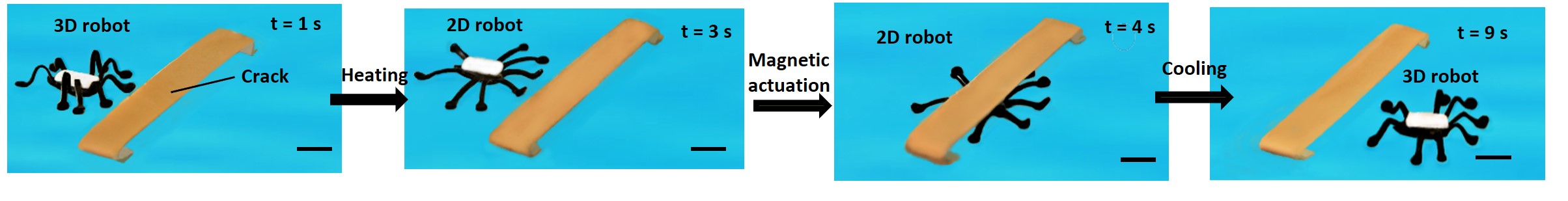
Figure 6. A magnetic LCE robot morphs between its 2D and 3D configurations to crawl underneath a narrow crack under thermal and magnetic stimuli. Scale bars, 5 mm.
3.2 Thermochromic LCEs for simultaneous color-changing and shape-morphing behaviours
Functional structures with reversible shape-morphing and color-changing capabilities are promising for many applications, including soft robotics, biomimetic camouflage devices, and many others. We realize such capabilities via spatially programmed liquid crystal elastomer (LCE) structures incorporated with thermochromic dyes [36]. By coupling the shape-changing behavior of LCEs resulting from the nematic-to-isotropic transition of mesogens with the color-changing thermochromic dyes, 3D thermochromic LCE structures change their shapes and colors simultaneously. Figure 7 shows a thermochromic LCE “octopus“ structure that can morph and change its color reversibly upon heating and cooling.

Figure 7. A thermochromic LCE “octopus“ structure that can morph and change its color reversibly under thermal stimuli. Scale bars, 3 mm.
3.3 LCEs as a platform for remote, reversible, and on-demand assembly
The reversible shape morphing properties of LCEs enable the use of LCEs as a powerful platform for remotely triggered, reversible assembly and reconfiguration of 3D mesostructures (Figure 8) [37]. As shown in Figure 8A, a uniaxially stretched LCE film enables the assembly of a 3D ribbon structure due to the elongation-contraction of LCEs upon thermal actuation. We perform polarized FTIR measurements of stretched LCE thin film samples (450 µm thick) as a means to explore the molecular alignment in the uniaxially stretched (0-12%) LCE assembly platform (Figure 8B-D). It is observed that the order parameter (S), a parameter to characterize the degree of mesogen alignment, increases with the strain level, with S values determined to be 0.195 and 0.377 for strains of 9% and 12%, respectively, suggesting a notable alignment of polymer chains within the LCE sample. This strategy allows the assembly of diverse structures of various material compositions (Figure 8E). In addition, a 3D buckled LCE structure can serve as a platform to biaxially assemble structures of other materials like ferromagnetic composites and shape memory polymers (Figure 8F).
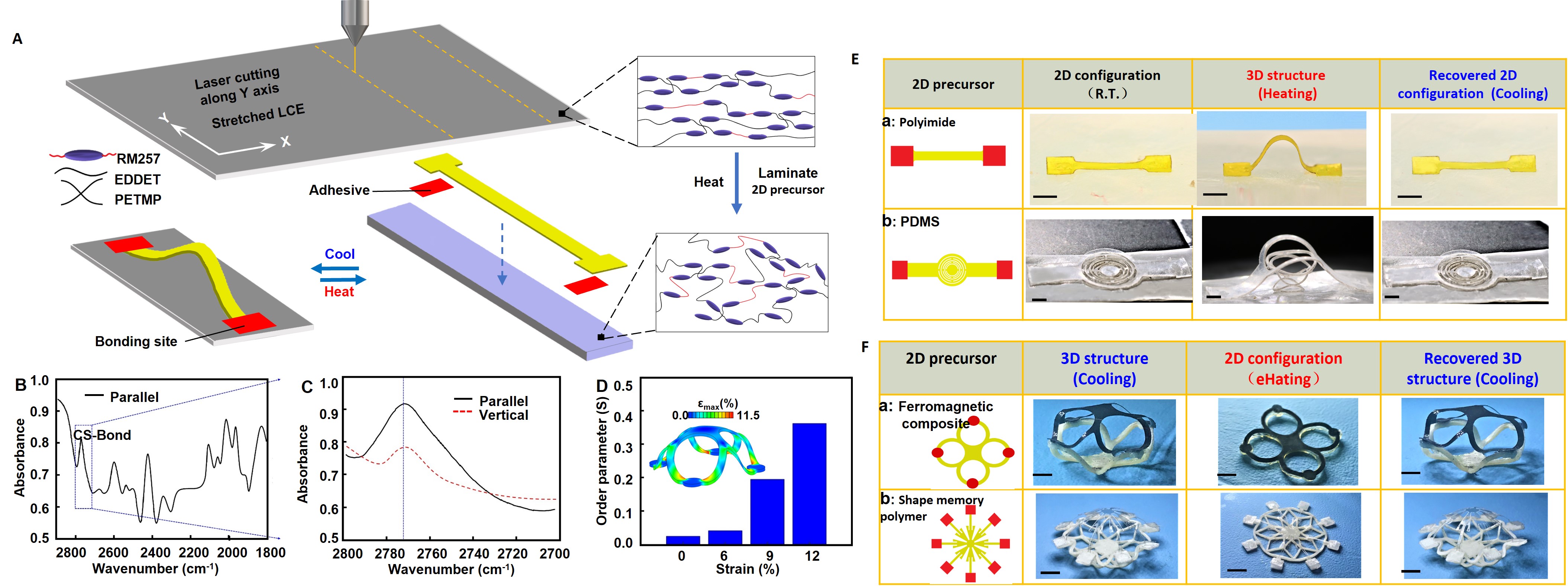
Figure 8. LCE as a substrate for remotely-controlled, reversible, on-demand assembly. (A) Schematic illustration of the assembly and reversible shape reconfiguration process for a 3D structure driven by the elongation-contraction switching of the permanently-stretched LCE substrate. (B) Polarized FTIR analysis of LCE sample stretched by 12% engineering strain. (C) Full spectrum IR absorbance at 0° polarization for creeped sample with monitored peak (~2770 cm-1) indicated. (D) Order parameters of LCE at different amplitude of tensile strain. (E) 3D structures assembled via an LCE thin film substrate over one reconfiguration cycle. Scale bars, 2 mm. (F) 3D structures assembled via an LCE 3D structure over one reconfiguration cycle. Scale bars, 2 mm.
The reversible, on-demand assembly capability is desired for a lot of applications such as in soft robotics and tunable electronic devices. As an example, we assemble a reconfigurable light-emitting system driven by the elongation–contraction behavior of the LCE substrate under heating and cooling[36]. Fiugre 9 schematically illustrates the design principle, where a 2D precursor of copper in spiral geometries is connected to a light-emitting diode (LED) and laminated onto a prestreched LCE substrate. Heating above 62 °C causes the LCE substrate to contract in the X-direction and to elongate in the Y-direction, buckling the 2D copper precursor into a 3D structure. Upon 3D assembly, the copper ribbons are brought into contact and thereby form a closed circuit to turn on the LED. Cooling the LCE substrate to room temperature leads to the contraction of the LCE substrate in the Y-direction and recovers the 3D structure to its 2D state, where the copper ribbons are separated, turning the LED off. Figure 9B shows experimental results of the reconfigurable light-emitting system upon heating and cooling the LCE substrate. Such a remotely tunable electronic system demonstrates the capability of the LCE platform for the on-demand, reversible shape morphing of 3D functional devices.
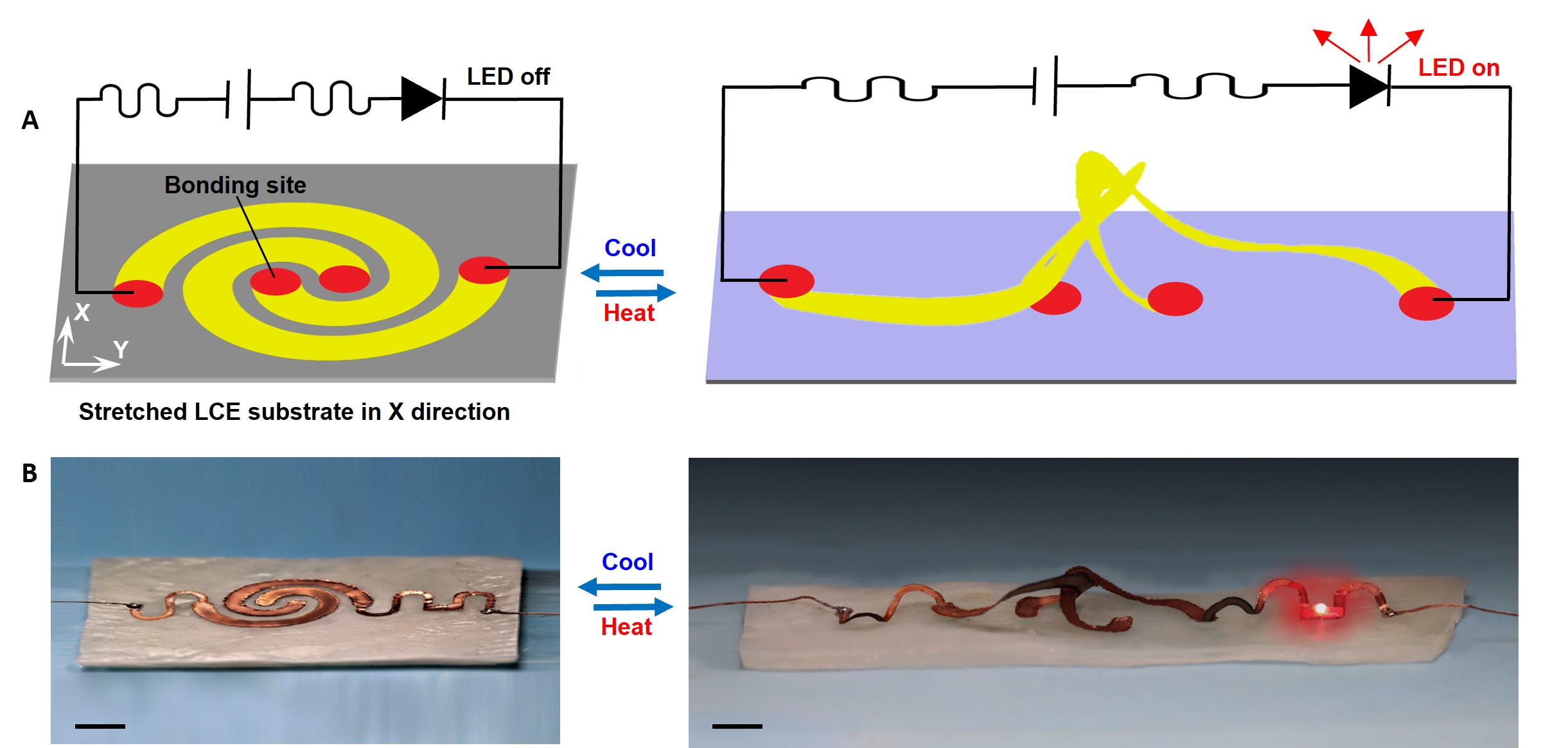
Figure 9. Reconfigurable light-emitting system. (A) Schematic illustration of the assembly and reconfiguration of a light-emitting system via the LCE platform. (B) Experimental results of the 2D (LED off) and 3D configurations (LED on) of the light-emitting system under cooling and heating of the LCE substrate. Scale bars, 3 mm.
Summary and outlook
To sum up, the large, reversible shape changes of LCEs, which result from the coupling between the alignment of liquid crystal molecules and the macroscopic deformation of polymer networks, have attracted much attention for applications including soft robotics and biomedical devices. In this club, we introduced the programming, multifunctionality, and potential applications of LCE structures.
Some open problems that can be addressed include:
· Characterization of spatial alignment in 3D LCE structures in situ and ex situ. The alignment of mesogens and polymer chains in LCEs is usually characterized via wide-angle X-ray scattering (WAXS)[38] and Fourier-transform infrared spectroscopy (FTIR)[6]. Both techniques, however, require 2D thin film samples for testing, which prevents their application to characterizing the molecular alignment in 3D LCE structures. Developing techniques to characterize mesogen alignment in 3D LCE structures both in situ and ex situ would allow the quantification of complicated mesogen orientations and provide important input for the design of LCE structures.
· Integration between experimental and modeling studies. Quantitative comparisons between experiments and theoretical predictions are generally lacking to validate the model for LCEs, improve its predictive capability, and guide the design of LCE structures. Therefore, it would be interesting to integrate modeling and experimental efforts to study the behaviors of LCEs, including 1) complicated structures with spatial alignment of mesogens, 2) the nematic-isotropic-transition induced shape morphing under external stimuli, beyond the current study of mechanical behaviors in the nematic state of LCEs under external loading conditions, and many other intriguing phenomena.
· Real applications of LCEs. Interesting demonstrations of using LCEs for applications like soft robotics have been shown. It would further advance the field of LCEs to find more practical applications and/or system-level demonstrations.
Here, we invite all researchers active in this field or have a general interest in liquid crystal elastomers, smart materials, and soft robots to share their perspectives. Introducing their recent progress related to this subject is also highly welcome. We look forward to a fruitful discussion.
References
1 Traugutt, N. A. et al. Liquid-Crystal-Elastomer-Based Dissipative Structures by Digital Light Processing 3D Printing. Advanced materials (Deerfield Beach, Fla.) 32, e2000797-e2000797, doi:10.1002/adma.202000797 (2020).
2 Davidson, E. C., Kotikian, A., Li, S., Aizenberg, J. & Lewis, J. A. 3D Printable and Reconfigurable Liquid Crystal Elastomers with Light-Induced Shape Memory via Dynamic Bond Exchange. Advanced materials (Deerfield Beach, Fla.) 32, e1905682-e1905682, doi:10.1002/adma.201905682 (2020).
3 He, Q. et al. Electrically controlled liquid crystal elastomer-based soft tubular actuator with multimodal actuation. Science advances 5, eaax5746-eaax5746, doi:10.1126/sciadv.aax5746 (2019).
4 Guin, T. et al. nLayered liquid crystal elastomer actuators. Nature Communications 9, 2531-Article No.: 2531, doi:10.1038/s41467-018-04911-4 (2018).
5 Ohm, C., Brehmer, M. & Zentel, R. Liquid crystalline elastomers as actuators and sensors. Advanced Materials 22, 3366-3387 (2010).
6 Harada, M. et al. In-situ analysis of the structural formation process of liquid–crystalline epoxy thermosets by simultaneous SAXS/WAXS measurements using synchrotron radiation. Polymer journal 45, 43-49 (2013).
7 Tammer, M., Li, J., Komp, A., Finkelmann, H. & Kremer, F. FTIR‐Spectroscopy on Segmental Reorientation of a Nematic Elastomer under External Mechanical Fields. Macromolecular Chemistry and Physics 206, 709-714 (2005).
8 Brömmel, F., Kramer, D. & Finkelmann, H. in Liquid Crystal Elastomers: Materials and Applications 1-48 (Springer, 2012).
9 Diaz-Calleja, R. & Riande, E. Biaxially stretched nematic liquid crystalline elastomers. The European physical journal. E, Soft matter 35, 2-2, doi:10.1140/epje/i2012-12002-5 (2012).
10 Küpfer, J. & Finkelmann, H. Nematic liquid single crystal elastomers. Die Makromolekulare Chemie, Rapid Communications 12, 717-726 (1991).
11 Finkelmann, H., Kock, H. J. & Rehage, G. Investigations on liquid crystalline polysiloxanes 3. Liquid crystalline elastomers—a new type of liquid crystalline material. Die Makromolekulare Chemie, Rapid Communications 2, 317-322 (1981).
12 Donnio, B., Wermter, H. & Finkelmann, H. A simple and versatile synthetic route for the preparation of main-chain, liquid-crystalline elastomers. Macromolecules 33, 7724-7729 (2000).
13 Barnes, M. & Verduzco, R. Direct shape programming of liquid crystal elastomers. Soft Matter 15, 870-879, doi:10.1039/C8SM02174K (2019).
14 Agrawal, A. et al. Surface wrinkling in liquid crystal elastomers. Soft Matter 8, 7138-7142 (2012).
15 Aharoni, H., Xia, Y., Zhang, X., Kamien, R. D. & Yang, S. Universal inverse design of surfaces with thin nematic elastomer sheets. Proceedings of the National Academy of Sciences 115, 7206-7211 (2018).
16 Babakhanova, G. et al. Liquid crystal elastomer coatings with programmed response of surface profile. Nature communications 9, 1-9 (2018).
17 Yaroshchuk, O. & Reznikov, Y. Photoalignment of liquid crystals: basics and current trends. Journal of Materials Chemistry 22, 286-300 (2012).
18 Buguin, A., Li, M.-H., Silberzan, P., Ladoux, B. & Keller, P. Micro-actuators: When artificial muscles made of nematic liquid crystal elastomers meet soft lithography. Journal of the American Chemical Society 128, 1088-1089 (2006).
19 De Jeu, W. H. Liquid crystal elastomers: materials and applications. Vol. 250 (Springer, 2012).
20 Ambulo, C. P. et al. Four-dimensional printing of liquid crystal elastomers. ACS applied materials & interfaces 9, 37332-37339 (2017).
21 Davidson, E. C., Kotikian, A., Li, S., Aizenberg, J. & Lewis, J. A. 3D Printable and Reconfigurable Liquid Crystal Elastomers with Light‐Induced Shape Memory via Dynamic Bond Exchange. Advanced Materials 32, 1905682 (2020).
22 Barnes, M. et al. Reactive 3D Printing of Shape-Programmable Liquid Crystal Elastomer Actuators. ACS Applied Materials & Interfaces 12, 28692-28699, doi:10.1021/acsami.0c07331 (2020).
23 Wang, Z. et al. Three-dimensional printing of functionally graded liquid crystal elastomer. Science Advances 6, eabc0034, doi:10.1126/sciadv.abc0034 (2020).
24 Kotikian, A., Truby, R. L., Boley, J. W., White, T. J. & Lewis, J. A. 3D Printing of Liquid Crystal Elastomeric Actuators with Spatially Programed Nematic Order. Advanced materials (Deerfield Beach, Fla.) 30, doi:10.1002/adma.201706164 (2018).
25 Roach, D. J. et al. Novel ink for ambient condition printing of liquid crystal elastomers for 4D printing. 27, 125011 (2018).
26 Zeng, H. et al. Alignment engineering in liquid crystalline elastomers: Free-form microstructures with multiple functionalities. Applied Physics Letters 106, 111902 (2015).
27 Guo, Y., Shahsavan, H. & Sitti, M. 3D Microstructures of Liquid Crystal Networks with Programmed Voxelated Director Fields. Advanced Materials 32, 2002753 (2020).
28 Li, Y., Yu, H., Yu, K., Guo, X. & Wang, X. Reconfigurable Three-Dimensional Mesotructures of Spatially Programmed Liquid Crystal Elastomers and Their Ferromagnetic Composites. Advanced Functional Materials 31, 2100338, doi:https://doi.org/10.1002/adfm.202100338 (2021).
29 Xu, S. et al. Assembly of micro/nanomaterials into complex, three-dimensional architectures by compressive buckling. 347, 154-159 (2015).
30 Yakacki, C. et al. Tailorable and programmable liquid-crystalline elastomers using a two-stage thiol–acrylate reaction. Rsc Advances 5, 18997-19001 (2015).
31 Saed, M. O., Torbati, A. H., Nair, D. P. & Yakacki, C. M. Synthesis of Programmable Main-chain Liquid-crystalline Elastomers Using a Two-stage Thiol-acrylate Reaction. Jove-Journal of Visualized Experiments, e53546-Article No.: e53546, doi:10.3791/53546 (2016).
32 Li, Y., Parlato, G., Masese, F., Kasi, R., Zhang, T., Wang, X. Morphing of Stiffness-Heterogeneous Liquid Crystal Elastomer Structures Via Mechanical Training and Locally Controlled Photopolymerization. Under reivew (2022).
33 Saed, M. O. et al. High strain actuation liquid crystal elastomers via modulation of mesophase structure. Soft Matter 13, 7537-7547 (2017).
34 Kim, Y., Yuk, H., Zhao, R. K., Chester, S. A. & Zhao, X. H. Printing ferromagnetic domains for untethered fast-transforming soft materials. Nature 558, 274-+, doi:10.1038/s41586-018-0185-0 (2018).
35 Hu, W., Lum, G. Z., Mastrangeli, M. & Sitti, M. Small-scale soft-bodied robot with multimodal locomotion. Nature 554, 81-85 (2018).
36 Li, Y., Teixeira, Y., Parlato, G., Wang, X. Three-Dimensional Thermochromic LCE Structures with Synchronous, Reversible Shape-Morphing and Color-Changing Capabilities for Soft Robotics. Under reivew (2022).
37 Li, Y., Luo, C., Yu, K., Wang, X. J. A. A. M. & Interfaces. Remotely Controlled, Reversible, On-Demand Assembly and Reconfiguration of 3D Mesostructures via Liquid Crystal Elastomer Platforms. 13, 8929-8939 (2021).
38 Tammer, M., Li, J., Komp, A., Finkelmann, H. & Kremer, F. FTIR-Spectroscopy on Segmental Reorientation of a Nematic Elastomer under External Mechanical Fields. Macromolecular Chemistry and Physics 206, 709-714, doi:https://doi.org/10.1002/macp.200500050 (2005).
- Xueju Sophie Wang's blog
- Log in or register to post comments
- 34643 reads


Comments
Does LCE form SIC?
Hi Xueju,
This is a fascinating summary. Thanks for sharing this timing review.
Some elastomers especially natural rubber show pronounced strain-induced crystallization (SIC). Does liquid crystal elastomer also experience strain-induced crystallization? If this is the case, how the strain-induced crystallization is coupled with the state transition in LCE?
Best,
Shaoting
Does LCE form SIC?
Hi Shaoting,
Thank you for your kind note and very good question! Actually, we did observe strain-induced alignment of polymer chains in LCEs. For example, in our work (ACS Applied Materials & Interfaces 13.7 (2021): 8929-8939 https://pubs.acs.org/doi/abs/10.1021/acsami.0c21371), which is also shown in Figure 8B-8D of this journal club, we characterized the alignment degree of polymer chains in uniaxially stretched LCEs as a function of strain using Polarized FTIR. We showed that the alignment degree (order parameter) of polymer chains increases with the strain.
Regarding your second question, the alignment of the polymer chains and the alignment of mesogen units in LCEs are inherently linked, especially for main-chain LCEs where mesogens are incorporated into the polymer backbone. Therefore, people usually use the polymer chain alignment to estimate the mesogen alignment degree including in our work. Given this strong coupling/linking between the polymer chain and mesogen alignment, during the nematic-to-isotropic state transition in LCEs, the aligned polymer networks are also disrupted into an isotropic state along with mesogens. Recently, Dr. Kai Yu also performed real-time characterizations of alignment and reorientation of polymer chains in LCEs using in situ optical measurements https://pubs.acs.org/doi/full/10.1021/acsami.1c20082.
Hopefully this is helpful.
Best
Xueju
Does LCE form SIC?
Hi Shaoting,
I probably should have clarified that the strain-induced alignment of polymer chains is observered but may be different from the SIC you mentioned. Please refer to the followed dicussion on semi-crystaline LC networks by integrating polymer cyrstalinity and liquid crystalinity within LCEs.
Semicrystalline LC networks
Hi Shaoting,
I am not aware of SIC in rubbery LCEs. But semicrystalline LC polymer networks can be made, where strain-induced (re)crystallization is expected. This is a new kind of material developed by a few groups such as Ware (https://pubs.acs.org/doi/10.1021/acs.macromol.7b00567) and Hayward (https://pubs.acs.org/doi/10.1021/acsmacrolett.0c00328). It is a very interesting material system with a lot of unknowns to explore. We had a recent paper using phase field modeling to study its phase transition under various light and temperature (https://journals.aps.org/pre/abstract/10.1103/PhysRevE.103.033003).
Semicrystalline LC networks
Hi Ruobing,
Thank you for sharing the great works of combining polymer crystallinity with liquid crystalinity in LCEs to offer both load-bearing and shape-morphing capabilities. It is indeed a very interesting material system that offers many new opportunities. The phase field model also looks quite interesting. It also reminds me a work along this line by Yakacki https://pubs.rsc.org/en/content/articlelanding/2017/sm/c7sm01380a, which discusses integrating semi-crystalinity with LCEs as well as tuning the rate of polymer crystalization (from 5 min to 2-3 hours) by varying the spacer length while maintaining the same mesogen (RM257) during the synthesis of LCEs.
Controlled local programming
Hi Xueju,
Thank you for putting together this nice and timely topic of LCE for the mechanics community. I am particularly interested in your methods of controlled local programming in 2.3. In addition to the crosslink density, it is interesting and also very challenging to achieve spatially patterned mesogen direction programming. Some existing methods include printing and surface alignment, which are relatively difficult to implement without certain expertise. Are there any other potential methods to achieve complex direction programming? In particular, I am wondering if designing the mechanics in the material/structure can play an important role in such programming.
Best regards,
Ruobing
Hi Ruobing,
Hi Ruobing,
In addtion to the 3D printing and surface alignment, people also used the imprint lithography to make the LCE 3D structure. In the printing and surface alignment techniques, the 2D film is in liquid crystal state and after heating, the 2D film transit to 3D structure. While with the imprint method, the LCE would change from the 3D shape to a 2D film after heating. The mesogen direction and alignment is determined by the localized stretching in the imprint process. In Xueju's work, the shape in the isotropic state is also a flat film. Very interesting design.
Best,
Zhijian
Hi Ruobing,
Hi Ruobing,
Thanks for your kind note and good question! Well-controlled spatial programming is important to create complicated shape-morphing LCE structures, but is usually difficult to achieve in a facile manner. We have tried a few different techniques, inlcuding buckling (compressive and tensile buckling), folding, and also imprinting that Zhijian mentioned. All of them actually worked pretty well regarding creating spatially aligned LCE structures with reversible shape-morphing capabilities. Designing the mechanics in the material/structure definitely plays an important role in such programming. One thing that would help with such design is the quantitative characterization of the spatial alignment as a function of strain/stress in the material/structure to serve as an input. But this type of characterization is also a challenge as I mentioned in this journal club, because most current techniques including WAXS and FTIR require 2D thin film samples for testing.
Best,
Xueju
Thank you. That is very interesting
Hi Zhijian and Xueju,
Thank you for the sharing the methods especially the imprint one. That is very interesting! I think there is a lot to explore in mechanics in this aspect of method.
Regards,
Ruobing
The stiffness in the spatial programming via buckling
Hi Xueju,
Thanks for the timely review. I am interested in the spatial programming via buckling. The lightly crosslinked LCE is usually very soft. Is it needed to tailor the crosslinking density and thickness of the lightly crosslinked LCE to make the buckling structure?
Best,
Zhijian
The stiffness in the spatial programming via buckling
Hi Zhijian,
Good question! The strain energy (W) required to buckle the structure is related to the elastic modulus (E), thickness (t), and lateral dimension (w) of the structure like ribbons via a simple scaling law: W ∝ Ewt^3. So we can tailor the thickness of the soft lightly crosslinked LCE to enable successful buckling. For structures shown in our work (https://onlinelibrary.wiley.com/doi/full/10.1002/adfm.202100338), we typically use 200-600 micronmeter thick LCE films. In addition, we can also enhance the modulus of pure LCEs by incorporating hard elements like magnetic particles (Figure 5A-5B in this journal club) or by modulating the space length during the synthesis of LCEs (introducing polymer crystalinity). We have not tried tailoring the crosslinking density in the lightly crosslinked stage of LCEs before buckling to increase its modulus, but we believe that will also help!
Thanks for the timely review
Hi Xuejue,
Thanks for the informative review. Using the poping structure to program LCE is very creative. Congratulations!
I have one general question regarding, which I also often ask myself and the students. What are the advantage/uniqueness of LCE as compared to shape memory polymers (in particular, two way shape memory polymer), for various practical applications?
shengqiang
LCEs and SMPs
Hi Shengqiang,
Thanks for your kind note. This is a very good question! Yes. Shape memory polymers (SMPs) can be classified into one-way, two-way, and multiple (multiple temporary shapes) SMPs. One major category of two-way SMPs is based on semicrystalline polymers that can exhibit a reversible shape memory property under constant stress, such as crystallization-induced elogntation during cooling and melting-induced contraction upon heating. Actually people also consider LCEs as another major category of two-way SMPs, like in this review paper on SMPs (https://onlinelibrary.wiley.com/doi/full/10.1002/adma.202000713#adma202000713-bib-0056). Compared to semicrystalline-polymer-based two-way SMPs, I think the major advantages of LCEs are still the soft elasticity, large, reversible shape changes, and softness due to the combination of polymer networks and liquid crystals (mesogens). The modulus of typical SMPs is usually on the order of GPa, while LCEs can be much softer (modulus less than 1 MPa) and therefore would be better for applications where very soft materials are needed like in soft robotics and tissue scaffolds. In addition, we can also incoporate polymer crystalinity into LCEs for load-bearing, shape-morphing capabilities like in the works of Taylor (https://pubs.acs.org/doi/10.1021/acs.macromol.7b00567) that Ruobing mentioned and Yakacki (https://pubs.rsc.org/en/content/articlelanding/2017/sm/c7sm01380a). Compared to one-way and multiple (temporary shapes) SMPs, I think the major advantage of LCEs is the reversible shape changes. To me, LCEs offer more functionality and flexibility compared to (other) SMPs. Please feel free to share your thoughts!
Best,
Xueju
Thanks, Xueju. Your
Thanks, Xueju. Your elaboration makes lots of sense.
mesogens aligned by buckling
Xueju, wonderful article! I have a question about mesogens aligned by buckling. In a two-step crosslinking, people typically apply relatively high strain to align mesogens. However, in your designs, you use compressive strain due to buckling to align mesogens. I expect the compressive strain is pretty low. What's the typical order parameter and spontaneous strain that you can achieve?
Mesogens aligned by buckling
Hi Lihua,
Great question! Yes. The maximum principal strain within the buckled 3D LCE structures we have so far varies from 2.2% to 23%. Regarding the order parameter, we were not able to directly measure it because the strain distributions and mesogen orientations in the 3D LCE structures are very complicated and current techniques for measuring mesogen alignment (WAXS and FTIR) require 2D thin film samples. To characterize the alignment within buckled 3D LCE structures, we performed FEA modeling on maximum principal strain distributions within the 3D structure and then conducted polarized FTIR measurements of stretched LCE thin film samples with strain levels corresponding to those from FEA modeling, since the mesogens and polymer chains tend to align in the direction of maximum principal strains. Here is the order parameter as a function of strains levels corresponding to those in a 3D LCE structure (also shown in Figure 8D in the post). It is observed that the order parameter increases with the strain level, achieving a magnitude of 0.37 at a strain of 12%, which shows a notable alignment of polymer chains within the LCE sample.
More information could be found from the following two papers.
https://onlinelibrary.wiley.com/doi/10.1002/adfm.202100338
https://pubs.acs.org/doi/10.1021/acsami.0c21371
Interstingly, we found that all the 3D buckled LCE structures have very good reversible shape-morphing capabilities even for those with very small strain levels (a few percent). There are still a lot of interesting questions we would like to explore here. For example, in our works, we only used a simple neo-Hookean model to simulate the buckling process. A model that incorporates the complicated constitutive laws of LCEs to simulate the buckling of LCE structures would be expected to improve the accuracy of the results. Also, in situ/ex situ measurement of the complicated spatial mesogen alignment in 3D LCE structures would provide important input for the modeling and design of shape-morphing 3D LCE structures. Collaborations are always welcome.
Best,
Xueju
LCE ABAQUS UMAT
Xueju, thank you for your response. This is interesting. Recently, we published the following papers on LCE shape morphing using a combined method of experiments, analytical differential geometry modeling, and finite element analysis. We have written an ABAQUS UMAT for LCEs based on the neo-classical model. It can be openly accessed as a supplementary material in the second paper, if you are interested in using it. Please feel free to let us know if you have any questions about it.
https://onlinelibrary.wiley.com/doi/abs/10.1002/adma.202000609
https://pubs.rsc.org/en/content/articlelanding/2022/sm/d1sm01830b/unauth
LCE ABAQUS UMAT
Lihua,
Thanks a lot for sharing your great works and openly accessibly code! I read both papers before but did not read the modeling part in details. I will take a closer look and will let you know if I have any questions.
Xueju
Mesogens aligned by buckling
Hi Xueju,
Congratulations! Wonderful and inspring summary! I have two questions:
1. Similar to Lihua, it is interesting to see the mesogen alignment under a relatively small stretching strain of 9%-12% through 3D buckling, as opposed to the conventional mechanical stretching over 120% to align them. Any idea on how to understand the small-strain induced alignment phenomenon in the buckled structure?
2. I see you are using laser cutting to cut the first-step cured LCE samples to certain patterns. The localized heating during the cutting could dramatically change the properties of the cutting area, will this affect the final 3D buckled strucuture? Thanks.
LCE in nature
Hi Xueju,
Thanks a lot for this excellent review! You give a very nice example of how mechanics can be tightly integrated with material and structure advancement to acheive new functions.
I have a very general question about LCE, which responds to heat and light. Are there LCE like materials/structures in nature that work in a similar way? We often seek inspiration from nature, such as hydrogels and composites. I do not find too much discussion about the nature counterpart of LCE.
Also, light and heat are generally coupled if the stimuli are lights. Is it possible to quntify the effects of light and heat indivually? Or they will likely be coupled in a nonlinear way.
Thanks.
Best,
Teng
Hi Teng
Hi Teng,
For LCE-like materials in nature: many biological molecules essentially form liquid crystalline phase, such as those in a biofilm. I could imagine as they are "crosslinked" in their nature form, they might be treated as liquid crystal networks. However, I am not in the field and am not sure if their mechanical behaviors show analogy to synthetic LC elastomers.
Light actuation of a LCE can be based on photothermal or photochemical. For photochemical mechanism, there are rich examples in nature that convert light to other forms of energy or signal using the same mechanism. Examples include the cis-trans photoisomerization for vision, photochemistry-induced DNA damage and repair, and photosynthesis.
The coupling between light and heat during an actuation is intriguing. If it is photothermal actuation, then the coupling is basically from photo-induced heating and the subsequent thermomechanics of LCE. If it is photochemistry, the light-temperature coupling might be more complex. They indeed couple in a nonlinear way. In both cases, kinetics may play important roles. I attach a recent theoretical work by my student on the light-temperature coupling in LCEs: https://www.sciencedirect.com/science/article/abs/pii/S2352431622000062
Also, in certain scenario, light and heat can be decoupled by properly designing the experiment. This was an important problem in light actuation of LCEs when it initially emerged: people were wondering whether the actuation is from photochemistry, or merely photochemistry-induced heating. A pioneering work by Yu and Ikeda et al answered this question by looking into light with various polarization and LCEs with polydomain (https://www.nature.com/articles/425145a).
Best,
Ruobing
Thanks Ruobing for the
Thanks Ruobing for the detailed explanations and sharing the nice work! The two papers are indeed very examples of coupled and decoupled light and heat effects.
Looking forward to more exciting work from you.
Best,
Teng
Ruobing, thanks for sharing
Ruobing, thanks for sharing your thoughts and the works!
Teng, all great questions!
Teng, all great questions! Regarding the biomimetics/inspiration from nature, please also check Dr. Chris Yakacki's comment on mimicking soft tissues with LCEs. LCEs have been explored a lot for applications in artificial muscles. One recent work by Shengqiang on LCE microfiber actuators can be found from the following link.
https://www.science.org/doi/10.1126/scirobotics.abi9704
hysteresis
Hi Sophie, this is an excellent overview of the subject which I really enjoyed reading. I have an intrest in the magnetically responsive system you created. Do you happen to know if any hysteretic effect sets in after repeated actuations of the system? The hysteresis need to be just magnetic but perhaps an overall one pertaining to the interaction of the mesogen and magnetic patterns.
Hysteresis
Hi Pradeep, great question! So far our study on magnetic LCEs is relatively qualative, focusing on the dual actuation, so I do not know if there is any hysteretic effect in the material after repeated actuations either purely from the magnetic part or from the interaction (e.g., magnetic forces can affect the alignment of mesogens) in the magnetic LCE composite. But it is a very interesting question to explore. I will keep you posted if we find something out.
Xueju (Sophie)
Hi Sophie,
Hi Sophie,
Thank you for summarizing all these fantastic research progress about LCE! Most of them have 2D geometries or small thicknesses in applications. I am wondering whether bulk LCE materials are also applied to 3D (volumetric) applications or can be programmed in 3D?
Best regards,
Jin
3D bulky LCEs
Hi Jin,
Good question! LCEs have many exceptional properties, including large, reversible shape changes that have great potential as actuators, high energy dissipation, soft elasticity, etc. When LCEs are used for actuation purposes, thin-film geometries are usually desired because of the ease of programming with existing techniques (mechanical alignment, surface patterning, etc.) and easy actuation. For example, LCEs are typically light- or heat-responsive, but neither light nor heat works well for very thick (bulky) LCEs due to the relatively shallow light penetration depth or the thermal gradient in thick LCEs during actuation. When LCEs are utilized for other properties like energy dissipation, usually bulky LCE structures are fabricated using techniques like molding or digital light processing (DLP) 3D printing, where mesogens do not need to be programmed because no actuation is needed (https://onlinelibrary.wiley.com/doi/full/10.1002/adma.202000797).
Regarding programming 3D (bulky/volumetric) LCEs, it is possible through techniques like the direct ink writing (DIW) 3D printing, where mesogens are aligned along the printing path during the fabrication of the 3D LCE structure. For 3D bulky LCEs prepared via other techniques like molding, mechanical programming may work but the alignment may not be uniform.
Xueju (Sophie)
Thank you for this sharing.
Thank you for this sharing. -Jin
Great Work!
Great work and summary, Sophie! To add to the conversation about practical applications and nature, LCEs mimic human tissues quite remarkably. Beyond the reversible actuation like a muscle, LCEs mimic soft tissues as they have low modulus (~1 MPa), high dissipation, hierarchical order, and anisotropy.
Hi Chris,
Hi Chris,
Thanks a lot for the great comments on the practical applications of LCEs and the biomimetics from nature! It is very helpful to share with the community, which I believe benefits a lot from many of your pioneering work in this field.
Xueju (Sophie)